A uniform thin ring of mass 0.4kg rolls without slipping on a horizontal surface with a linear velocity of 10 cm/s. The kinetic energy of the ring is
joule
joule
joule
joule
The correct Answer is:1
To find the kinetic energy of a uniform thin ring rolling without slipping, we need to consider both translational and rotational kinetic energy. Here’s a step-by-step solution:
Step 1: Identify the formulas for kinetic energy
The total kinetic energy (KE) of a rolling object is the sum of its translational kinetic energy (KE_trans) and rotational kinetic energy (KE_rot):
KEtotal=KEtrans+KErot
Where:
KEtrans=12mv2
KErot=12Iω2
Step 2: Determine the moment of inertia and angular velocity
For a uniform thin ring, the moment of inertia (I) is given by:
I=mr2
Where:
- m is the mass of the ring
- r is the radius of the ring
Since the ring rolls without slipping, the relationship between linear velocity (v) and angular velocity (ω) is:
v=rω⇒ω=vr
Step 3: Substitute the values into the kinetic energy formulas
Substituting the expression for angular velocity into the rotational kinetic energy formula:
KErot=12I(vr)2
Substituting the moment of inertia:
KErot=12(mr2)(vr)2=12mv2r2r2=12mv2
Step 4: Combine the kinetic energy components
Now we can combine the translational and rotational kinetic energy:
KEtotal=KEtrans+KErot=12mv2+12mv2=mv2
Step 5: Substitute the values for mass and velocity
Given:
- Mass m=0.4kg
- Velocity v=10cm/s=0.1m/s
Now, substituting these values into the total kinetic energy formula:
KEtotal=mv2=0.4×(0.1)2=0.4×0.01=0.004J=4×10−3J
Final Answer
The kinetic energy of the ring is:
KEtotal=4×10−3J
---
The total kinetic energy (KE) of a rolling object is the sum of its translational kinetic energy (KE_trans) and rotational kinetic energy (KE_rot):
KEtotal=KEtrans+KErot
Where:
KEtrans=12mv2
KErot=12Iω2
For a uniform thin ring, the moment of inertia (I) is given by:
I=mr2
Where:
- m is the mass of the ring
- r is the radius of the ring
v=rω⇒ω=vr
Substituting the expression for angular velocity into the rotational kinetic energy formula:
KErot=12I(vr)2
Substituting the moment of inertia:
KErot=12(mr2)(vr)2=12mv2r2r2=12mv2
Now we can combine the translational and rotational kinetic energy:
KEtotal=KEtrans+KErot=12mv2+12mv2=mv2
Given:
- Mass m=0.4kg
- Velocity v=10cm/s=0.1m/s
KEtotal=mv2=0.4×(0.1)2=0.4×0.01=0.004J=4×10−3J
The kinetic energy of the ring is:
KEtotal=4×10−3J
Topper's Solved these Questions
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONBOOK - VMC MODULES ENGLISHCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - EFFICIENT
50 Videos
- SYSTEM OF A PARTICLES & ROTATIONAL MOTIONBOOK - VMC MODULES ENGLISHCHAPTER - SYSTEM OF A PARTICLES & ROTATIONAL MOTIONEXERCISE - IN-CHAPTER EXERCISE F
10 Videos
- UNITS, MEASUREMENTS & ERRORSBOOK - VMC MODULES ENGLISHCHAPTER - UNITS, MEASUREMENTS & ERRORSEXERCISE - IN - CHAPTER EXERCISE - B
10 Videos
Similar Questions
A uniform hollow sphere of mass 400 g rolls without slipping on a plane horizontal surface with its centre moving at a speed of 10 cm/s. Its kinetic energy is:
View SolutionA uniform hollow sphere of mass 400 g rolls without slipping on a plane horizontal surface with its centre moving at a speed of 10 cm/s. Its kinetic energy is:
View SolutionA unifrom sphere of mass 500 g rolls without slipping on a plants horizontal surface with its center moving at a speed of 5.00cm/s .It kinetic energy is :
View SolutionA uniform hollow sphere of mass 200 g rolls without slipping on a plane horizontal surface with its centre moving at a speed of 5.00 cm/s. Its kinetic energy is:
View SolutionA uniform hollow sphere of mass 200 g rolls without slipping on a plane horizontal surface with its centre moving at a speed of 5.00 cm/s. Its kinetic energy is:
View SolutionA uniform sphere of mass 500g rolls without slipping on a plane surface so that its centre moves at a speed of 0.02m/s.
The total kinetic energy of rolling sphere would be (in J)
View SolutionA uniform sphere of mass 200 g rolls without slipping on a plane surface so that its centre moves at a speed of 2.00 cm/s. Find its kinetic energy.
View Solution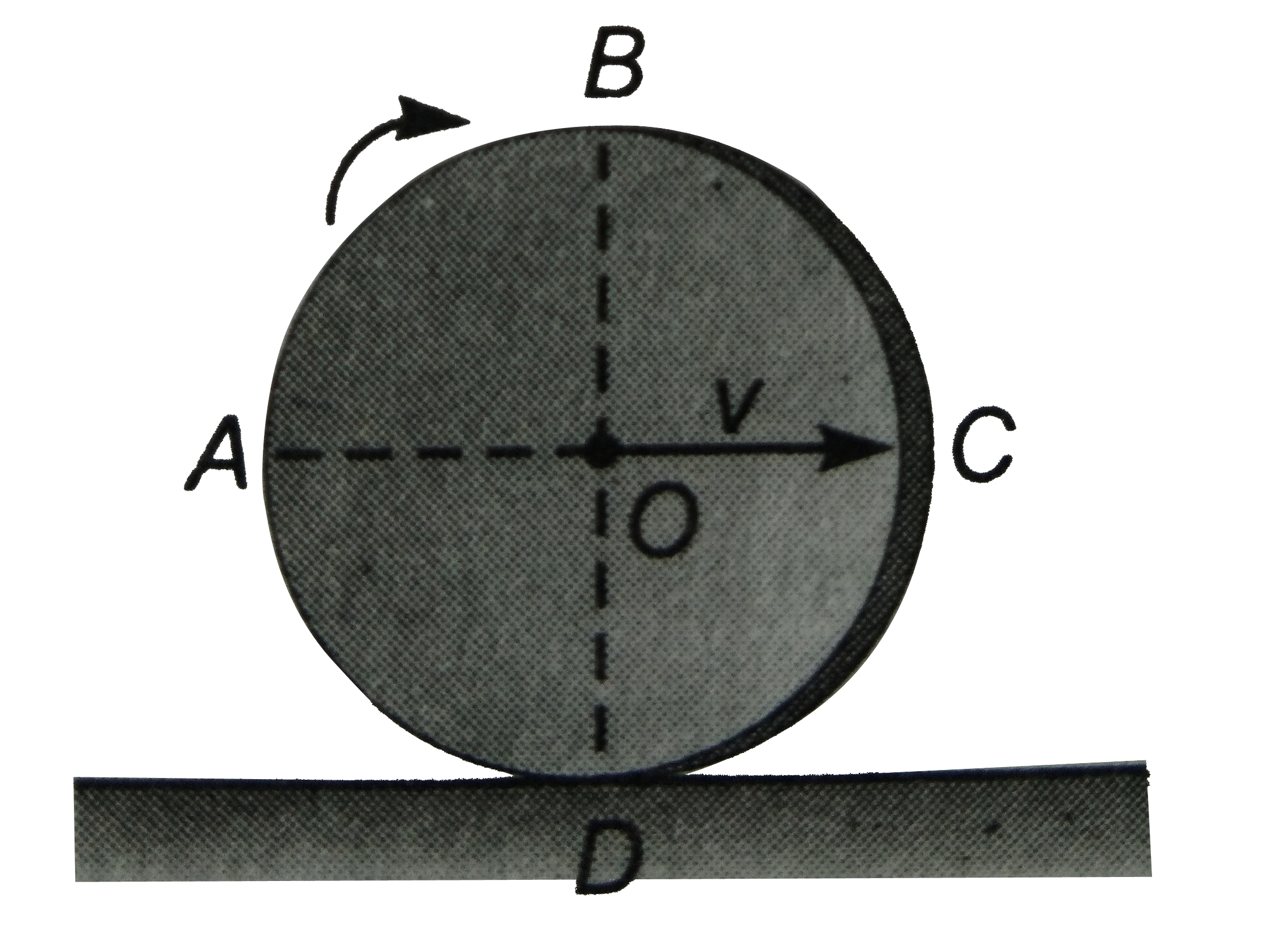
A uniform circular ring rolls without slipping on a horizontal surface. At any instant, its position is as shown in the figure. Then
View SolutionA ring rolls without slipping on a horizontal surface. At any instant its position is as shown in the figure.
View SolutionA wheel ( to be considered as a ring ) of mass m and radius R rolls without sliding on a horizontal surface with constant velocity v. It encounters a step of height R/2 at which it ascends without sliding
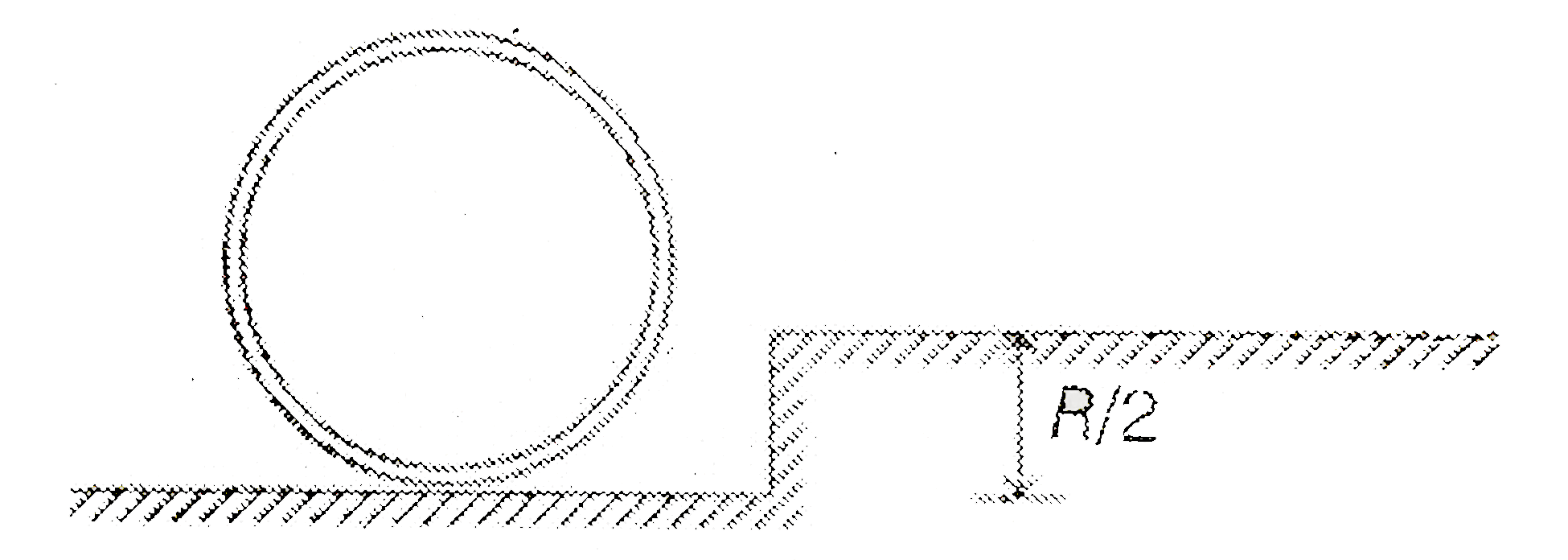
View Solution

