A metal disc of radius R rotates with an angular velcoity ω about an axis perpendiclar to its plane passing through its centre in a magnetic field B acting perpendicular to the plane of the disc. Calculate the induced emf between the rim and the axis of the disc.
pwBR2
wBR2
pwBR22
wBR22
The correct Answer is:D
To solve the problem of calculating the induced EMF between the rim and the axis of a rotating metal disc in a magnetic field, we can follow these steps:
1. Understanding the Setup:
- We have a metal disc of radius R rotating with an angular velocity ω about an axis perpendicular to its plane and passing through its center.
- A magnetic field B is acting perpendicular to the plane of the disc.
2. Induced EMF Formula:
- The induced EMF (E) in a circuit is given by the formula:
E=−dΦdt
where Φ is the magnetic flux.
3. Calculating Magnetic Flux:
- The magnetic flux Φ through the disc is given by:
Φ=B⋅A
where A is the area swept by the rotating disc. Initially, when the disc has not rotated, the area is 0.
4. Area Swept During Rotation:
- After one complete rotation, the area swept by the radius R is:
A=πR2
Therefore, the final magnetic flux after one complete rotation is:
Φfinal=B⋅πR2
5. Time Period of Rotation:
- The time period T for one complete rotation is given by:
T=2πω
6. Change in Magnetic Flux:
- The change in magnetic flux dΦ over the time period T is:
dΦ=Φfinal−Φinitial=B⋅πR2−0=B⋅πR2
7. Calculating Induced EMF:
- Now substituting dΦ and dt into the EMF formula:
E=−dΦdt=−B⋅πR2T
Substituting T=2πω:
E=−B⋅πR22πω=−B⋅πR2⋅ω2π
Simplifying this gives:
E=BR2ω2
8. Final Result:
- The induced EMF between the rim and the axis of the disc is:
E=BR2ω2
- We have a metal disc of radius R rotating with an angular velocity ω about an axis perpendicular to its plane and passing through its center.
- A magnetic field B is acting perpendicular to the plane of the disc.
- The induced EMF (E) in a circuit is given by the formula:
E=−dΦdt
where Φ is the magnetic flux.
- The magnetic flux Φ through the disc is given by:
Φ=B⋅A
where A is the area swept by the rotating disc. Initially, when the disc has not rotated, the area is 0.
- After one complete rotation, the area swept by the radius R is:
A=πR2
Therefore, the final magnetic flux after one complete rotation is:
Φfinal=B⋅πR2
- The time period T for one complete rotation is given by:
T=2πω
- The change in magnetic flux dΦ over the time period T is:
dΦ=Φfinal−Φinitial=B⋅πR2−0=B⋅πR2
- Now substituting dΦ and dt into the EMF formula:
E=−dΦdt=−B⋅πR2T
Substituting T=2πω:
E=−B⋅πR22πω=−B⋅πR2⋅ω2π
Simplifying this gives:
E=BR2ω2
- The induced EMF between the rim and the axis of the disc is:
E=BR2ω2
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTION & ALTERNATIVE CURRENT BOOK - VMC MODULES ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTION & ALTERNATIVE CURRENT EXERCISE - IMPECCABLE
52 Videos
- ELECTROMAGNETIC INDUCTION & ALTERNATIVE CURRENT BOOK - VMC MODULES ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTION & ALTERNATIVE CURRENT EXERCISE - ENABLE
50 Videos
- ELECTROMAGNETIC INDUCTION & ALTERNATING CURRENTBOOK - VMC MODULES ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTION & ALTERNATING CURRENTEXERCISE - IN-CHAPTER EXERCISE-G
10 Videos
- ELECTROSTATICSBOOK - VMC MODULES ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - JEE Advanced (Archive)
89 Videos
Similar Questions
A ring rotates with angular velocity ω about an axis perpendicular to the plane of the ring passing through the center of the ring.A constant magnetic field B exists parallel to the axis.Find the emf induced in the ring.
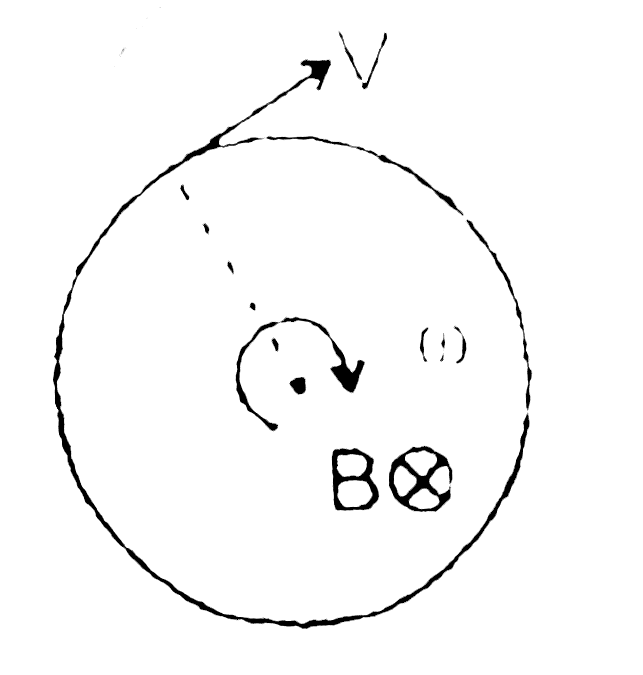
View SolutionA vertical disc of diameter 10 cm makes 20 revolutions per second about a horizontal axis passing through its centre. A uniform magnetic field 10−1 T acts perpendicular to the plane of the disc. Calculate the potential difference between its centre and rim in volts.
View Solutiona semicircle wire of radius R is rotated with constant angular velocity about an axis passing through one end and perpendicular to the plane of wire. There is a uniform magnetic field of strength B. The induced emf between the ends is
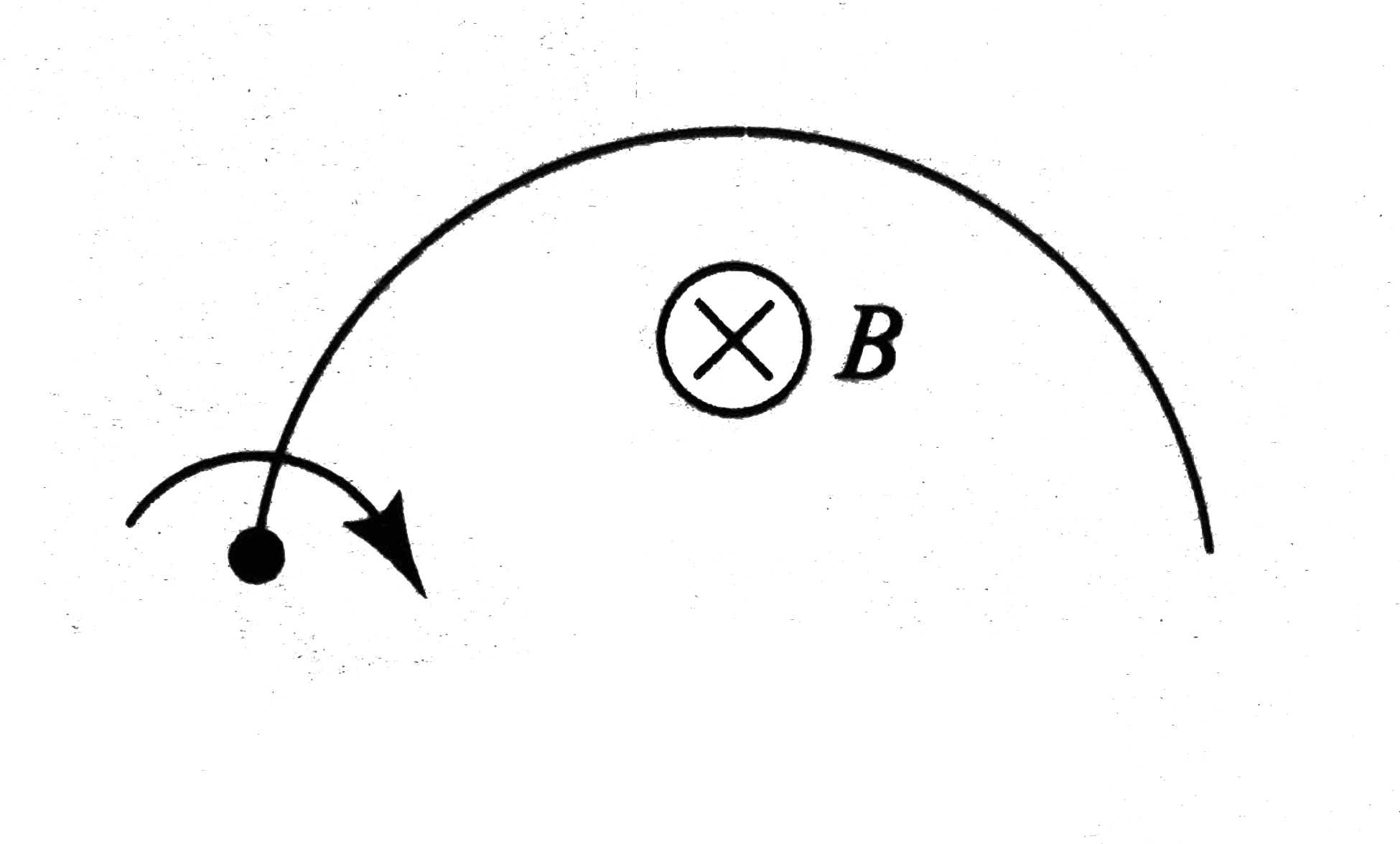
View Solutiona semicircle wire of radius R is rotated with constant angular velocity about an axis passing through one end and perpendicular to the plane of wire. There is a uniform magnetic field of strength B. The induced emf between the ends is

View SolutionA disc of radius R is pivoted at its rim. The period for small oscillations about an axis perpendicular to the plane of disc is
View SolutionThe moment of inertia of a copper disc, rotating about an axis passing through its centre and perpendicular to its plane
View SolutionA disc of mass m, radius r and carrying charge q, is rotating with angular speed ω about an axis passing through its centre and perpendicular to its plane. Calculate its magnetic moment
View SolutionA disc of mass m, radius r and carrying charge q, is rotating with angular speed ω about an axis passing through its centre and perpendicular to its plane. Calculate its magnetic moment
View SolutionA circular copper disc 100cm in radius rotates at 20πrad/s about an axis through its centre and perpendicular to the disc. A uniform magnetic field of 0.2T acts perpendicular to the disc. Calculate the potential difference developed between the axis of the disc and the rim
View SolutionA charge Q is uniformly distributed over the surface of non - conducting disc of radius R. The disc rotates about an axis perpendicular to its plane and passing through its centre with an angular to its plane and passing through its centre of the disc. If we keep both the amount of charge placed on the disc and its angular velocity to be constant and vary the radius of the disc then the variation of the magnetic induction at the centre of the disc will br represented by the figure:
View Solution
