If is the size of the magnified image and is the size of the diminished image in lens displacement method, then the size of the object is
The correct Answer is:A
To find the size of the object using the lens displacement method, we can follow these steps:
1. Understanding the Setup:
- We have two images formed by a convex lens: one is magnified (size x1) and the other is diminished (size x2).
- The distance between the object and the image is greater than 4f, where f is the focal length of the lens.
2. Defining Distances:
- Let d be the distance between the two positions of the lens.
- Let x be the distance from the lens to the object in the first position.
- The image distance for the first position is d−x and for the second position it is d+x.
3. Using Magnification:
- For the first position (magnified image):
Magnification(m1)=x1ho=d−xx
Rearranging gives:
ho=x⋅x1d−x(Equation 1)
- For the second position (diminished image):
Magnification(m2)=x2ho=d+xd−(d+x)=d+x−x
Rearranging gives:
ho=−x⋅x2d+x(Equation 2)
4. Equating the Two Expressions for ho:
- From Equation 1 and Equation 2, we can set them equal to each other:
x⋅x1d−x=−x⋅x2d+x
5. Cross Multiplying:
- Cross multiplying gives:
x⋅x1(d+x)=−x⋅x2(d−x)
6. Simplifying:
- Cancel x from both sides (assuming x≠0):
x1(d+x)=−x2(d−x)
- Rearranging gives:
x1d+x1x=−x2d+x2x
- Collecting like terms yields:
(x1+x2)x=−(x2−x1)d
7. Finding the Size of the Object:
- Solving for ho:
ho=√x1x2
Final Result:
The size of the object ho is given by:
ho=√x1x2
- We have two images formed by a convex lens: one is magnified (size x1) and the other is diminished (size x2).
- The distance between the object and the image is greater than 4f, where f is the focal length of the lens.
- Let d be the distance between the two positions of the lens.
- Let x be the distance from the lens to the object in the first position.
- The image distance for the first position is d−x and for the second position it is d+x.
- For the first position (magnified image):
Magnification(m1)=x1ho=d−xx
Rearranging gives:
ho=x⋅x1d−x(Equation 1)
Magnification(m2)=x2ho=d+xd−(d+x)=d+x−x
Rearranging gives:
ho=−x⋅x2d+x(Equation 2)
- From Equation 1 and Equation 2, we can set them equal to each other:
x⋅x1d−x=−x⋅x2d+x
- Cross multiplying gives:
x⋅x1(d+x)=−x⋅x2(d−x)
- Cancel x from both sides (assuming x≠0):
x1(d+x)=−x2(d−x)
- Rearranging gives:
x1d+x1x=−x2d+x2x
- Collecting like terms yields:
(x1+x2)x=−(x2−x1)d
- Solving for ho:
ho=√x1x2
The size of the object ho is given by:
ho=√x1x2
Topper's Solved these Questions
- RAY OPTICS AND WAVE OPTICS BOOK - VMC MODULES ENGLISHCHAPTER - RAY OPTICS AND WAVE OPTICS EXERCISE - EFFICIENT
49 Videos
- RAY OPTICS AND WAVE OPTICS BOOK - VMC MODULES ENGLISHCHAPTER - RAY OPTICS AND WAVE OPTICS EXERCISE - IMPECCABLE
58 Videos
- RAY OPTICS AND WAVE OPTICS BOOK - VMC MODULES ENGLISHCHAPTER - RAY OPTICS AND WAVE OPTICS EXERCISE - IMPECCABLE
58 Videos
- RAY OPTICS BOOK - VMC MODULES ENGLISHCHAPTER - RAY OPTICS EXERCISE - IN-CHAPTER EXERCISE-J
10 Videos
- REVISION TEST-15 JEE - 2020BOOK - VMC MODULES ENGLISHCHAPTER - REVISION TEST-15 JEE - 2020EXERCISE - PHYSICS
25 Videos
Similar Questions
If x1 is the size of the magnified image and x2 is the size of the diminsghed image in lens displacement method, then the size of the object is
View SolutionIf I1 and I2 be the size of the images respectively for the two positions of lens in the displacement method, then the size of the object is given by
View SolutionWhen the distance between the object and the screen is more than 4f. We can obtain image of the object on the screen for the two positions of the lens. If is called displacement method. In one case, the image is magnified and in the other case, it is diminished. If I1 and I2 be the sized of the two images, then the size of the object is
View SolutionA plane mirror is used to obtain an image of an object.
Compare the size of the image formed in it to the size of the object.
View SolutionFind the size of the image formed in the situation shown in figure.
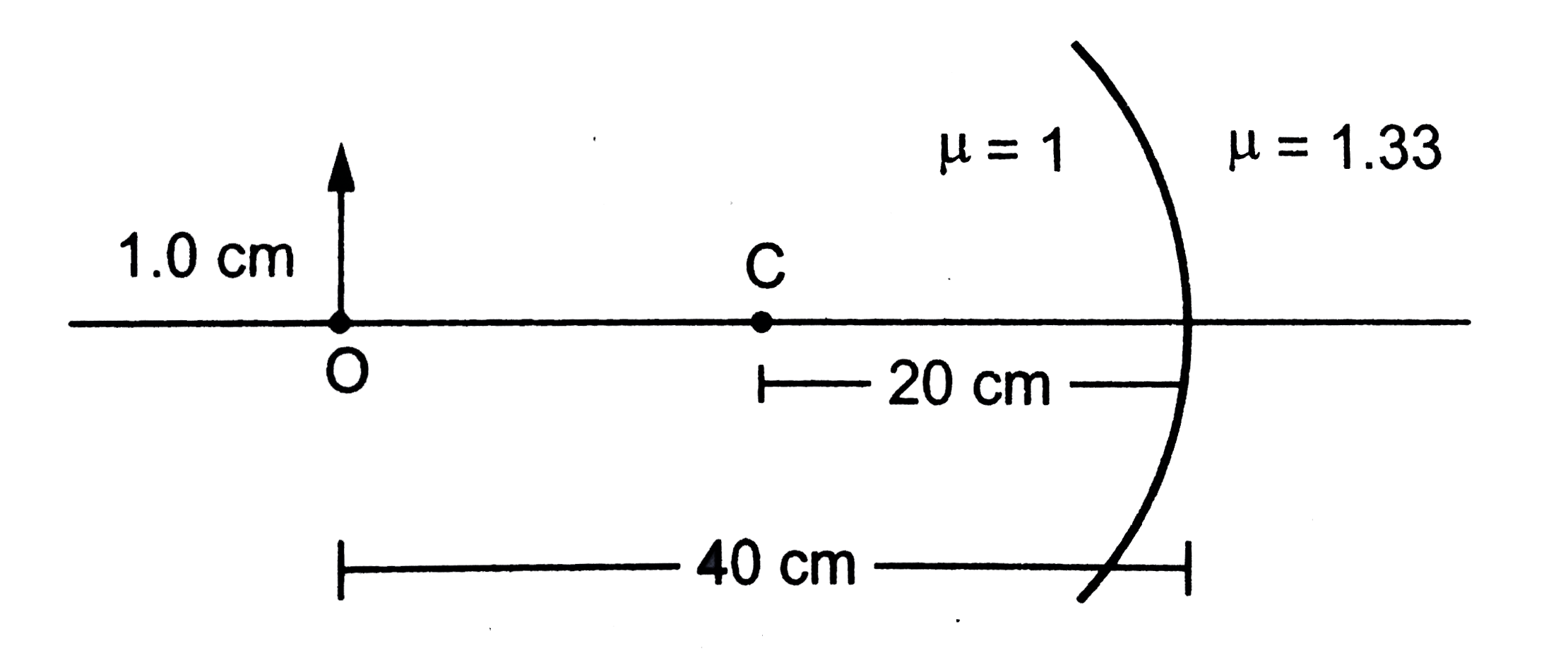
View SolutionAn object of size 2cm is placed at a distance of 40cm from a glass lens, close to the principal axis of the lens, and the lens forms an image of size 2cm. What is the size of the image and what type of image is formed if the lens and the object, in the same arrangement, is immersed into water ? The refractive index of the lens 1.5, and that of the water is 1.33.
View SolutionA concave mirror has a radius of curvature of 24 cm. How far is
an object from the mirror if an image is formed that is:
(a) virtual and 3.0 times the size of the object,
(b) real and 3.0 times the size of the object and
(c) real and 1/3 the size of the object?.
View Solution
What determines the size of a pinhole camera image?
View SolutionThe image of a candle flame formed by a lens is obtained on a screen placed on the other side of the lens. If the image is three times the size of the flame and the distance between lens and image is 80cm, at what distance should the candle be placed from the lens ? What is the nature of the image at a distance of 80cm from the lens ?
View SolutionAn object is placed 12cm in front of a lens to form an image on a screen. The size of the image is twice that of the object. What is the distance between the object and the image?
View Solution
