A particle starts from rest at t=0 and undergoes an acceleration as shown in figure.
Draw the velocity-time graph of the motion of the particle during the interval from zero to 4 second.
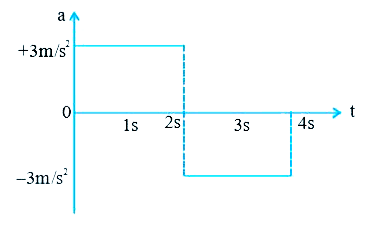
It follows from figure that between t = 0 to t = 2s, the acceleration of the particle is a=+3 m/s2. Since the particle starts from rest, u = 0. Therefore, the velocity of the particle during the time interval t = 0 and t = 2 is given by
v = u + at = 0 + 3t = 3t
Thus the velocity at t = 1 sec. is 3 m/s and at t = 2 s, the velocity is 6 m/s. During the time interval t = 3 s and t = 4 s, the initial velocity u = 6 m/s and acceleration is a=–3m/s2. Hence, during this time interval, the velocity of the particle is
v . = u + at . = 6 – 3t .
where t . = t – 2. Thus the velocity of the particle at t . = 1s or t = 3s is (6 – 3) = 3 m/s and t . = 2s or t = 4s, its velocity is (6 – 6) = 0.
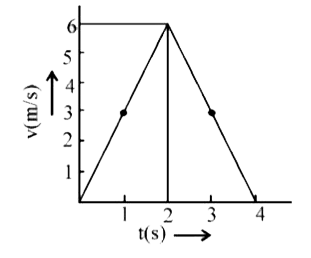
These result can be tabulated as follows:
Time (s) 0 1 2 3 4
Velocity (m/s) 0 3 6 3 0
Figure shows the velocity-time graph of the motion
Topper's Solved these Questions
- Motion in Straight Line BOOK - VMC MODULES ENGLISHCHAPTER - Motion in Straight Line EXERCISE - SOLVED EXAMPLES
20 Videos
- Motion in Straight Line BOOK - VMC MODULES ENGLISHCHAPTER - Motion in Straight Line EXERCISE - PRACTICE EXERCISE
80 Videos
- MOTION IN A STRAIGHT LINE & PLANE BOOK - VMC MODULES ENGLISHCHAPTER - MOTION IN A STRAIGHT LINE & PLANE EXERCISE - IMPECCABLE
52 Videos
- Motion in Two DimensionsBOOK - VMC MODULES ENGLISHCHAPTER - Motion in Two DimensionsEXERCISE - MCQ
2 Videos
Similar Questions
A particle starts from rest at t=0 and moves in a straight line with an acceleration as shown below. The velocity of the particle at t=3 s is

View SolutionA particle starts from rest with a time varying acceleration a=(2t−4). Here t is in second and a in m/s2
The velocity time graph of the particle is
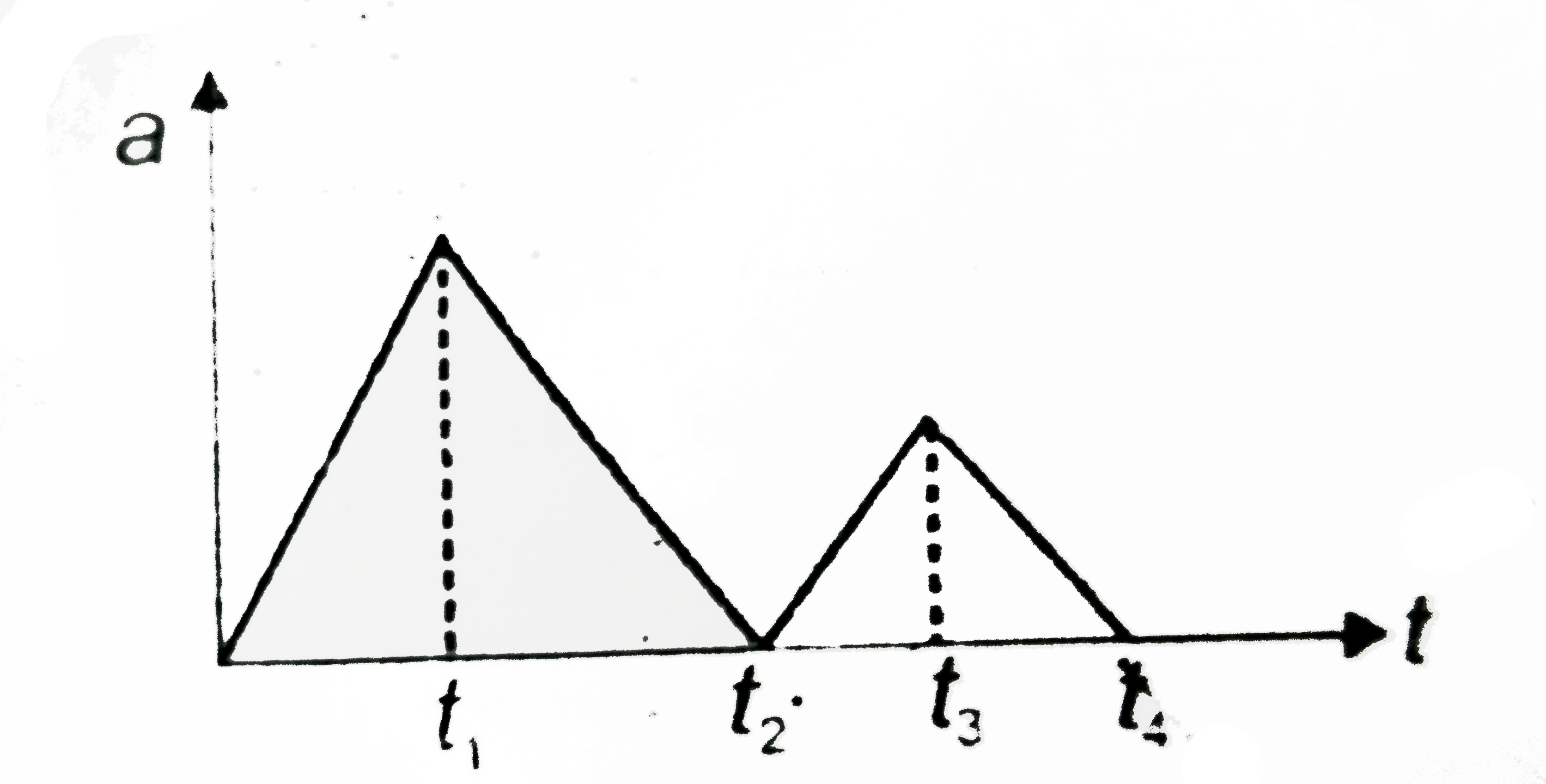
View SolutionA particle starting from rest moves in a straight line with acceleration as shown in the a-t graph. Find the distance (in m) travelled by the particle in the first four seconds from start of its motion.
Q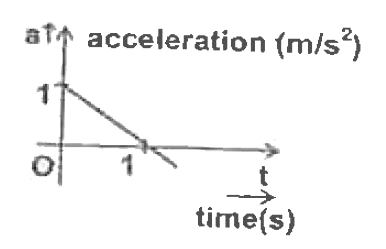
View SolutionA particle starts moving from rest on a straight line. Its acceleration a verses time t is shown in the figure. The speed of the particle is maximum at the instant
View SolutionA particle starts from rest at time t=0 and moves on a straight line with acceleration as plotted in fig. The speed of the particle will be maximum after time.

View SolutionA particle starts from rest and moves with constant acceleration. Then velocity displacement curve is:
View SolutionA particle starts motion from rest and moves along a straight line. Its velocity-time graph is shown. Find out speed of particle at t=2s and at t=3s.

View SolutionA particle starts from rest and moves with an acceleration of a={2+|t−2|}m/s2 The velocity of the particle at t=4 sec is
View SolutionA particle starts from rest and moves with an acceleration of a={2+|t−2|}m/s2 The velocity of the particle at t=4 sec is
View SolutionA particle starts from rest with constant acceleration. The ratio of space-average velocity to the time average velocity is :-
View Solution