The acceleration of an athlete running at 36 kmph when negotiating a turn of radius 25 m is
To solve the problem of finding the acceleration of an athlete running at 36 km/h while negotiating a turn of radius 25 m, we will follow these steps:
Step 1: Convert the speed from km/h to m/s
The speed given is 36 km/h. To convert this to meters per second (m/s), we use the conversion factor:
Speed (m/s)=Speed (km/h)×518
Calculating this:
Speed (m/s)=36×518=10 m/s
Step 2: Identify the radius of the turn
The radius of the turn is given as 25 m. We will denote this as r=25 m.
Step 3: Use the formula for centripetal acceleration
When an object moves in a circular path, it experiences centripetal acceleration, which can be calculated using the formula:
a=v2r
where v is the velocity and r is the radius of the circular path.
Step 4: Substitute the values into the formula
Substituting the values we have:
a=(10 m/s)225 m=100 m2/s225 m
Step 5: Calculate the acceleration
Now, performing the calculation:
a=10025=4 m/s2
Final Answer
The acceleration of the athlete while negotiating the turn is:
Acceleration=4 m/s2
---
The speed given is 36 km/h. To convert this to meters per second (m/s), we use the conversion factor:
Speed (m/s)=Speed (km/h)×518
Calculating this:
Speed (m/s)=36×518=10 m/s
The radius of the turn is given as 25 m. We will denote this as r=25 m.
When an object moves in a circular path, it experiences centripetal acceleration, which can be calculated using the formula:
a=v2r
where v is the velocity and r is the radius of the circular path.
Substituting the values we have:
a=(10 m/s)225 m=100 m2/s225 m
Now, performing the calculation:
a=10025=4 m/s2
The acceleration of the athlete while negotiating the turn is:
Acceleration=4 m/s2
Topper's Solved these Questions
- Motion in Straight Line BOOK - VMC MODULES ENGLISHCHAPTER - Motion in Straight Line EXERCISE - SOLVED EXAMPLES
20 Videos
- Motion in Straight Line BOOK - VMC MODULES ENGLISHCHAPTER - Motion in Straight Line EXERCISE - PRACTICE EXERCISE
80 Videos
- MOTION IN A STRAIGHT LINE & PLANE BOOK - VMC MODULES ENGLISHCHAPTER - MOTION IN A STRAIGHT LINE & PLANE EXERCISE - IMPECCABLE
52 Videos
- Motion in Two DimensionsBOOK - VMC MODULES ENGLISHCHAPTER - Motion in Two DimensionsEXERCISE - MCQ
2 Videos
Similar Questions
Figur shows the total acceleration and velocity of a particle moving clockwise in a circle of radius 2.5m at a given instant of time. At this instant, find:
(a) the radius acceleration,
(b) the speed of the acceleration,
(c) its tangential acceleration.
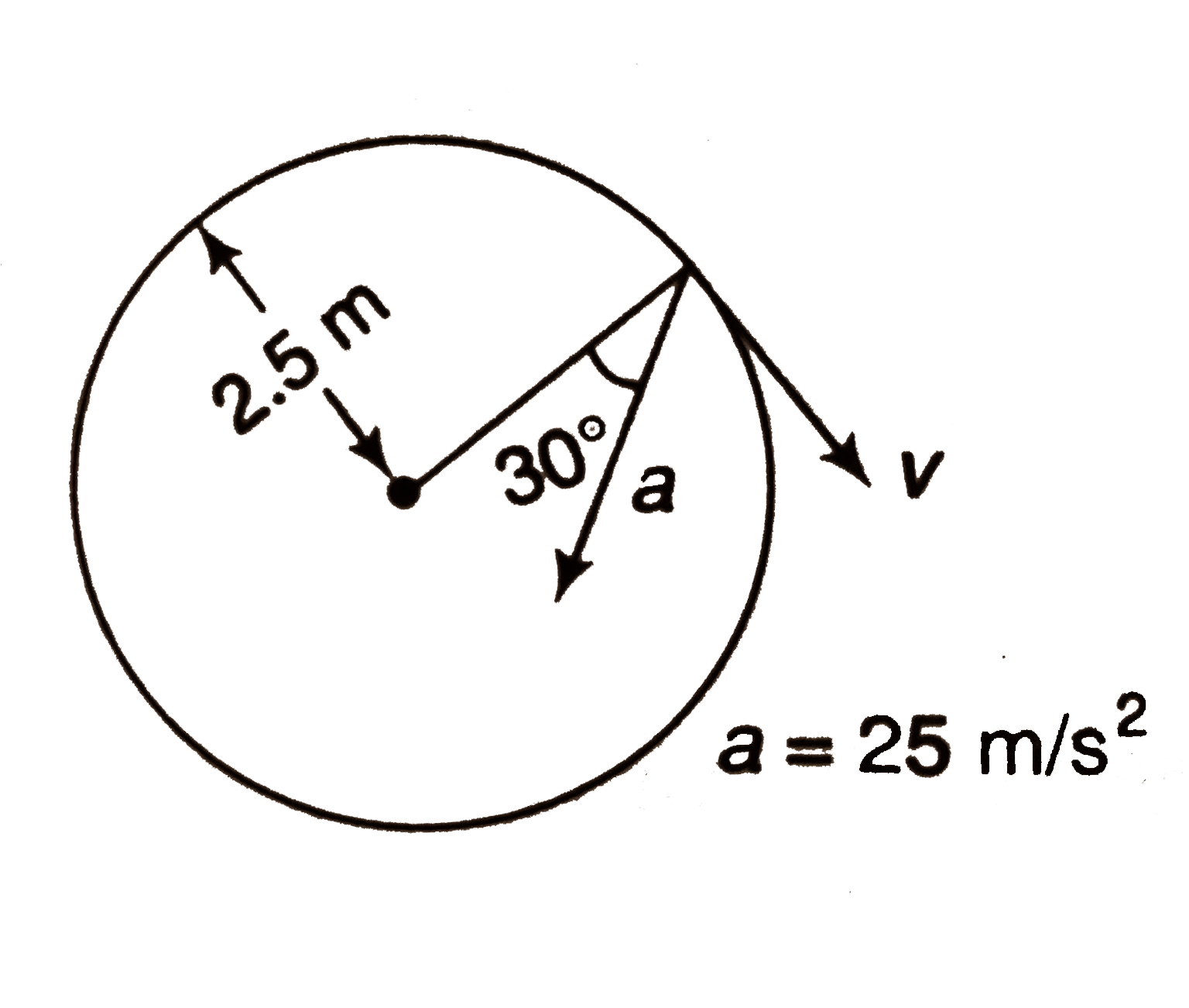
View SolutionCalculate the magnitude of linear acceleration of a particle moving in a circle of radius 0.5 m at the instant when its angular velocity is 2.5 rad s–1 and its angular acceleration is 6rads−2.
View SolutionCalculate the magnitude of linear acceleration of a particle moving in a circle of radius 0.5 m at the instant when its angular velocity is 2.5 rad s–1 and its angular acceleration is 6rads−2.
View SolutionA scooter weighing 150 g together with its rider moving at 36 km/hr is to take a turn of radius 30 m. What horizontal force on the scooter is needed to make the turn possible?
View SolutionA scooter weighing 150 kg together with its rider moving at 36 km/hr is to take a turn of radius 30 m. What horizontal force on the scooter is needed to make the turn possible?If the horizontal force needed for the turn in the previous problem is to be supplied by the normal force by the road what should be the proper angle of banking?
View SolutionA road is banked at an angle of 30∘ to the horizontal for negotiating a curve of radius 10√3 m. At what velocity will a car experience no friction while negotiating the curve? Take g=10ms−2
View SolutionThe centripetal force required by a 1000 kg car that takes a turn of radius 50 m at a speed of 36 kmph is
View SolutionThe distance between the inner and outer wheels of a car is 1.75 m and its centre of gravity is 0-50 m above the ground. What is the maximum speed with which the car can negotiate a curve of radius of 40 m without overturning ?
View SolutionA simple pendulum is suspended from the ceiling of a car taking a turn of radius 10 m at a speed of 36 km/h. Find the angle made by the string of the pendulum with the vertical if this angle does not change during the turn. Take g=10ms2.
View SolutionA jogger running at 9 kmph alongside a railway track is 240 metres ahead of the engine of a 120 metre long train running at 45 kmph in the same direction. In how much time will the train pass the jogger? 3.6 sec b. 36 sec c. 18 sec d. 72 sec
View Solution
