If the torque on a body is zero, then?
Jω=constant
Iω=constant
Iω22=constant
mv22=constant
The correct Answer is:B
To solve the question "If the torque on a body is zero, then?" we can follow these steps:
1. Understanding Torque:
- Torque (τ) is defined as the rate of change of angular momentum (L). Mathematically, this is expressed as:
τ=dLdt
2. Setting Torque to Zero:
- According to the problem, the torque on the body is zero:
τ=0
3. Implication of Zero Torque:
- If the torque is zero, then the rate of change of angular momentum must also be zero:
dLdt=0
- This implies that the angular momentum (L) is constant over time.
4. Relating Angular Momentum to Moment of Inertia and Angular Velocity:
- Angular momentum can be expressed in terms of moment of inertia (I) and angular velocity (ω):
L=I⋅ω
5. Conclusion:
- Since L is constant and L=I⋅ω, it follows that the product of moment of inertia and angular velocity must also be constant. Therefore, if the moment of inertia changes, the angular velocity must change in such a way that their product remains constant.
Final Answer:
If the torque on a body is zero, then the angular momentum of the body is constant, which implies that the product of moment of inertia and angular velocity is also constant.
---
- Torque (τ) is defined as the rate of change of angular momentum (L). Mathematically, this is expressed as:
τ=dLdt
- According to the problem, the torque on the body is zero:
τ=0
- If the torque is zero, then the rate of change of angular momentum must also be zero:
dLdt=0
- This implies that the angular momentum (L) is constant over time.
- Angular momentum can be expressed in terms of moment of inertia (I) and angular velocity (ω):
L=I⋅ω
- Since L is constant and L=I⋅ω, it follows that the product of moment of inertia and angular velocity must also be constant. Therefore, if the moment of inertia changes, the angular velocity must change in such a way that their product remains constant.
If the torque on a body is zero, then the angular momentum of the body is constant, which implies that the product of moment of inertia and angular velocity is also constant.
Topper's Solved these Questions
- SYSTEM OF A PARTICLES & ROTATIONAL MOTIONBook:VMC MODULES ENGLISHChapter:SYSTEM OF A PARTICLES & ROTATIONAL MOTIONExercise:IN-CHAPTER EXERCISE E
Explore 10 Videos
- SYSTEM OF A PARTICLES & ROTATIONAL MOTIONBook:VMC MODULES ENGLISHChapter:SYSTEM OF A PARTICLES & ROTATIONAL MOTIONExercise:IN-CHAPTER EXERCISE F
Explore 10 Videos
- SYSTEM OF A PARTICLES & ROTATIONAL MOTIONBook:VMC MODULES ENGLISHChapter:SYSTEM OF A PARTICLES & ROTATIONAL MOTIONExercise:IN-CHAPTER EXERCISE C
Explore 10 Videos
- SIMPLE HARMONIC MOTION Book:VMC MODULES ENGLISHChapter:SIMPLE HARMONIC MOTION Exercise:7-previous year question
Explore 46 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONBook:VMC MODULES ENGLISHChapter:SYSTEM OF PARTICLES AND ROTATIONAL MOTIONExercise:IMPECCABLE
Explore 56 Videos
Similar Questions
A : For a body to be in rotational equilibrium the net torque acting on the body about any point is zero.
R : For net torque to be zero, net force should also be zero.
View SolutionA body is in translational equilibrium under the sctin of coplanar forces. If the torque of these force is zero about a point is it necessary that it will also be zero abut any other point?
View Solution
Knowledge Check
Assertion: If external force on a body is zero, its acceleration is zero.
Reason: This is the simple from of Newton's second law of motion.AIf both assertion and reason are true and reason is the correct explanation of assertion.BIf both assertion and reason are true and reason is the not correct explanation of assertion.CIf assertion is true but reason is falseDIf both assertion and reason are false.The torque acting on a body is the rotational, analogue of
Amass of the bodyBlinear kinetic energy of the bodyClinear velocity of the bodyDforce in linear motion
In net force on a rigid body is zero. Then, match the following table.
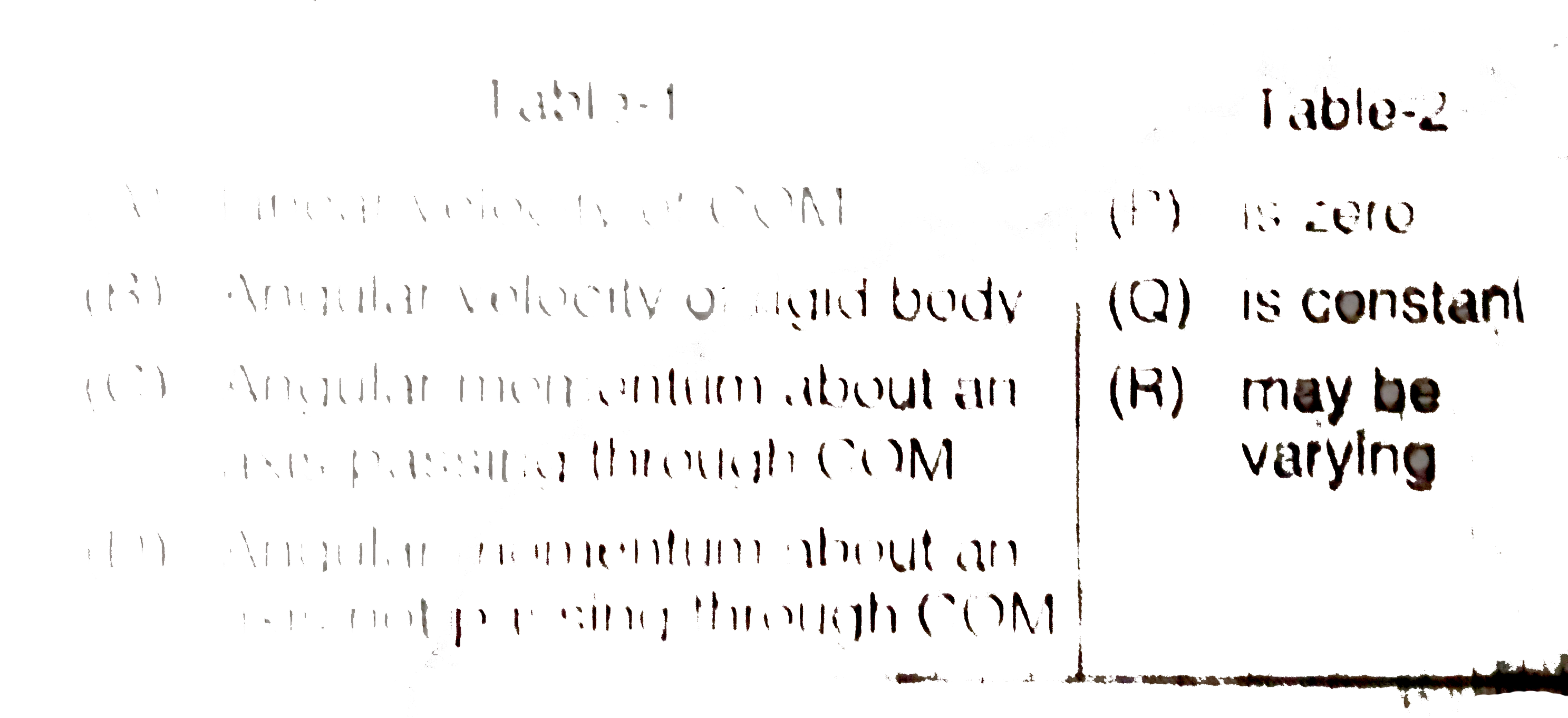
View SolutionIt torque is zero, then
View SolutionWhich of following statements are correct ? l (a) Centre of mass of a body always coincides with the centre of gravity of the body
(b) Central of mass of a body is the point at which the total garvitational torque on the body is zero
(c ) Couple on a body produces both trasnlational and rotation motion in a body
(d) Mechinical advantage greater than one means that small efforts can be used to lift a large load
View SolutionIf the torque acting on a system is zero then, which of the followin quantities is conserced ?
View SolutionWhat are the two factors on which the torque applied on a body depends?
View SolutionShow that the torque acting on a body is equal to the product of moment of inertia and the angular acceleration of the body.
View Solution
