The frequency distribution of marks obtained by 28 students in a test carrying 40 marks is given below:
Marks:0−1010−2020−3030−40Number of students:6xy6
If the mean of the above data is 20, then the difference between x and y is
3
2
1
0
The correct Answer is:D
To solve the problem step by step, we will follow the outlined process:
Step 1: Set up the frequency distribution table
The frequency distribution of marks obtained by 28 students is given as follows:
| Marks | Number of Students (Frequency) |
|----------|-------------------------------|
| 0 - 10 | 6 |
| 10 - 20 | x |
| 20 - 30 | y |
| 30 - 40 | 6 |
Step 2: Calculate total frequency
The total number of students is given as 28. Therefore, we can write the equation for total frequency:
6+x+y+6=28
Simplifying this gives:
x+y+12=28
Thus,
x+y=16(Equation 1)
Step 3: Calculate midpoints and frequency times midpoints
Next, we calculate the midpoints (x_i) for each class interval:
- For 0-10: 0+102=5
- For 10-20: 10+202=15
- For 20-30: 20+302=25
- For 30-40: 30+402=35
Now we calculate fi×xi:
| Marks | Frequency (f_i) | Midpoint (x_i) | fi×xi |
|----------|-----------------|----------------|-----------------------|
| 0 - 10 | 6 | 5 | 6×5=30 |
| 10 - 20 | x | 15 | 15x |
| 20 - 30 | y | 25 | 25y |
| 30 - 40 | 6 | 35 | 6×35=210 |
Step 4: Calculate the mean
The mean is given as 20. The formula for mean is:
Mean=Σ(fi×xi)Σfi
Substituting the values we have:
20=30+15x+25y+21028
Multiplying both sides by 28 gives:
560=30+15x+25y+210
Simplifying this leads to:
560=240+15x+25y
Thus,
15x+25y=560−240
This simplifies to:
15x+25y=320(Equation 2)
Step 5: Solve the system of equations
We now have two equations:
1. x+y=16 (Equation 1)
2. 15x+25y=320 (Equation 2)
From Equation 1, we can express y in terms of x:
y=16−x
Substituting this into Equation 2:
15x+25(16−x)=320
Expanding this gives:
15x+400−25x=320
Combining like terms results in:
−10x+400=320
Solving for x:
−10x=320−400
−10x=−80
x=8
Now substituting x back into Equation 1 to find y:
8+y=16
y=16−8=8
Step 6: Find the difference between x and y
Now we find the difference between x and y:
Difference=x−y=8−8=0
Final Answer
The difference between x and y is 0.
---
The frequency distribution of marks obtained by 28 students is given as follows:
|----------|-------------------------------|
| 0 - 10 | 6 |
| 10 - 20 | x |
| 20 - 30 | y |
| 30 - 40 | 6 |
The total number of students is given as 28. Therefore, we can write the equation for total frequency:
Next, we calculate the midpoints (x_i) for each class interval:
- For 10-20: 10+202=15
- For 20-30: 20+302=25
- For 30-40: 30+402=35
|----------|-----------------|----------------|-----------------------|
| 0 - 10 | 6 | 5 | 6×5=30 |
| 10 - 20 | x | 15 | 15x |
| 20 - 30 | y | 25 | 25y |
| 30 - 40 | 6 | 35 | 6×35=210 |
The mean is given as 20. The formula for mean is:
We now have two equations:
2. 15x+25y=320 (Equation 2)
−10x=−80
x=8
y=16−8=8
Now we find the difference between x and y:
The difference between x and y is 0.
Topper's Solved these Questions
- MEASURES OF CENTRAL TENDENCYBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - MEASURES OF CENTRAL TENDENCYEXERCISE - Exercise
40 Videos
- MAXIMA AND MINIMA BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - MAXIMA AND MINIMA EXERCISE - Chapter Test
29 Videos
- MISCELLANEOUS EQUATIONS AND INEQUATIONS BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - MISCELLANEOUS EQUATIONS AND INEQUATIONS EXERCISE - Chapter Test
29 Videos
Similar Questions
The frequency distribution of the marks obtained by 100 students in a test carrying 50 marks is given below. Then the mean is
Marks 0−910−1920−2930−3940−49No. of students 8 15 20 45 12
View SolutionIf for somex∈R, the frequency distribution of the marks obtained by 20 students in a test is:
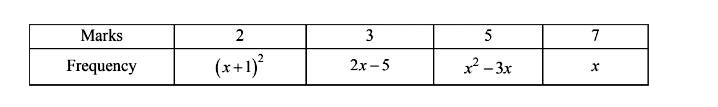
Then the mean of the marks is:
View SolutionFind the mode of the following distribution of marks obtained by 80 students: Marks obtained 0-10 10-20 20-30 30-40 40-50 No. of students 6 10 12 32 20
View SolutionFind the mode of the following distribution of marks obtained by 80 students: Marks obtained 0-10 10-20 20-30 30-40 40-50 No. of students 6 10 12 32 20
View SolutionFind the mode of the following distribution of marks obtained by 80 students: Marks obtained 0-10 10-20 20-30 30-40 40-50 No. of students 6 10 12 32 20
View SolutionFind the mode of the following frequency distribution of marks obtained by 50 students. Marks obtained 0−10 10−20 20−30 30−40 40−50 No. of students 5 12 20 10 3
View SolutionFind the mean for the following data.
Marks obtained10−2020−3030−4040−5050−6060−70Number of students8612527
View SolutionFollowing is the distribution fo marks obtained by 50 students in a test.
Marks more than01020304050No. of Students50464020103
If 60% of the students pass the test, find the minimum marks obtained by a pass students.
View SolutionCalculate the mean devation from the mean the following data:
Marks0−1010−2020−3030−4040−50No. of stu.5815166
View SolutionThe marks scored by 55 students in a test are given below: Marks 0-5 5-10 10-15 15-20 20-25 25-30 30-35 No. of Students 2 6 13 17 11 4 2 Construct a cumulative frequency distribution.
View Solution
