Two rods of lengths slide along the and y-axis , respectively, in such a manner that their ends are concyclic. Find the locus of the center of the circle passing through the endpoints.
The correct Answer is:B
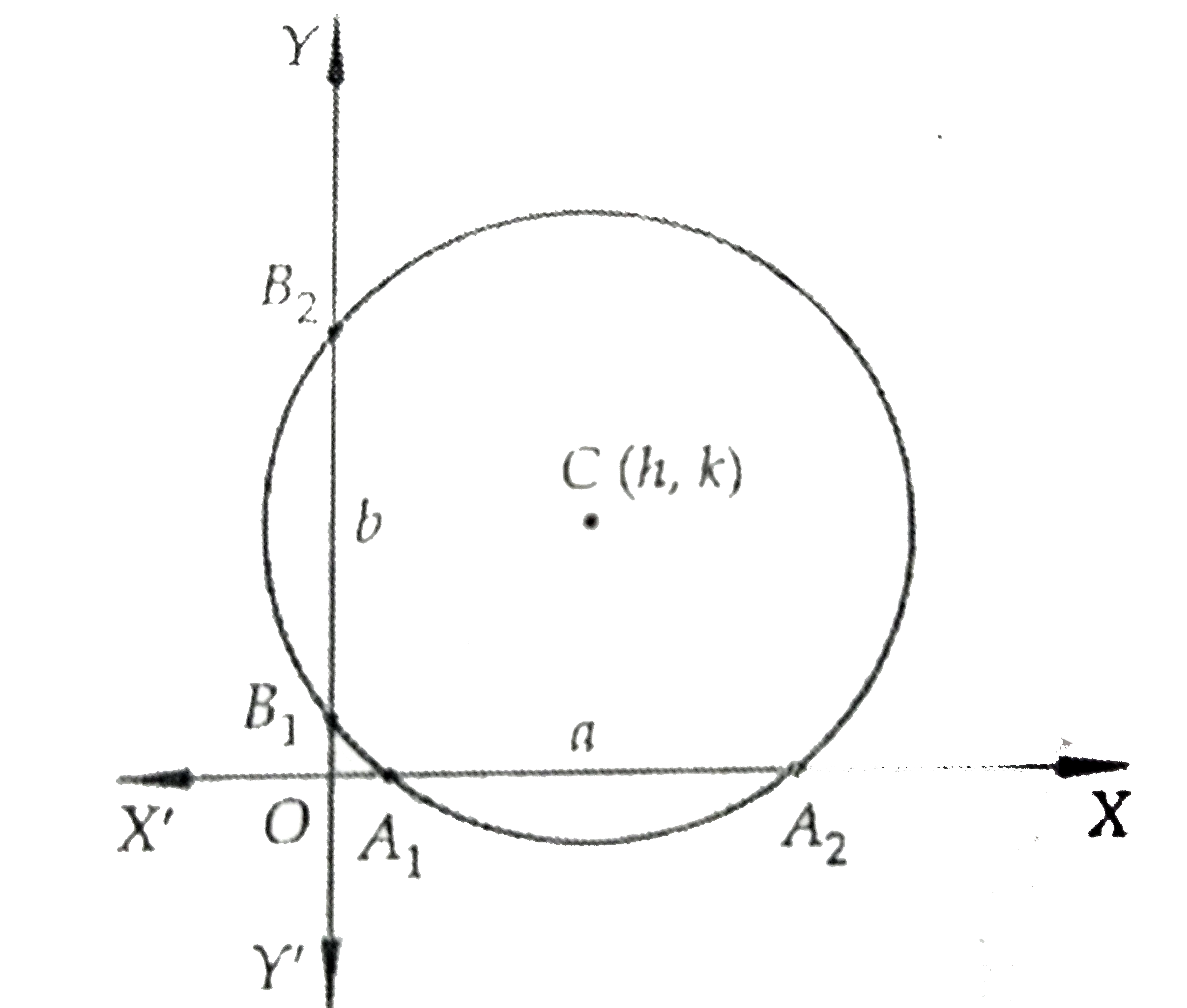
Let and be two rods of lengths a and b which slide along OX and OY respectively. Let be the circle passing through and . Then,
= Intercept on y-axis
And,
Intercept on y-axis
Here, c is the variable which is to be eliminated to find the locus of the centre (-g, -f) of the circle .
Eliminating c from (i) and (ii), we get
Thus, the locus of (-g, -f) i.e. the centre of the circle is
Topper's Solved these Questions
- CIRCLESBook:OBJECTIVE RD SHARMA ENGLISHChapter:CIRCLESExercise:Section I - Solved Mcqs
Explore 108 Videos
- CIRCLESBook:OBJECTIVE RD SHARMA ENGLISHChapter:CIRCLESExercise:Section-I (Solved MCQs)
Explore 1 Video
- CARTESIAN PRODUCT OF SETS AND RELATIONS Book:OBJECTIVE RD SHARMA ENGLISHChapter:CARTESIAN PRODUCT OF SETS AND RELATIONS Exercise:Chapter Test
Explore 30 Videos
- COMPLEX NUMBERS Book:OBJECTIVE RD SHARMA ENGLISHChapter:COMPLEX NUMBERS Exercise:Chapter Test
Explore 58 Videos
Similar Questions
If one end of the diameter is (1, 1) and the other end lies on the line x+y=3 , then find the locus of the center of the circle.
View SolutionIf one end of the diameter is (1, 1) and the other end lies on the line x+y=3 , then find the locus of the center of the circle.
View SolutionIf the intercepts of the variable circle on the x- and y-axis are 2 units and 4 units, respectively, then find the locus of the center of the variable circle.
View SolutionIf the intercepts of the variable circle on the x- and yl-axis are 2 units and 4 units, respectively, then find the locus of the center of the variable circle.
View SolutionTwo rods of equal lengths(l) and equal mass M are kept along x and y axis respectively such that their centre of mass lie at origin. The moment of inertia about an line y = x, is
View SolutionFind the equation of the circle with radius 5 whose center lies on the x-axis and passes through the point (2, 3).
View SolutionA variable chord is drawn through the origin to the circle x2+y2−2ax=0 . Find the locus of the center of the circle drawn on this chord as diameter.
View SolutionA circle passes through A(1,1) and touches x-axis then the locus of the other end of the diameter through A is
View SolutionAn iron rod of length 2l is sliding on two mutually perpendicular lines . Find the locus of the midpoint of the rod.
View SolutionA circle touching the X-axis at (3, 0) and making a intercept of length 8 on the Y-axis passes through the point
View Solution
