A solid sphere of volume V and density ρ floats at the interface of two immiscible liquids of densities ρ1andρ2 respectively. If ρ1<ρ<ρ2, then the ration of volume of the parts of the sphere in upper and lower liquids is :Solution in Malayalam
ρ−ρ2ρ2−ρ
ρ2−ρρ−ρ1
ρ+ρ1ρ+ρ2
ρ+ρ2ρ+ρ1
The correct Answer is:A
Let V1andV2 be the volumes, then
V1+V2=V
As ball is floating.
Weight of ball = upthrust on ball due to two liquids
Vρg=V1ρ1g+V2ρ2g
⇒ Vρ=V1ρ1+(V−V1)ρ2
⇒ V1=(ρ−ρ2ρ1−ρ2)
Fraction in upper part =V1V=ρ−ρ2ρ−ρ2
Fraction in lower part = 1−V1V=1−ρ−ρ2ρ1−ρ2
=ρ1−ρρ1−ρ2
∴ Ratio of lower and upper parts =ρ−ρ2ρ1−ρ
Similar Questions
A solid uniform ball of volume V floats on the interface of two immiscible liquids. The specific gravity of the upper liquid is rho_(1) and that of lower one is rho_(2) and the specific gravity of ball is rho(rho_(1) lt rho lt rho_(2)) . The fraction of the ball in the upper liquid Is
View SolutionA cylindrical block floats vertically in a liquid of density rho_(1) kept in a container such that the fraction of volume of the cylinder inside the liquid is x_(1) . Then some amount of another immiscible liquid of density rho_(2) (rho_(2) lt rho_(1)) is added to the liquid in the container so that the cylinder now floats just fully immersed in the liquids with x_(2) fraction of volume of the cylinder inside the liquid of density rho_(1) . The ratio rho_(1)//rho_(2) will be
04:38View SolutionVolume V and Density rho A solid of two liquids whose density rho_(1) And rho_(2) Yes, I am swimming in it. If rho_(1)lt rho lt rho_(2) , Then the ratio of the upper volume and lower volume of the sphere will be
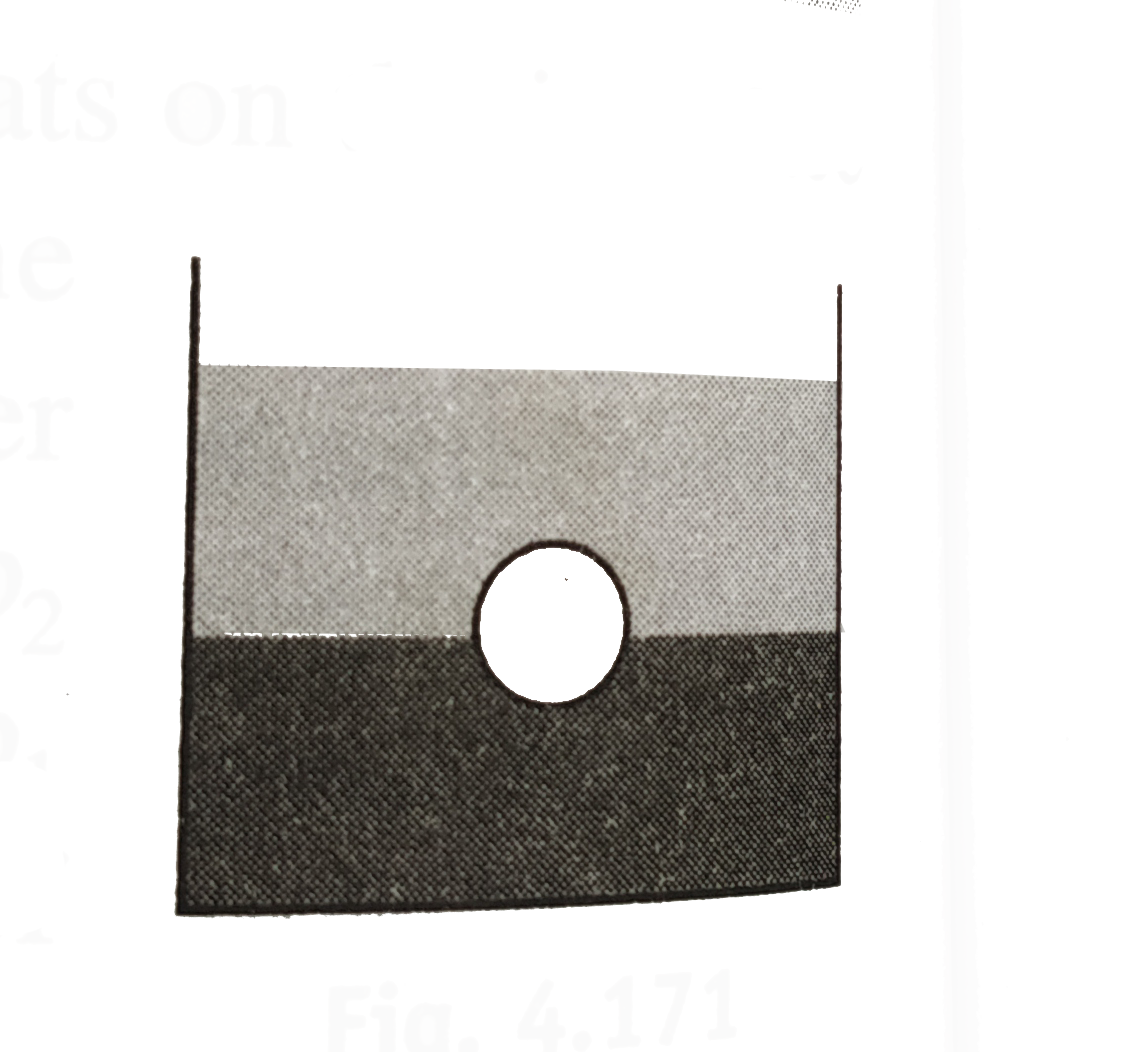
03:28View SolutionA solid uniform ball having volume V and density rho floats at the interface of two unmixible liquids as shown in Fig. 7(CF).7. The densities of the upper and lower liquids are rho_(1) and rho_(2) respectively, such that rho_(1) lt rho lt rho_(2) . What fractio9n of the volume of the ball will be in the lower liquid.
03:59View SolutionA body of density ρ floats with volume V1 of its total volume V immersed in a liquid of density ρ1 and with the remainder of volume V2 immersed in another liquid of density ρ2 where ρ1>ρ2. Find the volume immersed in two liquids. (V1 and V2).
03:02View SolutionA solid uniform ball of volume V floats on the interface of two immiscible liquids (see the figure). The specific gravity of the upper liquid is ρ1 and that of lower one is ρ2 and the specific gravity of ball is ρ(ρ1>ρ>ρ2) The fraction of the volume the ball in the u of upper liquid is
 03:51View Solution
03:51View SolutionA solid sphere having volume V and density ρ floats at the interface of two immiscible liquids of densites ρ1 and ρ2 respectively. If ρ1<ρ<ρ2, then the ratio of volume of the parts of the sphere in upper and lower liquid is
03:20View SolutionA solid uniform ball of volume V floats on the interface of two immiscible liquids (see the figure). The specific gravity of the upper liquid is ρ1 and that of lower one is ρ2 and the specific gravity of ball is ρ(ρ1>ρ>ρ2) The fraction of the volume the ball in the upper liquid is
 04:21View Solution
04:21View Solution

