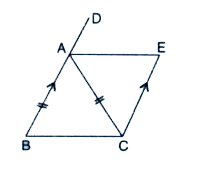
Equilateral triangles ABD and ACE are drawn on sides AB and AC respectively of a ΔABC outside it. Prove that :
(i) ∠DAC=∠EAB (ii) DC=BE
To solve the problem, we need to prove two statements about the equilateral triangles ABD and ACE drawn on the sides AB and AC of triangle ABC.
Step 1: Understand the Setup
We have triangle ABC with equilateral triangles ABD and ACE constructed on sides AB and AC respectively.
Step 2: Identify Angles in Equilateral Triangles
Since both triangles ABD and ACE are equilateral:
- ∠DAB=60∘
- ∠EAC=60∘
Step 3: Express Angles in Terms of Other Angles
Using the angle subtraction formula:
- ∠DAB=∠DAC−∠BAC
- ∠EAC=∠EAB−∠BAC
Step 4: Set Up the Equation
Since both ∠DAB and ∠EAC equal 60∘:
∠DAC−∠BAC=60∘
∠EAB−∠BAC=60∘
Step 5: Equate the Two Expressions
From the above equations, we can equate:
∠DAC−∠BAC=∠EAB−∠BAC
Step 6: Simplify the Equation
Subtract ∠BAC from both sides:
∠DAC=∠EAB
This proves the first part of the question.
Step 7: Prove the Second Part
To prove DC=BE, we will use triangle congruence.
Step 8: Identify the Triangles
Consider triangles ACD and BAE:
- AD=AB (sides of equilateral triangle ABD)
- AE=AC (sides of equilateral triangle ACE)
- ∠DAC=∠EAB (proven in step 6)
Step 9: Apply the Side-Angle-Side (SAS) Congruence Criterion
Since:
- AD=AB
- AE=AC
- ∠DAC=∠EAB
By the SAS criterion, triangles ACD and BAE are congruent.
Step 10: Use Corresponding Parts of Congruent Triangles (CPCTC)
From the congruence of triangles ACD and BAE, we have:
CD=BE
This proves the second part of the question.
Final Conclusion
We have successfully proven both parts:
(i) ∠DAC=∠EAB
(ii) DC=BE
We have triangle ABC with equilateral triangles ABD and ACE constructed on sides AB and AC respectively.
Since both triangles ABD and ACE are equilateral:
- ∠DAB=60∘
- ∠EAC=60∘
Using the angle subtraction formula:
- ∠DAB=∠DAC−∠BAC
- ∠EAC=∠EAB−∠BAC
Since both ∠DAB and ∠EAC equal 60∘:
∠DAC−∠BAC=60∘
∠EAB−∠BAC=60∘
From the above equations, we can equate:
∠DAC−∠BAC=∠EAB−∠BAC
Subtract ∠BAC from both sides:
∠DAC=∠EAB
This proves the first part of the question.
To prove DC=BE, we will use triangle congruence.
Consider triangles ACD and BAE:
- AD=AB (sides of equilateral triangle ABD)
- AE=AC (sides of equilateral triangle ACE)
- ∠DAC=∠EAB (proven in step 6)
Since:
- AD=AB
- AE=AC
- ∠DAC=∠EAB
From the congruence of triangles ACD and BAE, we have:
CD=BE
This proves the second part of the question.
We have successfully proven both parts:
(i) ∠DAC=∠EAB
(ii) DC=BE
Topper's Solved these Questions
- TRIANGLESBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - TRIANGLESEXERCISE - Exercise 7b
17 Videos
- TRIANGLESBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - TRIANGLESEXERCISE - Revision Exercise (very Short Answer Questions)
10 Videos
- TRIANGLESBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - TRIANGLESEXERCISE - Problems From NCERT/exemplar
5 Videos
- SURFACE AREA AND VOLUMEBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - SURFACE AREA AND VOLUMEEXERCISE - Revision Exercise (long Answer Questions)
10 Videos
Similar Questions
The given figure shows a parallelogram ABCD. Squares ABPQ and ADRS are drawn on sides AB and AD of the parallelogram ABCD. Prove that :
(i) ∠SAQ=∠ABC
(ii) SQ = AC
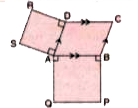
View SolutionOn the sides AB and AC of triangle ABC, equilateral triangles ABD and ACE are drawn. Prove that :
(i) ∠CAD=∠BAE
(ii) CD = BE
View SolutionIn triangle ABC, D and E are mid-points of sides AB and BC respectively. Also, F is a point in side AC so that DF is parallel to BC
(i) Prove that DBEF is a parallelogram.
View SolutionL and M are the mid-points of sides AB and DC respectively of parallelogram ABCD. Prove that segments DL and BM trisect diagonal AC.
View SolutionIn the given triangle P, Q and R are the mid points of sides AB, BC and AC respectively. Prove that triangle PQR is similar to triangle ABC.

View SolutionIn ΔABC,D,E and F are mid-point of sides AB ,BC and AC respectively , Prove that AE and DF bisect each other.
View SolutionIn the adjoining figure, BM and CN are the altitudes drawn to the sides AC and AB respectively of ΔABC. If BM=CN, prove that ΔABC is isosceles.

View SolutionThe sides AB and AC are equal of an isosceles triangle ABC. D E and F are the mid-points of sides BC, CA and AB respectively. Prove that:
(i) Line segment AD is perpedicular to line segment EF.
(ii) Line segment AD bisects the line segment EF.
View SolutionOn the sides AB and AC of triangle ABC, equilateral triangles ABD and ACE are drawn. Prove that :
∠CAD=∠BAE
View SolutionIn the given figure, AB//EC,AB=ACandAE bisects ∠DAC. Prove that:
(i) ∠EAC=∠ACB
(ii) ABCE is a parallelogram.
 .
.
View Solution
