Question

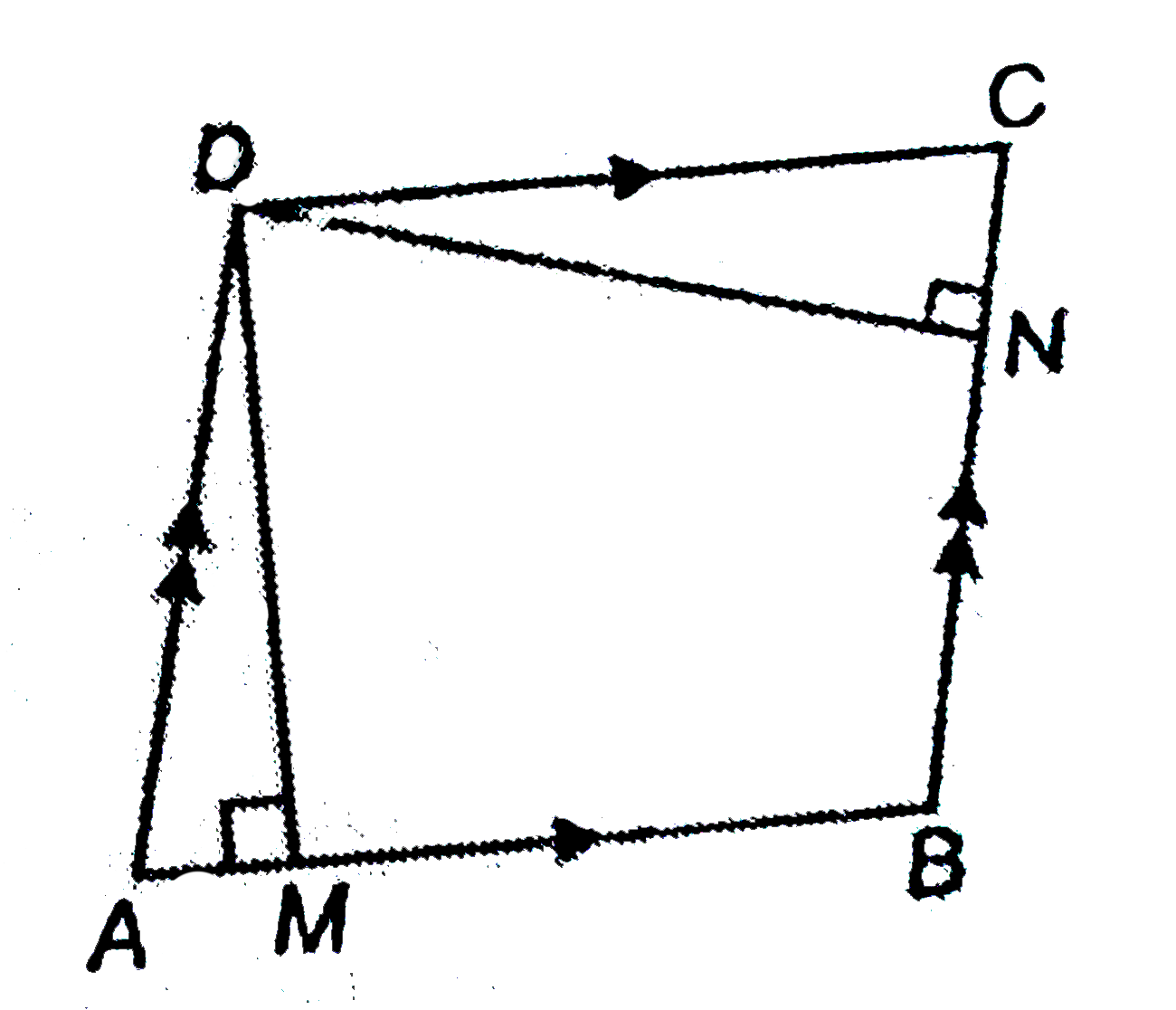
In the adjoining figure , $\angle BCD=\angle ADB$ (each ${90}^{\circ }$) . angle BC= 3 cm and length of side opposite $\angle C$ in $\Delta BCD$ is 5 cm , then find the squre root of length of side opposite to $\angle D$ in $\Delta ADB$.

Explanation

To solve the problem step by step, we will follow the given information and apply the Pythagorean theorem where necessary.

Step 1: Understand the problem
We have two triangles, ΔBCD and ΔADB, with the following information:
- Angle BCD = Angle ADB = 90 degrees
- BC = 3 cm
- The length of the side opposite angle C (BD) in ΔBCD = 5 cm
- We need to find the square root of the length of the side opposite angle D (AB) in ΔADB.

Step 2: Identify the sides in triangle BCD
In triangle BCD:
- BD is the hypotenuse = 5 cm
- BC is one of the sides = 3 cm
- We need to find CD using the Pythagorean theorem.

Step 3: Apply the Pythagorean theorem in triangle BCD
According to the Pythagorean theorem:
\( BD^2 = BC^2 + CD^2 \)
Substituting the known values:
\( 5^2 = 3^2 + CD^2 \)
\( 25 = 9 + CD^2 \)
\( CD^2 = 25 - 9 \)
\( CD^2 = 16 \)
Taking the square root:
\( CD = 4 \text{ cm} \)

Step 4: Establish relationships in triangle ADB
In triangle ADB:
- AD is the hypotenuse
- AE is the perpendicular from D to AB
- DE is the base = BC = 3 cm
- CD = BE = 4 cm (since CD = BE, as DE is parallel to BC)

Step 5: Set up the equation for triangle ADB
Using the Pythagorean theorem in triangle ADB:
\( AD^2 = AE^2 + DE^2 \)
Let AE = x cm, then:
\( AD^2 = x^2 + 3^2 \)
\( AD^2 = x^2 + 9 \) (Equation 1)

Step 6: Set up the equation for triangle ABD
In triangle ABD:
- AB is the hypotenuse
- BD = 4 cm
Using the Pythagorean theorem:
\( AB^2 = AD^2 + BD^2 \)
Substituting the known values:
\( AB^2 = AD^2 + 4^2 \)
\( AB^2 = AD^2 + 16 \) (Equation 2)

Step 7: Substitute Equation 1 into Equation 2
From Equation 1, we have:
\( AD^2 = x^2 + 9 \)
Substituting this into Equation 2:
\( AB^2 = (x^2 + 9) + 16 \)
\( AB^2 = x^2 + 25 \)

Step 8: Express AB in terms of x
We know that:
\( AB = x + 4 \)
So:
\( (x + 4)^2 = x^2 + 25 \)
Expanding the left side:
\( x^2 + 8x + 16 = x^2 + 25 \)
Subtracting \( x^2 \) from both sides:
\( 8x + 16 = 25 \)
\( 8x = 25 - 16 \)
\( 8x = 9 \)
\( x = \frac{9}{8} = 1.125 \text{ cm} \)

Step 9: Find AB
Now substituting back to find AB:
\( AB = x + 4 = 1.125 + 4 = 5.125 \text{ cm} \)

Step 10: Find the square root of AB
Now we need to find the square root of AB:
\( \sqrt{AB} = \sqrt{5.125} \)
Calculating:
\( \sqrt{5.125} \approx 2.265 \text{ cm} \)

Final Answer
The square root of the length of the side opposite to angle D in triangle ADB is approximately \( 2.265 \text{ cm} \).