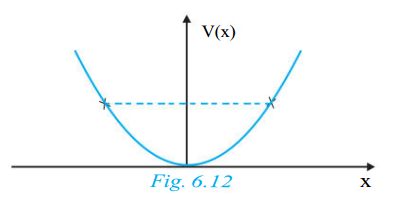
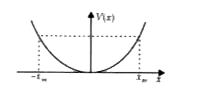
The potential energy function for a particle executing linear simple harmonic motion is given by V{x) = , wherek is the force constant of the oscillator. For , the graph of V[x) versus x is shown in Fig. 6.12. Show that a particle of total energy 1 J moving under this potential must ‘turn back’ when it reaches x =overset+- 2 m.
Solution in Punjabi
Step by step video & image solution for The potential energy function for a particle executing linear simple harmonic motion is given by V{x) = kx^2//2, wherek is the force constant of the oscillator. For k = 0.5 N m^-1, the graph of V[x) versus x is shown in Fig. 6.12. Show that a particle of total energy 1 J moving under this potential must ‘turn back’ when it reaches x =overset+- 2 m. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Fill in the blanks:- The volume of a cube side 1 cm is equal to ….m3
View SolutionThe potential energy function for a particle executing simple harmonic motion is given by V(x)=(1)/(2)kx^(2) , where k is the force constant of the oscillatore. For k=(1)/(2)Nm^(-1) , show that a particle of total energy 1 joule moving under this potential must turn back when it reaches x=+-2m.
View SolutionA particle is executing simple harmonic motion in a conservative force field. The total energy of simple harmonic motion is given by E=ax2+bv2where ‘x’ is the displacement from mean position x = 0 and v is the velocity of the particle at x then choose the INCORRECT statements.{Potential energy at mean position is assumed to be zero}
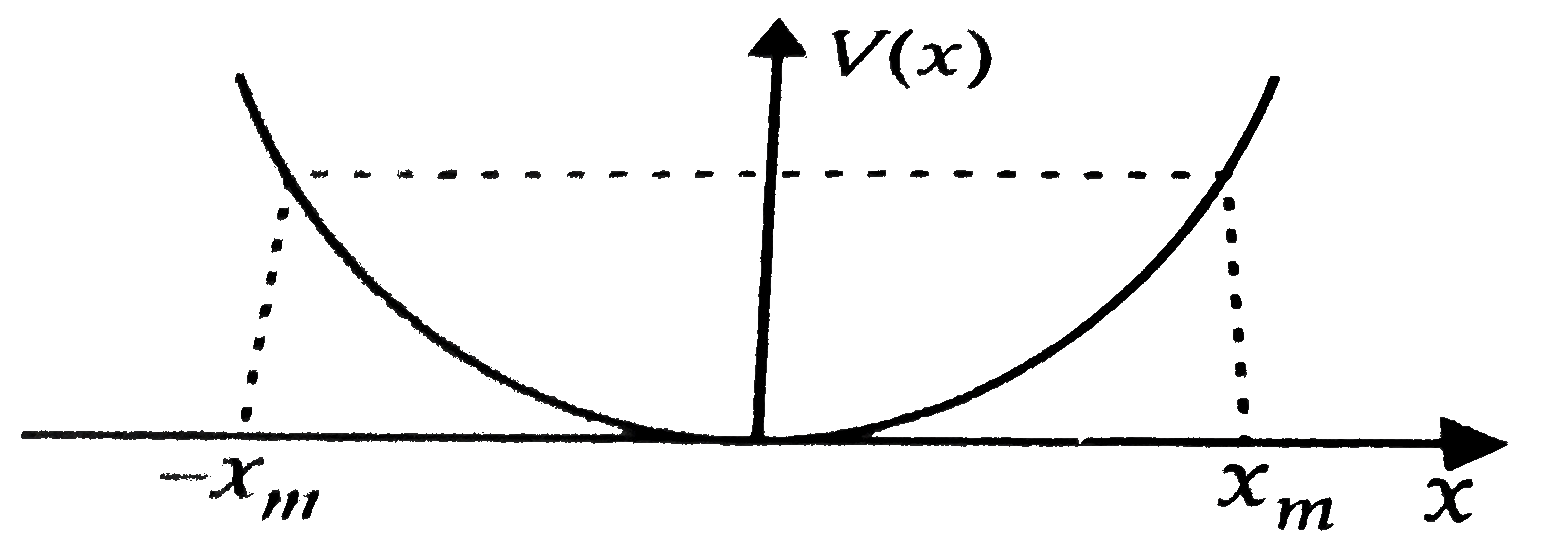
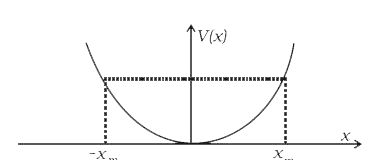
View SolutionThe potential energy function for a particle executing linear SHM is given by V(x)=12kx2 where k is the force constant of the oscillator. For k=0.5Nm−1, the graph of V(x) versus x is shown in the figure A particle of total energy E turns back when it reaches x=±xm.if V and K indicate the potential energy and kinetic energy respectively of the particle at x=+xm,then which of the following is correct?
View Solutionरेखीय सरल आवर्त गति कर रहे किसी कण का स्थितिज ऊर्जा फलन V(x)12kx2 है, जहाँ k दोलन का बल नियतांक है। k=0।5Nm−1 के लिए V (x ) व x के मध्य ग्राफ चित्र में दिखाया गया है। यह दिखाइए की इस विभव के अंतर्गत गतिमान कुल 1 J ऊर्जा wale कण को अवश्य ही वापिस आना' चाहिए , अब यह x=±2 मीटर पर पहुँचता है।
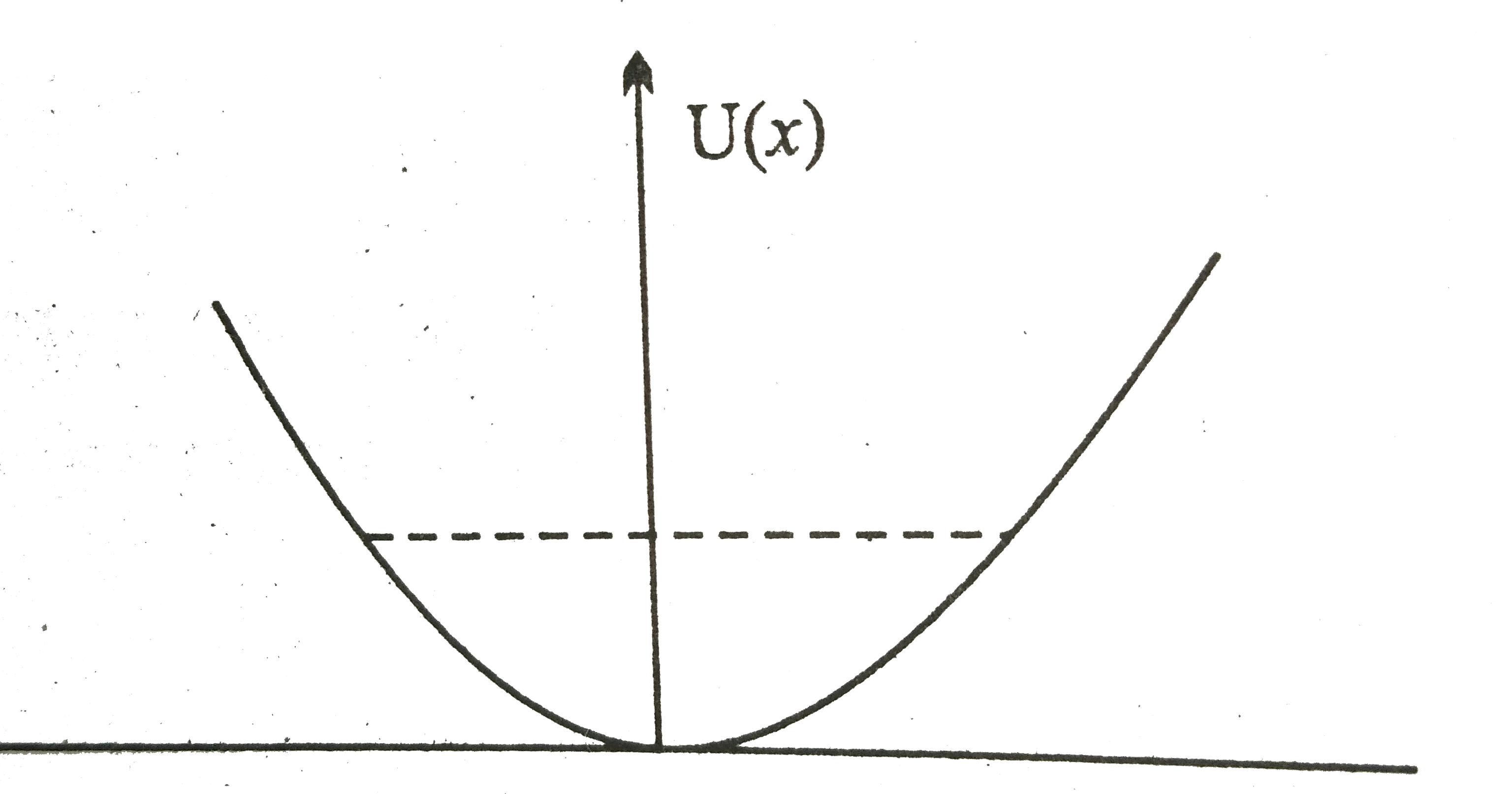
View Solutionरेखीय सरल आवर्त गति कर रहे किसी कण का स्थितिज ऊर्जा फलन U(x)=kx2/2 है, जहाँ k दोलक का बल नियतांक है । k=0.5Nm−1 के लिए U(x) व x के मध्य ग्राफ चित्र में दिखाया गया है । यह दिखाइए कि इस विभव के अंतर्गत गतिमान कुल 1J ऊर्जा वाले कण को अवश्य ही 'वापिस आना' चाहिए जब यह x=±2m पर पहुँचता है ।
View SolutionPotential energy of a particle is 12mω2x2, where m and ω are constants. Prove that the motion of the particle is simple harmonic.
View SolutionThe potential energy function for a particle executing linear simple harmonic motion is given by V(x)= kx^(2)"/"2 , where k is the force constant of the oscillator. For k= 0.5 Nm^(1) , the graph of V(x) versus x is shown in Fig. 6. 12. Show that a particle of total energy 1 J moving under this potential must 'turn back' when it reaches x= pm 2m .
View SolutionThe potential energy function for a particle executing simple harmonic motion is given by V(x)=12kx2, where k is the force constant of the oscillatore. For k=12Nm−1, show that a particle of total energy 1 joule moving under this potential must turn back when it reaches x=±2m.
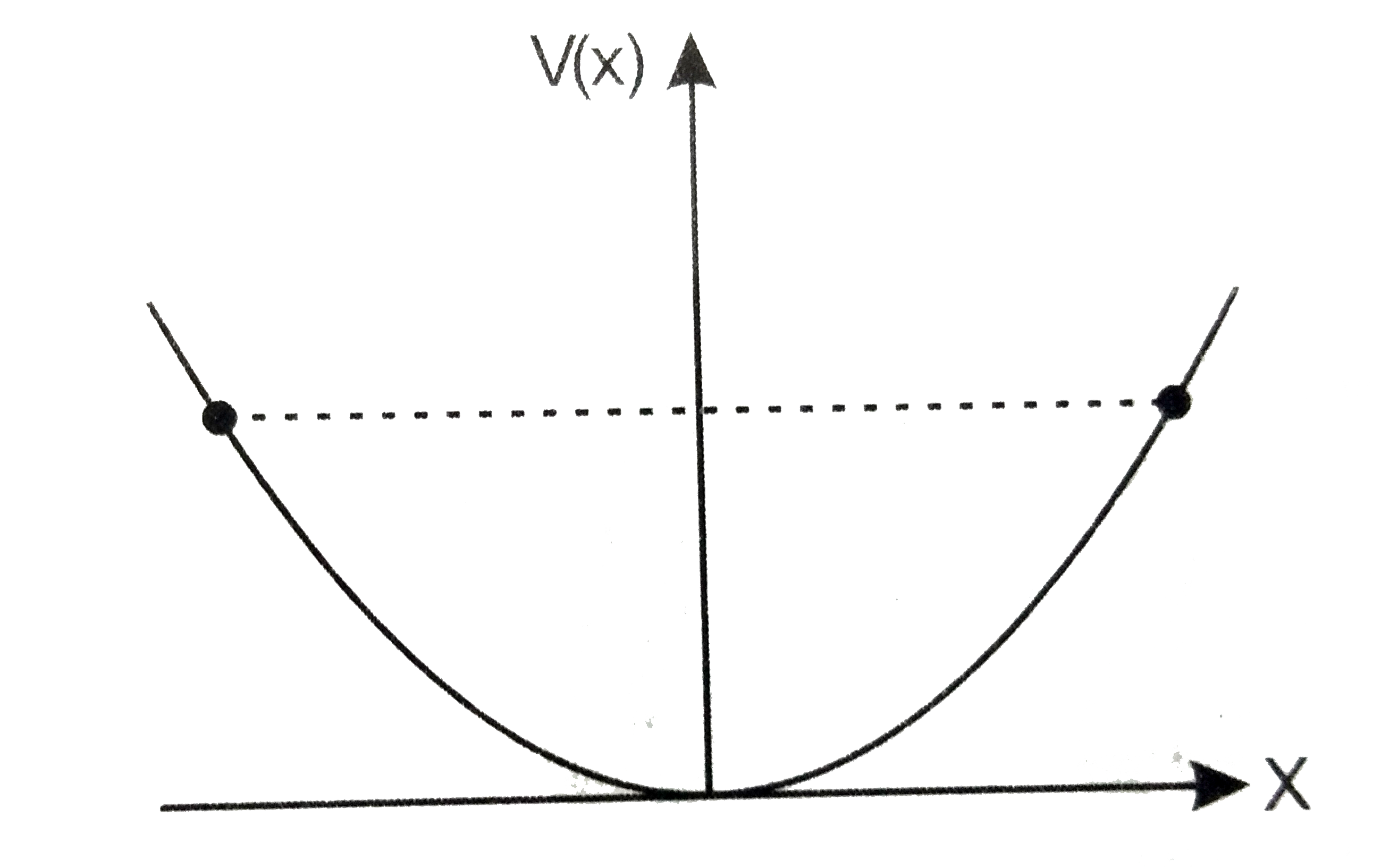
View Solutionसरल रेखीय गति कर रहे किसी कण के लिए स्थितिज ऊर्जा फलन को V(x)=12kx2 के द्वारा निरूपित किया जाता है, जहाँ दोलित्र का बल गुणांक है। k=0.5Nm−1 के लिए, V(x) बनाम x के आरेख को चित्र में दर्शाया गया है। कुल ऊर्जा E का कण उस समय वापस हो जाता है जब यह x=±xm पर पहुँचता है। यदि x=±xm पर किसी कण की स्थितिज व गतिज ऊर्जा को क्रमश: V व K के द्वारा दर्शाया जाता है, तब निम्न में से कौन-सा सही है?
View SolutionThe potential energy function for a particle executing simple harmonic motion is given by V(x)=12kx2, where k is the force constant of the oscillatore. For k=12Nm−1, show that a particle of total energy 1 joule moving under this potential must turn back when it reaches x=±2m.
View SolutionThe potential energy function for a particle executing linear simple harmonic motion is given U(x)=1/2kx^(2) , where k is the force constant of the oscillator. For k=0.5 Nm^(-1) , the graph of U(x) on Y-axis x is shown figure. The particle has total energy 1J. Moving this potential it turns back when it reaches x=+- _________m
View SolutionThe potential energy function for a particle executing linear simple harmonic motion is given by V(x)=kx22 where k is the force constant of the oscillator . For k=0.5Nm−1 , the graph of V(x) versus x is shown in figure . Show that a particle of total energy 1 J movng under this potential must 'turn back ' when it reaches x=±2m .
View SolutionThe potential energy function for a particle executing linear SHM is given by V(x)=12kx2 wher e k is the force constant of the oscillator (figure). For k = 0.5 N/m ,the graph ofV(x) versus x is shown in the figure . A particle of total energy E turns back when it reaches x=±xm . If V and K indicate the PE and KE , respectively of the particle at x=+xm , then which of the following is correct ?
View SolutionThe potential energy function for a particle executing linear SHM is given by V(x)=12kx2 where k is the force constant of the oscillator. For k=0.5Nm−1, the graph of V(x) versus x is shown in the figure A particle of total energy E turns back when it reaches x=±xm.if V and K indicate the potential energy and kinetic energy respectively of the particle at x=+xm,then which of the following is correct?
View Solutionसरल आवर्त गति (SHM) करते किसी कण का स्थैितिज ऊर्जा फलन है - V(x)=12kx2 जहाँ k दोलित्र का बल नियतांक है। k = 0.5N/m के लिए V(x) और x का ग्राफ चित्र 6.2 में दर्शाया गया है। E ऊर्जा का कोई कण x=±xm पर पहुँच कर वापस लौटता है। यदि x=+xm पर V एवं K क्रमशः कण की स्थैितिज ऊर्जा (P.E.) एवं गतिज ऊर्जा (K.E.) निरुपित करते हों तो निम्नलिखित में कौन-सा कथन सही है?
View Solution