Show that for a projectile the angle between the velocity and the x-axis as a function of time is given by
Let v0x and v0y be the initial component velocity of the projectile at O along OX direction and OY direction respectively, where OX is horizontal and oy is vertical let the projectile go from o to p in time t and vx,vy be the component velocity of projectile at P along horizontal and vertical directions respectively. Then vy=voy−gt and vx−vox
If θ is the angle which the resultant velocity →v makes with horizontal direction. Then
tanθ=vyvc=voy−gtvox or
θ=tan−1[voy−gtvox]
Similar Questions
Shown that for a projectile the angle between the velocity and the x-axis as a function of time is given by
θ(t)=tan−1(voy−>vax)
(b) Show that the projection angle θ0 for a projectile lauched from the origin is given by
θ0=(tan−1(4hm)R)
Where the symbols have their usual meaning.
View Solution(a) Show that for a projectile the angle between the velocity and the x-axis as function of time is given
by theta_(t) = tam ^(-1) ( 9 4 h_m)/R)
where the sybols have thir usual meanings.
View Solution
Knowledge Check
In case of a projectile motion, what is the angle between the velocity and acceleration at the highest point?
A0∘B45∘C90∘D180∘In case of a projectile motion, what is the angle between the velocity and acceleration at the highest point?
A0∘B45∘C90∘D180∘If θ is the angle between the velocity and acceleration of a projectile at a point of its path, its value when the projectile is at the highest point is :
A0∘B180∘C90∘D45∘
Show that for a projectile the angle between the velocity and the x-axis as function of time is given
by θt=tan−1(v0y−gtvox)
(b) Shows that the projection angle θ0 for a projectile launched from the origin is given by θt=tan−1(4hmR)
where the symbols have their usual meanings.
View SolutionFigure shows the position of a particle moving on the x - axis as a function of time
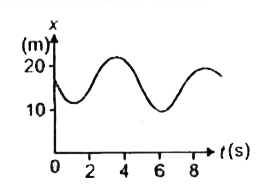
View SolutionFig. shows the graph of the x-coordinate of a particle going along the x-axis as a function of time. Find (a) the average velocity during 0 to 10s, (b) instantaneous velocity at 2, 5, 8 and 12s.
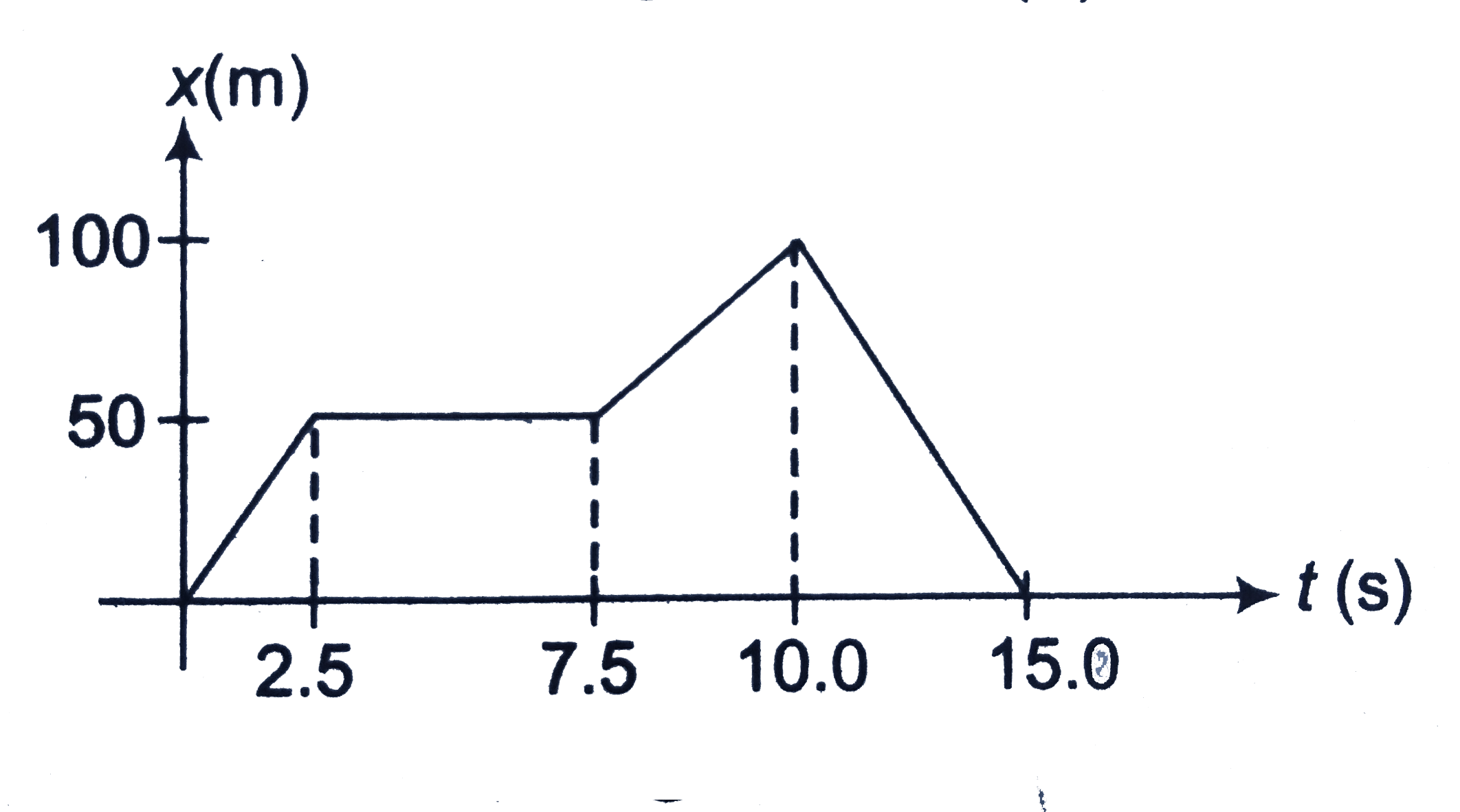
View SolutionFigure shows the position of a particle moving on the x - axis as a function of time

View SolutionAssertion : In projectile motion, the angle between the instantaneous velocity and acceleration at the highest point is 180∘.
Reason : At the highest point, velocity of projectile will be in horizontal direction only.
View Solution
