Three resistances each of 5Ω are connected to form a triangle. How much is the resistance between two ends of any arm of the triangle in this combination? (Write the answer only)
Text Solution
Consider the figure,
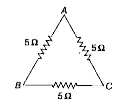
∵ Resistance RABandRAC are connected in series.
So, RS=5+5=10Ω
Also, RS is connected in parallel with RBC.
∴RP=RSRBCRS+RBC=10×510+5=103=3.33Ω
So, equivalent resistance between two ends (B and C) of triangle is 3.33Ω.
|
Similar Questions
Knowledge Check
Three resistance each of 4Ω are connected to form a triangle. The resistance between any two terminals is
A12ΩB2ΩC6ΩD83ΩThree resistances of 2Ω each are connected in a triangle. The resistance in ohms between any two comers is
A3ΩB4ΩC6ΩD43ΩThree resistances of 2Ω each are connected in a triangle. The resistance in ohms between any two comers is
A3ΩB4ΩC6ΩD43Ω
Three resistance of 12Ω are connected in the form of an equilateral triangle. The effective resistance between two corners, is
View SolutionThree resistances 4Ω each of are connected in the form of an equilateral triangle. The effective resistance between two corners is
View SolutionThree resistors, each of 2Ω are connected together in a triangular shape. The resistance offered between any two vertices will be
View SolutionSix equal resistances, each 1Ω, are joined to form a network as shown in fig. Then the resistance between any two corners is
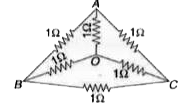
View SolutionSix equal resistances, each 1Ω, are joined to form a network as showing figure Then the resistance between any two corners is
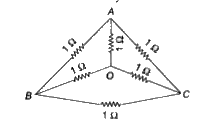
View Solution
