Three $2 Ω$ resistors are arranged in a triangle. The resistance between any two corners of the triangle is

$6 Ω$

$2 Ω$

$4 / 3 Ω$

$3 / 4 Ω$

Text Solution

Verified by Experts

(c) According to the question,

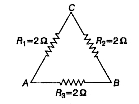
In the above circuit, between terminals A and B.
$R_{1} and R_{2}$ are connected in series combination and its equivalent resistance is parallel with $R_{3}$ .
Now, $R . = R_{1} + R_{2} = 2 + 2 = 4 Ω$
$\frac{1}{R_{eq}} = \frac{1}{R .} + \frac{1}{R_{3}} \Rightarrow \frac{1}{R_{eq}} = \frac{1}{4} + \frac{1}{2}$
$\frac{1}{R_{eq}} = \frac{1 + 2}{4} \Rightarrow \frac{1}{R_{eq}} = \frac{3}{4} \Rightarrow R_{eq} = \frac{4}{3} Ω$

A wire has a resistance of 12 ohm . It is bent in the form of equilateral triangle. The effective resistance between any two corners of the triangle is
A9 ohms
B12 ohms
C6 ohms
D $8 / 3$ ohms
Three resistances of 2 $Ω$ each are connected in a triangle. The resistance in ohms between any two comers is
A $3 Ω$
B $4 Ω$
C $6 Ω$
D $\frac{4}{3} Ω$
Three resistances of 2 $Ω$ each are connected in a triangle. The resistance in ohms between any two comers is
A $3 Ω$
B $4 Ω$
C $6 Ω$
D $\frac{4}{3} Ω$