A uniform metallic wire has a resistance of 18 Ω and is bent into an equiolateral triangle. Then, the resistance between any two vertices of the triangle is :
4 Ω
8 Ω
12 Ω
2 Ω
To solve the problem of finding the resistance between any two vertices of an equilateral triangle formed by bending a uniform metallic wire with a total resistance of 18 Ω, we can follow these steps:
1. Determine the Resistance of Each Side of the Triangle:
The total resistance of the wire is 18 Ω. When the wire is bent into an equilateral triangle, it forms three equal sides. Therefore, the resistance of each side (R_side) can be calculated as:
Rside=Rtotal3=18Ω3=6Ω
2. Identify the Configuration of the Resistances:
Let’s label the vertices of the triangle as A, B, and C. The resistances between the vertices are:
- R_AB (between A and B)
- R_BC (between B and C)
- R_CA (between C and A)
Each of these resistances is equal to 6 Ω.
3. Analyze the Resistance Between Two Vertices (e.g., A and B):
To find the equivalent resistance between vertices A and B (R_AB), we note that the resistances R_AC and R_BC are in parallel with respect to the path between A and B.
4. Calculate the Equivalent Resistance of R_AC and R_BC:
The two resistances R_AC and R_BC are in parallel. The formula for equivalent resistance (R_parallel) for two resistors in parallel is given by:
1Rparallel=1RAC+1RBC
Substituting the values:
1Rparallel=16+16=26=13
Therefore, the equivalent resistance R_parallel is:
Rparallel=3Ω
5. Add the Series Resistance:
The equivalent resistance R_AB is the sum of the parallel resistance R_parallel and the resistance of the side AB (which is also 6 Ω):
RAB=Rparallel+RAB=3Ω+6Ω=9Ω
6. Final Calculation:
Thus, the equivalent resistance between any two vertices of the triangle is:
RAB=4Ω
Final Answer:
The resistance between any two vertices of the triangle is 4 Ω.
The total resistance of the wire is 18 Ω. When the wire is bent into an equilateral triangle, it forms three equal sides. Therefore, the resistance of each side (R_side) can be calculated as:
Rside=Rtotal3=18Ω3=6Ω
Let’s label the vertices of the triangle as A, B, and C. The resistances between the vertices are:
- R_AB (between A and B)
- R_BC (between B and C)
- R_CA (between C and A)
To find the equivalent resistance between vertices A and B (R_AB), we note that the resistances R_AC and R_BC are in parallel with respect to the path between A and B.
The two resistances R_AC and R_BC are in parallel. The formula for equivalent resistance (R_parallel) for two resistors in parallel is given by:
1Rparallel=1RAC+1RBC
Substituting the values:
1Rparallel=16+16=26=13
Therefore, the equivalent resistance R_parallel is:
Rparallel=3Ω
The equivalent resistance R_AB is the sum of the parallel resistance R_parallel and the resistance of the side AB (which is also 6 Ω):
RAB=Rparallel+RAB=3Ω+6Ω=9Ω
Thus, the equivalent resistance between any two vertices of the triangle is:
RAB=4Ω
The resistance between any two vertices of the triangle is 4 Ω.
Topper's Solved these Questions
Similar Questions
A wire has a resistance of 12 ohm . It is bent in the form of equilateral triangle. The effective resistance between any two corners of the triangle is
View SolutionA wire has resistance of 24Ω is bent in the following shape. The effective resistance between A and B is
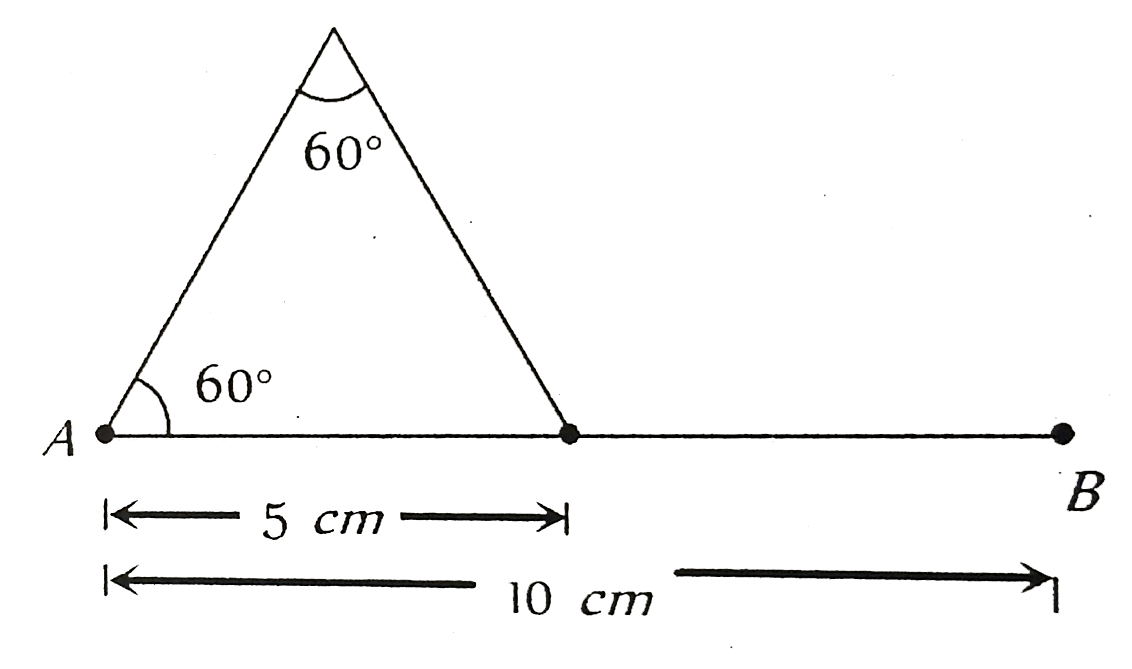
View SolutionThree resistance each of 4Ω are connected to form a triangle. The resistance between any two terminals is
View SolutionA metallic wire having a resistance of 20Ω is bent in order to form a complete circle. Calculate the resistance between any two diametrically opposite points on the circle.
View SolutionA wire has resistance 12Ω. It is bent in the form of a circle. The effective resistance between two points across a diameter is.
View SolutionThree resistors each of 2 ohm are connected together in a triangular shape. The resistance between any two vertices will be
View SolutionThe resistance of each side of an equilateral triangle is 6 ohm. Calculate the equivalent resistance between ends of a side.
View SolutionA metal wire has a resistance of 35Ω. If its length is increased to double by drawing it, then its new resistance will be
View SolutionA wire has a resistance of 10Ω. It is stretched by 1/10 of its original length. Then its resistance will be
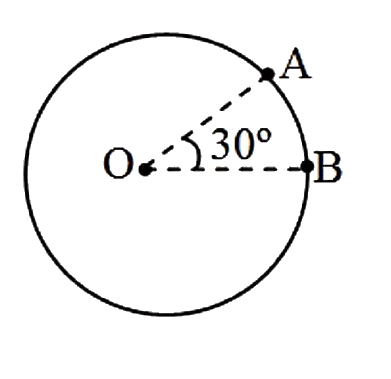
View SolutionA uniform wire of resistance 36Ω is bent in the form of a circle. The Effective resistance between A and B is (O is the centre of circle):
View Solution
