Given a rectangle ABCD, determine two points K and L on the sides BC and CD such that the triangles ABK, AKL and ADL have same area.
The correct Answer is:K is (√5−12)BC units from B and L is (√5−12)DA units from D
Topper's Solved these Questions
- BOOK - RESONANCECHAPTER - GEOMETRYEXERCISE - Exercise-1 (Part-I: Previous Asked Question For Pre RMO)50videos
GEOMETRY
- BOOK - RESONANCECHAPTER - FUNDAMENTAL OF MATHEMATICSEXERCISE - Exercise138videos
FUNDAMENTAL OF MATHEMATICS
- BOOK - RESONANCECHAPTER - INDEFINITE INTEGRATION EXERCISE - SELF PRACTIC PROBLEMS25videos
INDEFINITE INTEGRATION
Similar Questions
Knowledge Check
- Question 1 - Select One
If P and Q are the middle points of the sides BC and CD of the parallelogram ABCD, then AP+AQ is equal to
AACB12ACC23ACD32AC - Question 2 - Select One
ABCD is a rectangle of perimeter 48 cm with AB = 6 cm and CD = DE .What is the area of the triangle ACE ?
 A18cm2B24cm2C30cm2D36cm2
A18cm2B24cm2C30cm2D36cm2 - Question 3 - Select One
In the figure, ABCD is a rectangle. Points E and F cut the sides BC and CD of the rectangle respectively such that EC = 3, FC = 4, and AD = 12, and the area of the crossed and the shaded regions in the figure are equal. Which one of the following equals the perimeter of rectangle ABCD?
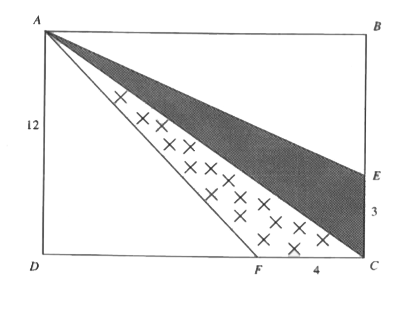 A42B50C56D64
A42B50C56D64
The point H orthocentre of the triangle ABC. A point K is taken on the straight line CH such that ABK is a right triangle. Prove that the area of the triangle ABK is the geometric mean between the area of the triangles ABC and ABH.
View SolutionABCD is a rectangle and P, Q, R and S are mid-points of the sides AB, BC, CD and DA respectively. Show that the quadrilateral PQRS is a rhombus.
View SolutionABCD is a rectangle and P, Q, R and S are mid-points of the sides AB, BC, CD and DA respectively. Show that the quadrilateral PQRS is a rhombus.
View SolutionGiven a parallelogram ABCD where X and Y are the mid-points of the sides BC and CD respectively . Prove that:
ar (ΔAXY)=38×ar(/gm)ABCDView SolutionIn the given figure, ABCD is a rectangle in AB−40cmandBC=25cm. If P,Q,R,S be the mid-points of the sides AB,BC,CDandDA respectively, find the area of the shaded region.
View Solution

