If P and Q are the middle points of the sides BC and CD of the parallelogram ABCD, then AP+AQ is equal to
AC
12AC
23AC
32AC
The correct Answer is:D
To solve the problem, we need to find the sum of the vectors →AP+→AQ where P and Q are the midpoints of sides BC and CD of the parallelogram ABCD.
1. Identify Points and Vectors:
Let the position vectors of points A,B,C,D be represented as →A,→B,→C,→D respectively. Since ABCD is a parallelogram, we have:
→C=→A+→B−→D
2. Find Midpoints P and Q:
The midpoint P of side BC can be expressed as:
→P=→B+→C2
The midpoint Q of side CD can be expressed as:
→Q=→C+→D2
3. Express →AP and →AQ:
The vector →AP is given by:
→AP=→P−→A=(→B+→C2)−→A
The vector →AQ is given by:
→AQ=→Q−→A=(→C+→D2)−→A
4. Substituting →C:
Substitute →C=→A+→B−→D into the equations for →AP and →AQ:
→AP=→B+(→A+→B−→D)2−→A=2→B+→A−→D2−→A=2→B−→A−→D2
→AQ=(→A+→B−→D)+→D2−→A=→A+→B2−→A=→B−→A2
5. Adding →AP and →AQ:
Now, we can add →AP and →AQ:
→AP+→AQ=(2→B−→A−→D2)+(→B−→A2)
Combine the terms:
=(2→B−→A−→D)+(→B−→A)2=3→B−2→A−→D2
6. Expressing in terms of →AC:
Since →AC=→C−→A:
→AC=→A+→B−→D−→A=→B−→D
Therefore, we can express →AP+→AQ in terms of →AC:
→AP+→AQ=32→AC
Conclusion:
Thus, we find that:
→AP+→AQ=32→AC
Let the position vectors of points A,B,C,D be represented as →A,→B,→C,→D respectively. Since ABCD is a parallelogram, we have:
→C=→A+→B−→D
The midpoint P of side BC can be expressed as:
→P=→B+→C2
The midpoint Q of side CD can be expressed as:
→Q=→C+→D2
The vector →AP is given by:
→AP=→P−→A=(→B+→C2)−→A
The vector →AQ is given by:
→AQ=→Q−→A=(→C+→D2)−→A
Substitute →C=→A+→B−→D into the equations for →AP and →AQ:
→AP=→B+(→A+→B−→D)2−→A=2→B+→A−→D2−→A=2→B−→A−→D2
→AQ=(→A+→B−→D)+→D2−→A=→A+→B2−→A=→B−→A2
Now, we can add →AP and →AQ:
→AP+→AQ=(2→B−→A−→D2)+(→B−→A2)
Combine the terms:
=(2→B−→A−→D)+(→B−→A)2=3→B−2→A−→D2
Since →AC=→C−→A:
→AC=→A+→B−→D−→A=→B−→D
Therefore, we can express →AP+→AQ in terms of →AC:
→AP+→AQ=32→AC
Thus, we find that:
→AP+→AQ=32→AC
Topper's Solved these Questions
- VECTOR ALGEBRABook:ARIHANT MATHS ENGLISHChapter:VECTOR ALGEBRAExercise:Exercise (More Than One Correct Option Type Questions)
Explore 7 Videos
- VECTOR ALGEBRABook:ARIHANT MATHS ENGLISHChapter:VECTOR ALGEBRAExercise:Exercise (Statement I And Ii Type Questions)
Explore 3 Videos
- VECTOR ALGEBRABook:ARIHANT MATHS ENGLISHChapter:VECTOR ALGEBRAExercise:VECTOR ALGEBRA EXERCISES 1: Single Option Correct Type Questions
Explore 1 Video
- TRIGONOMETRIC FUNCTIONS AND IDENTITIESBook:ARIHANT MATHS ENGLISHChapter:TRIGONOMETRIC FUNCTIONS AND IDENTITIESExercise:Exercise (Questions Asked In Previous 13 Years Exam)
Explore 19 Videos
Similar Questions
If P and Q are the mid points of the sides AB and CD of a parallelogram ABCD, prove that DP and BQ cut the trisection which also the points of trisection of DP and BQ respectively.
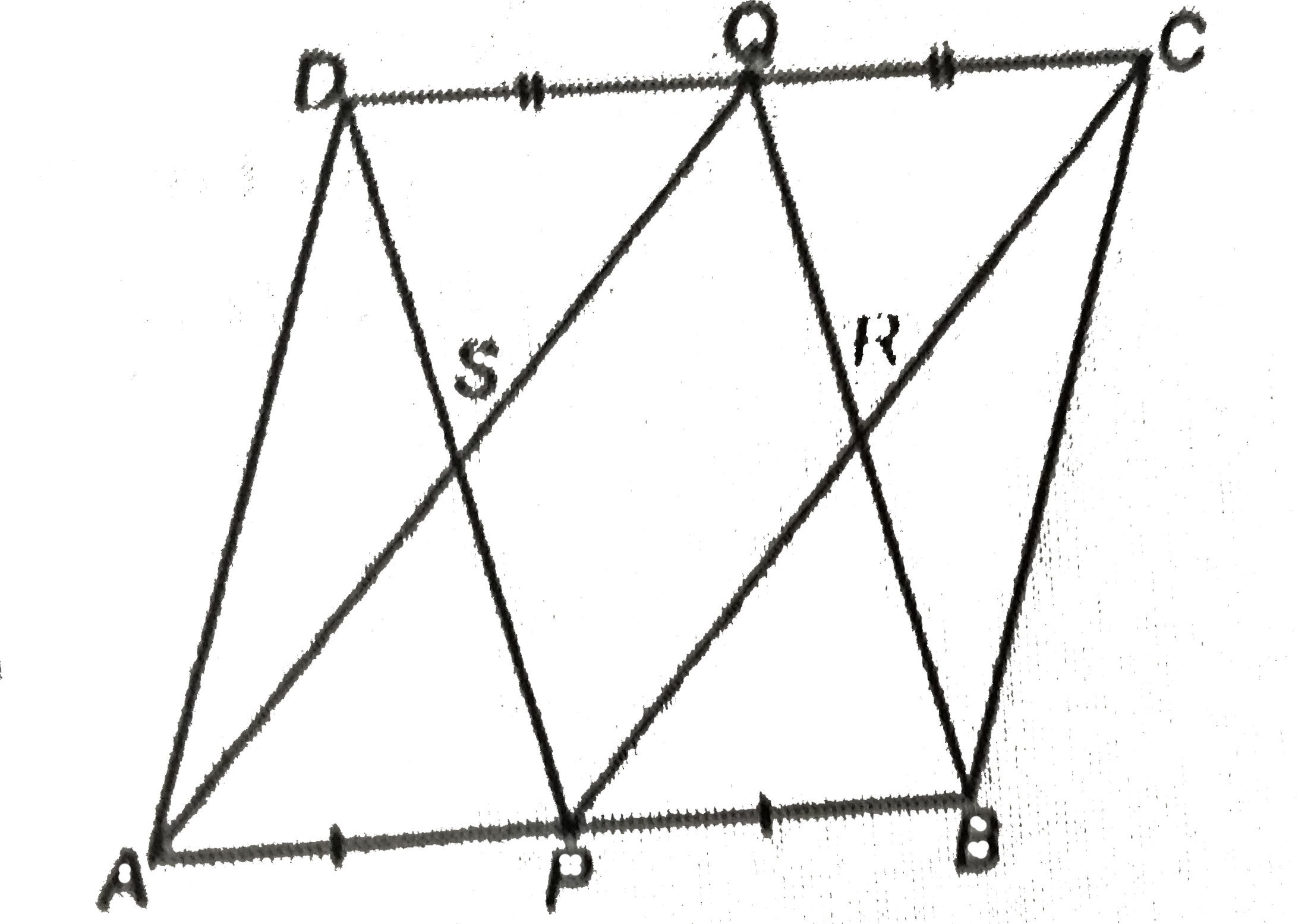
View SolutionP and Q are the mid-point of the opposite sides AB and CD of a parallelogram ABCD. AQ intersects DP at S and BQ intersects CP at R. Show that PQRS is a parallelogram.
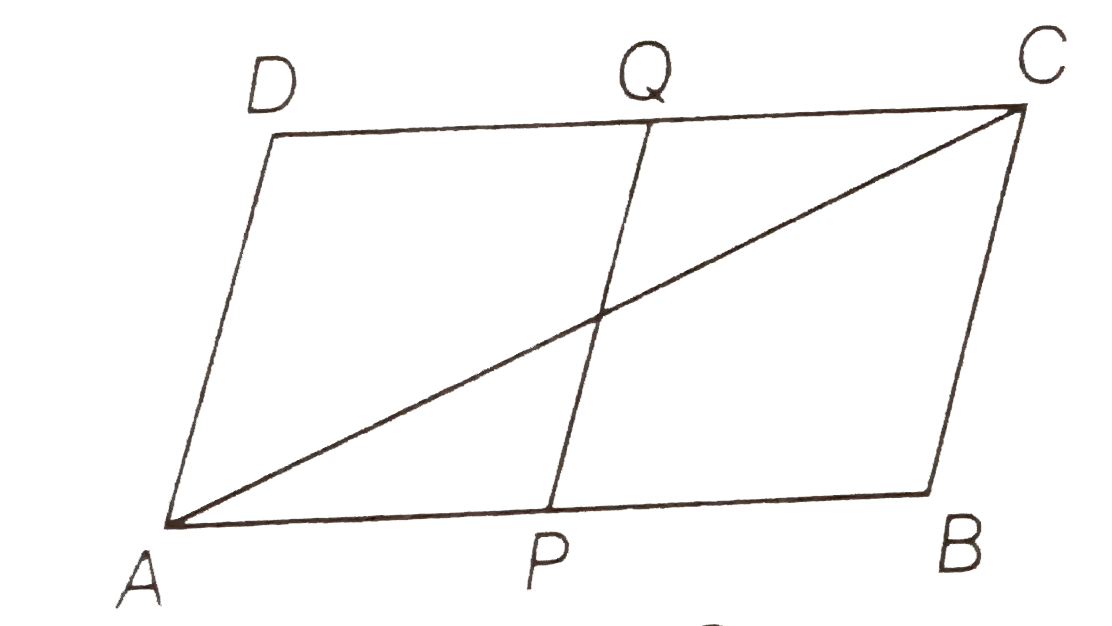
View SolutionIn the diagram below, P and Q are midpoints of sides BC and AD respectively of the parallelogram ABCD. If side AB = diagonal BD: prove that the quadrilateral BPDQ is a rectangle.

View SolutionP and Q are points on opposite sides AD and BC of a parallelogram ABCD such that PQ passes through the point of intersection O of its diagonals AC and BD. Show that PQ is bisected at O.
View SolutionE, F, G and H are the mid-points of the sides of a parallelogram ABCD. Show that area of quadrilateral EFGH is half of the area of parallelogram ABCD.
View SolutionP and Q are the points of trisection of the diagonal BD of a parallelogram ABCD. Prove that CQ is parallel to AP.
View SolutionP and Q are any two points lying on the sides DC and AD respectively of a parallelogram ABCD. Show that ar (APB) = ar (BQC).
View SolutionP, Q , R and S are respectively the mid-points of the sides AB, BC, CD and DA of a quadrilateral ABCD in which AC = BD. Prove that PQRS is a rhombus.
View SolutionP,Q,R and S are the mid points of sides AB,BC , CD and DA respectively of rhombus ABCD. Show that PQRS is a rectangle.
Under what conditions will PQRS be a square ?
View SolutionPoints P and Q have been taken on opposite sides AB and CD, respectively of a parallelogram ABCD such that AP=CQ. Show that AC and PQ bisect each other.
View Solution
