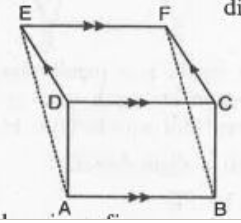
ABCD ও ABEF দুটি সামান্তরিক হলে, প্রমাণ করো, CDEF একটি সামান্তরিক।
Step by step video & image solution for ABCD ও ABEF দুটি সামান্তরিক হলে, প্রমাণ করো, CDEF একটি সামান্তরিক। by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
ক্রয়মূল্য ও বিক্রয়মূল্যের অনুপাত 10:11 হলে, শতকরা লাভ -
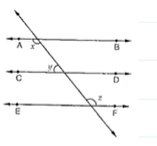
View SolutionIn Figure, If ABCD, CDEF and y:z=3:7, find x
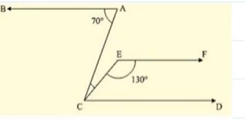
View SolutionIn Fig. If ABCD and CD EF, Find ∠ACE
View Solution2Ag(s)+Fe^(2+)(aq)rarr2Ag^+(aq)+Fe(s) বিক্রিয়াটি কী সম্ভব? ব্যাখ্যা করো। দেওয়া আছে, Fe^(2+)|Fe ও Ag^+|Ag তড়িদ্দ্বারের প্রমাণ বিজারণ বিভবের মান যথাক্রমে -0.44 ও +0.80V।
View SolutionABCD ఒక సమాంతర చతుర్భుజము ABEF ఒక దీర్ఘచతురస్రము DG, AB పైకి గీచిన లంబము అయిన (ABCD)వై||= (ABEF) వై|| అని చూపండి.
View Solutionযদি |z1|=|z2|এবংarg z1 + arg z2 = 0হয়,তবেপ্রমাণকরোz1 = 1/(z2)।
View Solutionযদি |z1|=|z2|এবংarg z1 ~ arg z2 = piহয়,তবেপ্রমাণকরোz1 + z2 = 0।
View Solutionমনে করো xy সমতলে অবস্থিত S একটি বৃত্ত, যা x2+y2 = 4 সমীকরণ দ্বারা সংজ্ঞাত। মনে করো S বৃত্তের ওপর P এমন একটি বিন্দু যার স্থানাঙ্ক দুটি (x স্থানাঙ্ক ও yস্থানাঙ্ক) ধনাত্মক। মনে করো P বিন্দুতে S বৃত্তের স্পর্শক অক্ষদ্বয়কে M ও N বিন্দুতে ছেদ করেছে। তবে MN সরলরেখার মধ্যবিন্দু যে বক্রের ওপর অবস্থিত, তা হল—
View Solutionα+β=π3[α>0,β>0]'হলেtan alpha tan beta -র বৃহত্তম মান নির্ণয় করো|
View Solution|→α|=4, abs(vecbeta) =3এবংabs(vecalphaxxvecbeta) =6 হলে, vecalpha ও vecbeta ভেক্টর দুটির অন্তর্গত কোণ হবে—
View SolutionABCD is parallelogram and ABEF is a rectangle and DG is perpendicular on AB.
Prove that (i) ar (ABCD) = ar(ABEF)
(ii) ar (ABCD) = AB×DG
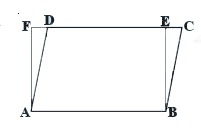
View SolutionIn the adjacent figure ABCD is a parallelogram ABEF is a rectangle show that ΔAFD≅ΔBEC.
View SolutionABCD is parallelogram and ABEF is a rectangle and DG is perpendicular on AB.
Prove that (i) ar (ABCD) = ar(ABEF)
(ii) ar (ABCD) = AB×DG
View SolutionABCD is parallelogram and ABEF is a rectangle and DG is perpendicular on AB.
Prove that (i) ar (ABCD) = ar(ABEF)
(ii) ar (ABCD) = AB×DG
View SolutionABCD is parallelogram and ABEF is a rectangle and DG is perpendicular on AB.
Prove that (i) ar (ABCD) = ar(ABEF)
(ii) ar (ABCD) = AB×DG
View SolutionIn the given figure
 ABCD is a rectangle and a parallelogram CDEF drawn on opposite side of CD. If ar(∣∣gmABFE)=60cm2 and ar(∣∣gmCDEF)=32cm2, find ar(rect ABCD).
ABCD is a rectangle and a parallelogram CDEF drawn on opposite side of CD. If ar(∣∣gmABFE)=60cm2 and ar(∣∣gmCDEF)=32cm2, find ar(rect ABCD).
View Solution
