ABCD is parallelogram and ABEF is a rectangle and DG is perpendicular on AB.
Prove that (i) ar (ABCD) = ar(ABEF)
(ii) ar (ABCD) = AB×DG Solution in Gujrati
Step by step video & image solution for ABCD is parallelogram and ABEF is a rectangle and DG is perpendicular on AB. Prove that (i) ar (ABCD) = ar(ABEF) (ii) ar (ABCD) = AB xx DG by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
If 1cm represents 5m, what would be an area of 6 square cm. represent ?
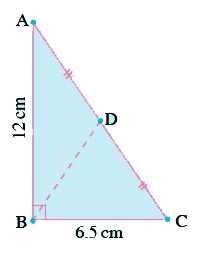
View SolutionIn ΔABC,∠ABC=90∘,AD=DC,AB=12cmandBC=6.5 cm. Find the area of ΔADB .
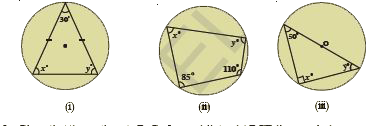
View SolutionFind the values of x and y in the figures given below
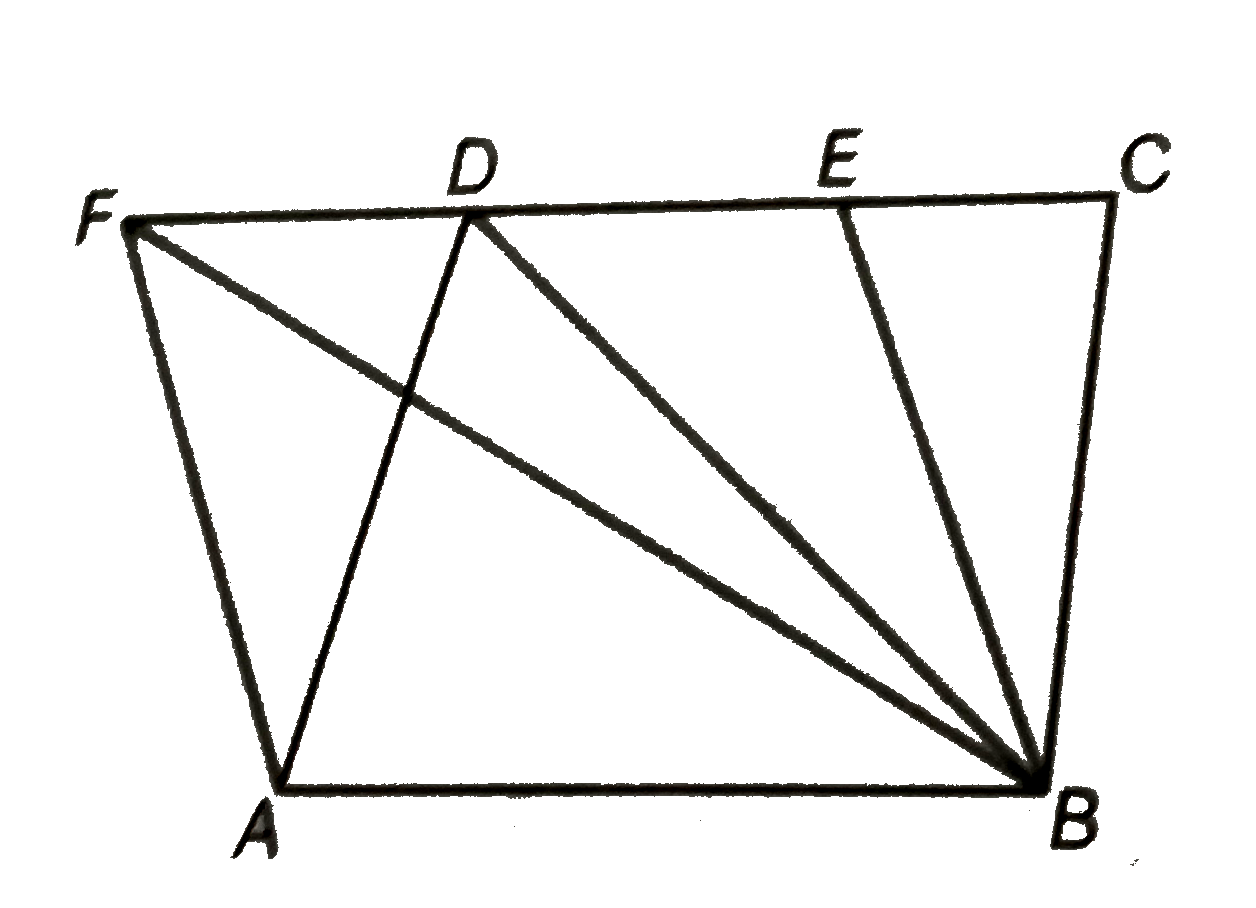
View SolutionThe area of the parallelogram ABCD is 90CM2. Find
(i) ar (ABEF) (ii) ar (ΔABD)
(iii) ar (ΔBEF)
View Solutionआक्रति 9.13 में , ABCD एक समांतर चतुर्भुज है और EFCD एक आयत है ।
साथ ही , AL⊥DC है सिद्ध कीजिए कि
(i) ar(ABCD)=ar(EFCD)
(ii)(ABCD)=DC×AL
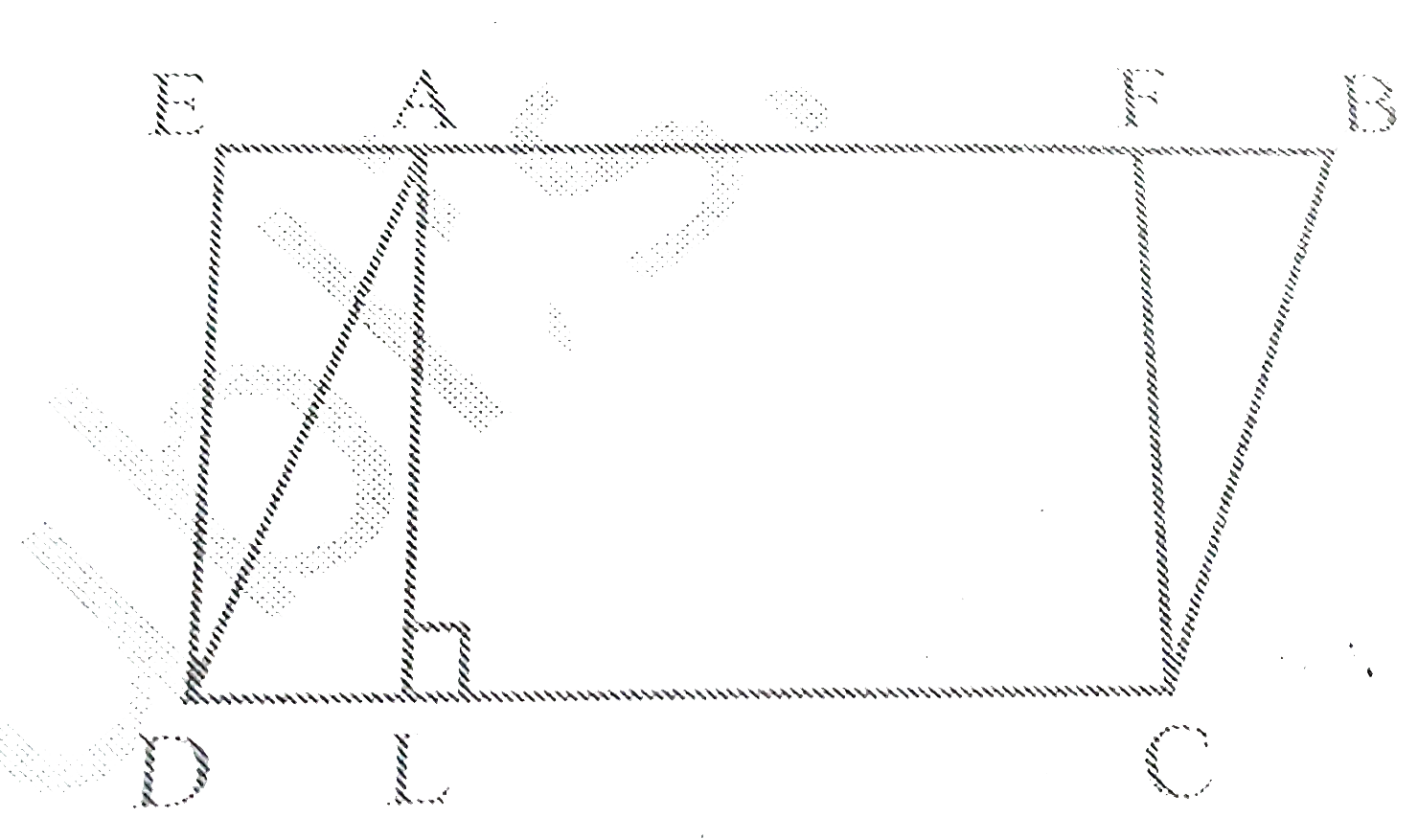
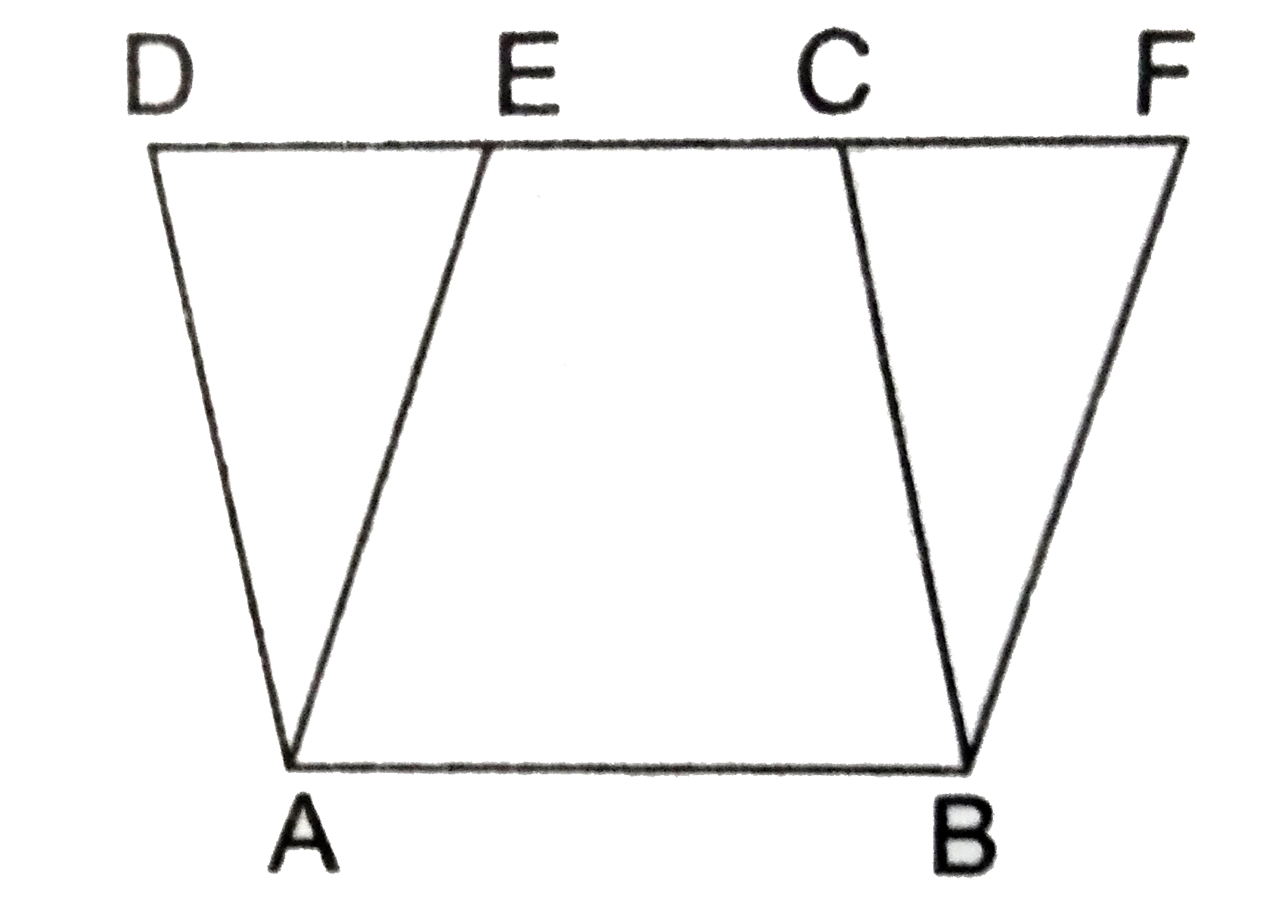
View SolutionIn the given figure, ABCD and ABEF are parallelograms such that ar(quad. EABC) 17cm2 and ar(||gm ABCD) =25cm2. Then ar(△BCF) = ?
View Solutionचित्र में , ABCD एक समान्तर चतुर्भुज है तथा EFCD एक आयत है। साथ ही AL⊥DC, तो सिद्ध कीजिए कि
(i)ar(ABCD)=ar(EFCD)
(ii)ar(ABCD)=DC×AL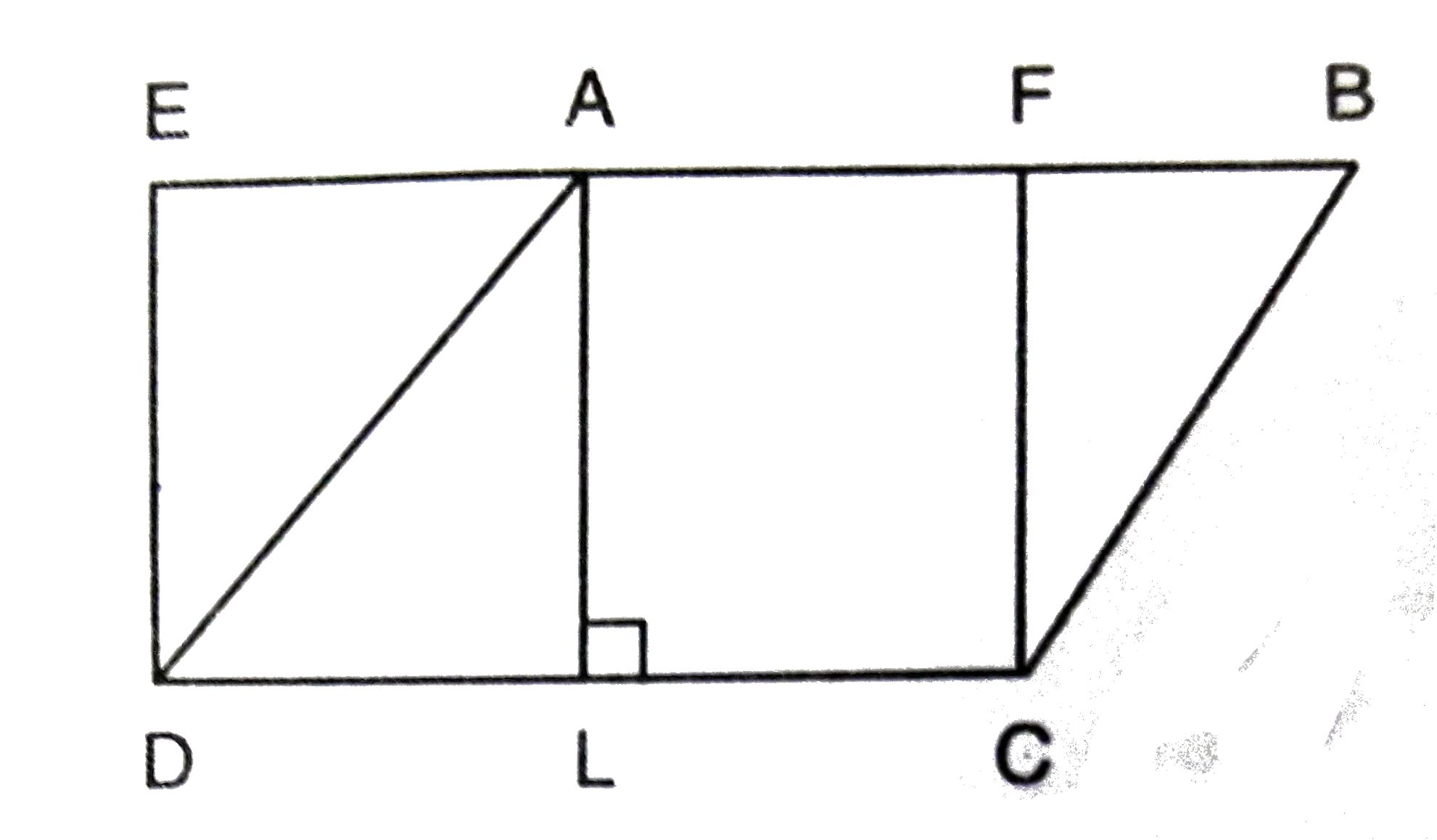
View SolutionABCD ఒక సమాంతర చతుర్భుజము ABEF ఒక దీర్ఘచతురస్రము DG, AB పైకి గీచిన లంబము అయిన (ABCD)వై||= (ABEF) వై|| అని చూపండి.
View SolutionABCD ఒక సమాంతర చతుర్భుజము ABEF ఒక దీర్ఘచతురస్రము DG, AB పైకి గీచిన లంబము అయిన (ABCD)వై||=ABxDG వై|| అని చూపండి.
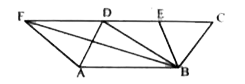
View Solutionसमान्तर चतुर्भुज ABCD का क्षेत्रफल 90cm2 है। (आकृति देखिए) तो निम्नलिखित का क्षेत्रफल ज्ञात कीजिए।
(i)ar(ABEF) , (ii)ar(ABD) , (iii) ar(BEF)
View SolutionABCD is parallelogram and ABEF is a rectangle and DG is perpendicular on AB.
Prove that (i) ar (ABCD) = ar(ABEF)
(ii) ar (ABCD) = AB×DG
View SolutionTriangle ABC and parallelogram ABEF are on the same base, AB as in between the same parallels AB and EF. Prove that ar (ΔABC)=12 ar(|| gm ABEF)
View SolutionABCD is parallelogram and ABEF is a rectangle and DG is perpendicular on AB.
Prove that (i) ar (ABCD) = ar(ABEF)
(ii) ar (ABCD) = AB×DG
View SolutionTriangle ABC and parallelogram ABEF are on the same base, AB as in between the same parallels AB and EF. Prove that ar (ΔABC)=12 ar(|| gm ABEF)
View SolutionTriangle ABC and parallelogram ABEF are on the same base, AB as in between the same parallels AB and EF. Prove that ar (ΔABC)=12 ar(|| gm ABEF)
View Solution
