A real inverted image in a concave mirror is represented by (,, are corrdinates)
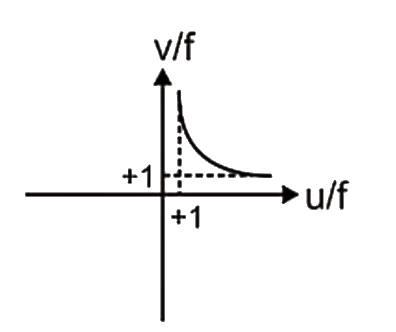
The correct Answer is:A
To solve the problem of identifying the correct graph representing the relationship between the coordinates of a real inverted image in a concave mirror, we can follow these steps:
1. Understand the Mirror Formula:
The mirror formula for a concave mirror is given by:
1f=1u+1v
where f is the focal length, u is the object distance (negative for real objects in concave mirrors), and v is the image distance (negative for real images).
2. Rearranging the Formula:
We can rearrange the mirror formula to express it in a different form:
1v=1f−1u
Multiplying through by f gives:
fv+fu=1
This can be rewritten as:
1u+1v=1f
3. Identifying Variables:
Let's set:
- x=1u
- y=1v
Thus, the equation becomes:
x+y=1f
4. Graph Interpretation:
The equation x+y=1f represents a straight line in the xy-plane. However, since we are dealing with a hyperbolic relationship due to the nature of the mirror, we need to consider the behavior of x and y as they approach their limits.
5. Behavior of the Graph:
- If x (which corresponds to 1u) approaches 0 (i.e., u approaches infinity), then y (which corresponds to 1v) approaches 1f.
- Conversely, if y approaches 0 (i.e., v approaches infinity), then x approaches 1f.
6. Conclusion on Graph:
The resulting graph will be a hyperbola that opens towards the positive axes, indicating the relationship between u and v for real inverted images in a concave mirror.
7. Selecting the Correct Graph:
After analyzing the options provided, the correct graph that represents this relationship is the one that depicts a hyperbola in the first quadrant, confirming that both u and v are negative for real images.
The mirror formula for a concave mirror is given by:
1f=1u+1v
where f is the focal length, u is the object distance (negative for real objects in concave mirrors), and v is the image distance (negative for real images).
We can rearrange the mirror formula to express it in a different form:
1v=1f−1u
Multiplying through by f gives:
fv+fu=1
This can be rewritten as:
1u+1v=1f
Let's set:
- x=1u
- y=1v
Thus, the equation becomes:
x+y=1f
The equation x+y=1f represents a straight line in the xy-plane. However, since we are dealing with a hyperbolic relationship due to the nature of the mirror, we need to consider the behavior of x and y as they approach their limits.
- If x (which corresponds to 1u) approaches 0 (i.e., u approaches infinity), then y (which corresponds to 1v) approaches 1f.
- Conversely, if y approaches 0 (i.e., v approaches infinity), then x approaches 1f.
The resulting graph will be a hyperbola that opens towards the positive axes, indicating the relationship between u and v for real inverted images in a concave mirror.
After analyzing the options provided, the correct graph that represents this relationship is the one that depicts a hyperbola in the first quadrant, confirming that both u and v are negative for real images.
Similar Questions
The image formed by a concave mirror
View SolutionThe image formed by a plane mirror is real. True/False
View SolutionThe minimum sum of the distances of a real object and a real image from a concave mirror of radius of curvature 20cm is:
View SolutionAs the position of an object (u) reflected from a concave mirror is varied, the position of the image (v) also varies. By latting the u changes from 0 to +∞ the graph between v versus u will be
View SolutionThe distance between an object and its doubly magnified image by a concave mirror is: [Assume f= focal length]
View SolutionA plane mirror is placed at a distance of 50 cm from a concave mirror of focal length 16 cm. Where should a short object be placed between the mirrors and facing both the mirrors so that its virtual image in the plane mirror coincides with the real image in concave mirror? What is the ratio of the sizes of the two images?
View SolutionState the following statements as TRUE or FALSE.
a. A convex mirror cannot from a real image for a real object.
b. The image formed by a convex mirror is always diminished and erect.
c. Virtual image formed by a concave mirror is always enlarged.
d. Only in the case of a concave mirror, it may happen that the object and its image move in same direction.
e. In the case of a concave mirror, the image always move faster than the object.
f. If an object is placed in front of a diverging mirror at a distance equal to its focal length, then the height of image formed is half of the height of object.
g. For two positions of an object , a concave mirror can form englarged image.
h. Concave mirror is used as a rear view mirror in motor vehicles.
i. If some portion of the mirror ois covered, then complete image will be formed but of reduced brightness.
j. A plane mirror always forms an erect iamge of same size as that of the object.
k. The image formed by a plane mirror has left-right reversal.
l. A virtual object means a converging beam.
View SolutionIf the distance between the virtual image from Its real object is 60 cm in case of a concave mirror . The focal length of the concave mirror If the image formed is 3 times magnified image is
View SolutionAn object is placed at a distance u from a concave mirror and its real image is received on a screen placed at a distance of v from the mirror. If f is the focal length of the mirror, then the graph between 1/v versus 1/u is
View SolutionThe erect image formed by a concave mirror is of size double the size of object. How are u and v related?
View Solution
