An object will continue moving uniformly until
the resultant force acting on it begins to decrease
the resultant force on it is zero
the resultant force is at right angle to its rotation
the resultant force on it is increased continuously
The correct Answer is:B
To solve the problem of finding the ratio of the moment of inertia about an axis perpendicular to a rectangular plate passing through points O and O', we can follow these steps:
Step 1: Understand the Geometry
We have a rectangular plate with dimensions 60 cm and 80 cm. The points O and O' are located such that O is perpendicular to the plate. We need to find the distance between O and O' using the Pythagorean theorem.
Step 2: Calculate the Length OP
Using the Pythagorean theorem:
OP=√(60cm)2+(80cm)2
Calculating:
OP=√3600+6400=√10000=100cm
Step 3: Find the Distance OO'
Since O' is the midpoint of OP, we can find OO' as:
OO′=OP2=100cm2=50cm
Step 4: Moment of Inertia about O'
The moment of inertia IO′ about point O' can be calculated using the formula:
IO′=m12(a2+b2)
where a=60cm and b=80cm. Thus:
IO′=m12(602+802)=m12(3600+6400)=m12(10000)=10000m12
Step 5: Moment of Inertia about O
Using the parallel axis theorem, the moment of inertia about point O can be calculated as:
IO=IO′+m⋅d2
where d=OO′=50cm:
IO=10000m12+m⋅(502)=10000m12+m⋅2500
Factoring out m:
IO=m(1000012+2500)
Step 6: Simplify the Expression for IO
To combine the terms:
IO=m(1000012+2500⋅1212)=m(10000+3000012)=m(4000012)
Step 7: Find the Ratio of IO to IO′
Now, we can find the ratio:
IOIO′=m⋅4000012m⋅1000012=4000010000=4
Step 8: Final Ratio
Thus, the ratio of the moment of inertia about O to that about O' is:
IOIO′=4
We have a rectangular plate with dimensions 60 cm and 80 cm. The points O and O' are located such that O is perpendicular to the plate. We need to find the distance between O and O' using the Pythagorean theorem.
Using the Pythagorean theorem:
OP=√(60cm)2+(80cm)2
Calculating:
OP=√3600+6400=√10000=100cm
Since O' is the midpoint of OP, we can find OO' as:
OO′=OP2=100cm2=50cm
The moment of inertia IO′ about point O' can be calculated using the formula:
IO′=m12(a2+b2)
where a=60cm and b=80cm. Thus:
IO′=m12(602+802)=m12(3600+6400)=m12(10000)=10000m12
Using the parallel axis theorem, the moment of inertia about point O can be calculated as:
IO=IO′+m⋅d2
where d=OO′=50cm:
IO=10000m12+m⋅(502)=10000m12+m⋅2500
Factoring out m:
IO=m(1000012+2500)
To combine the terms:
IO=m(1000012+2500⋅1212)=m(10000+3000012)=m(4000012)
Now, we can find the ratio:
IOIO′=m⋅4000012m⋅1000012=4000010000=4
Thus, the ratio of the moment of inertia about O to that about O' is:
IOIO′=4
Similar Questions
What are the speeds of two objects if, when they move uniformly towards each other, they get 4 m closer in each second and when they move uniformly in the same direction with the original speeds, they get 4 m closer each 10 s?
View SolutionA particle is moving uniformly in a circular path of radius r. When it moves through an angular displacement θ, then the magnitude of the corresponding linear displacement will be
View SolutionAn object is moving with a uniform acceleration which is parallel to its instantaneous direction of motion. The dispalcement (s)-velocity (v) graph of this object is.
View SolutionAn object is moving with a uniform acceleration which is parallel to its instantaneous direction of motion. The dispalcement (s)-velocity (v) graph of this object is.
View SolutionAn object is moving with a uniform acceleration which is parallel to its instantaneous direction of motion. The displacement velocity (v) graph of this object is
View SolutionAn object revolves uniformly in a circle of diameter 0.80 m and completes 100 rev min−1. Find its time period and angular velocity.
View SolutionAn object is moving towards a moving mirror as shown in the figure. Find speed of image with respect to ground in m/sec.
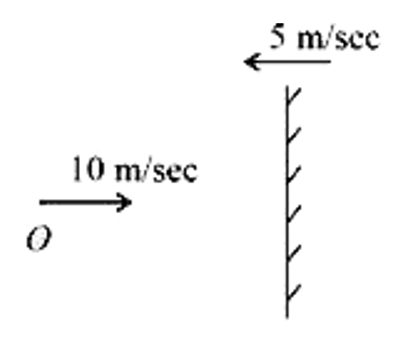
View SolutionDraw the velocity-time graph of an object moving with uniform negative acceleration.
View SolutionAn object of mass 15kg is moving with a uniform velocity of 4m/s. what is the kinetic energy possessed by the object ?
View SolutionAn object is moving in a circle at constant speed v. The magnitude of rate of change of momentum of the object.
View Solution
