An object is moving with a uniform acceleration which is parallel to its instantaneous direction of motion. The displacement velocity (v) graph of this object is

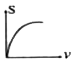

The correct Answer is:C
To solve the problem of determining the displacement-velocity (v) graph of an object moving with uniform acceleration parallel to its instantaneous direction of motion, we can follow these steps:
1. Understanding Uniform Acceleration:
- When an object is moving with uniform acceleration, it means that its acceleration (a) is constant over time. This implies that the change in velocity (v) is uniform.
2. Relating Displacement and Velocity:
- The relationship between displacement (s), initial velocity (u), final velocity (v), and acceleration (a) can be described by the equation:
v2=u2+2as
- Here, s is the displacement, u is the initial velocity, and v is the final velocity.
3. Graphing Displacement vs. Velocity:
- Rearranging the equation gives:
s=v2−u22a
- This equation indicates that displacement (s) is proportional to the square of the velocity (v) when acceleration (a) is constant.
4. Shape of the Graph:
- The graph of displacement (s) versus velocity (v) will be a parabola since s is a quadratic function of v. Specifically, it will open upwards, starting from the point where v=u (initial velocity).
5. Conclusion:
- Therefore, the displacement-velocity graph of the object moving with uniform acceleration parallel to its instantaneous direction of motion is a parabola.
- When an object is moving with uniform acceleration, it means that its acceleration (a) is constant over time. This implies that the change in velocity (v) is uniform.
- The relationship between displacement (s), initial velocity (u), final velocity (v), and acceleration (a) can be described by the equation:
v2=u2+2as
- Here, s is the displacement, u is the initial velocity, and v is the final velocity.
- Rearranging the equation gives:
s=v2−u22a
- This equation indicates that displacement (s) is proportional to the square of the velocity (v) when acceleration (a) is constant.
- The graph of displacement (s) versus velocity (v) will be a parabola since s is a quadratic function of v. Specifically, it will open upwards, starting from the point where v=u (initial velocity).
- Therefore, the displacement-velocity graph of the object moving with uniform acceleration parallel to its instantaneous direction of motion is a parabola.
Topper's Solved these Questions
- MOTION IN A STRAIGHT LINE BOOK - AAKASH SERIESCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - EXERCISE -II
80 Videos
- MOTION IN A STRAIGHT LINE BOOK - AAKASH SERIESCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - PRACTICE EXERCISE
58 Videos
- MOTION IN A STRAIGHT LINE BOOK - AAKASH SERIESCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - very Short answer type question
15 Videos
- MOTION IN A PLANEBOOK - AAKASH SERIESCHAPTER - MOTION IN A PLANEEXERCISE - QUESTION FOR DESCRIPTIVE ANSWER
7 Videos
- MOVING CHARGES AND MAGNETISMBOOK - AAKASH SERIESCHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - EXERCISE-III
49 Videos
Similar Questions
An object is moving with a uniform acceleration which is parallel to its instantaneous direction of motion. The dispalcement (s)-velocity (v) graph of this object is.
View SolutionAn object is moving with a uniform acceleration which is parallel to its instantaneous direction of motion. The dispalcement (s)-velocity (v) graph of this object is.
View Solution
Knowledge Check
Starting from rest a body moves with uniform acceleration and acquires a velocity v after n seconds. Then its displacement in the last two seconds is
A2v(n+1)/nB2v(n−1)/nC2v(n+1)2/nDv(n−1)2n
The displacement (s)- velocity ( v ) graph of a particle if it starts moving from rest with a uniform acceleration which is parallel to its instantaneous direction of motion is
View SolutionThe slope of velocity-time graph for uniform motion of an object is
View SolutionAssertion: A uniform circular motion is an acceleration motion.
Reason: Direction of acceleration is parallel to velocity vector.
View SolutionCan an object accelerate If its velocity is constant?
View SolutionState which of the following situations are possible and give an example for each of these :
(a) An body with a constant acceleration but with zero velocity.
(b) An object moving with an acceleration but with uniform speed
(c) An object moving in a certain direction with an acceleration in the perpendicular direction.
View SolutionA : The relative acceleration of two objects moving under the effect of gravity, only is always zero, irrespective of direction of motion.
R : The acceleration of object moving under the effect of gravity have acceleration always in downward direction and is independent from size and mass of object.
View SolutionSay Yes or No:
a. Can an object moving towards north have acceleration towards south?
b. Can an object reverse the direction of its motion even thouth it has reverse the direcleration?
C. Can an object reverse the derction of its acceleration even though it continues to move in the same dirction?
d. Average speed is the magnitude of average velocity
e. At any instant of time. the directions of change in velocity and acceleration are differnt.
View Solution
