A 24 V battery of internal resistance 4.0Ω is connected to a variable resistance. At what value of the current drawn from the battery is the rate of heat produced in the resistor maximum?
(a)2A
(b)3A
(c)4A
(d)6A
The correct Answer is:B
To solve the problem step by step, we need to determine the current at which the rate of heat produced in the resistor is maximum.
Step 1: Understand the circuit
We have a battery with an electromotive force (emf) of E=24V and an internal resistance r=4Ω. The battery is connected to a variable resistance R.
Step 2: Condition for maximum heat
The rate of heat produced in the resistor is maximum when the resistance of the resistor R is equal to the internal resistance r of the battery. Therefore, we set:
R=r=4Ω
Step 3: Calculate total resistance
The total resistance Rtotal in the circuit is the sum of the internal resistance and the variable resistance:
Rtotal=r+R=4Ω+4Ω=8Ω
Step 4: Calculate the current
Using Ohm's law, the current I drawn from the battery can be calculated as:
I=ERtotal=24V8Ω=3A
Conclusion
The current drawn from the battery at which the rate of heat produced in the resistor is maximum is:
3A
We have a battery with an electromotive force (emf) of E=24V and an internal resistance r=4Ω. The battery is connected to a variable resistance R.
The rate of heat produced in the resistor is maximum when the resistance of the resistor R is equal to the internal resistance r of the battery. Therefore, we set:
R=r=4Ω
The total resistance Rtotal in the circuit is the sum of the internal resistance and the variable resistance:
Rtotal=r+R=4Ω+4Ω=8Ω
Using Ohm's law, the current I drawn from the battery can be calculated as:
I=ERtotal=24V8Ω=3A
The current drawn from the battery at which the rate of heat produced in the resistor is maximum is:
3A
Similar Questions
A battery of emf 2V and internal resistance 0.5(Ω)is connected across a resistance 1.5(Ω). Find the current flow through battery ?
View SolutionA battery of e.m.f. 10V and internal resistance 0.5ohm is connected across a variable resistance R. The value of R for which the power delivered in it is maximum is given by
View Solution
Knowledge Check
A battery of emf 15 V and internal resistance of 4Ω is connected to a resistor. If the current in the circuit is 2A and the circuit is closed. Resistance of the resistor and terminal voltage of the battery will be
A2.5Ω,6VB3.5Ω,6VC2.5Ω,7VD3.5Ω,7VA battery having 12V emf and internal resistance 3Ω is connected to a resistor. If the current in the circuit is 1A, then the resistance of resistor and lost voltage of the battery when circuit is closed will be
A7Ω,7VB8Ω,8VC9Ω,9VD9Ω,10V
A battery of emf 10 V and internal resistance 3Ω is connected to a resistor. If the current in the circuit is 0.5 A, what is the resistance of the resistors ? What is the terminal voltage of the battery when the circuit is closed ?
View SolutionA battery of emf E has an internal resistance r. A variable resistacne R is connected to the terminals of the battery. A current i is drawn from the battery. V is the terminal potential difference. If R alone is gradually reduced to zero, which of the following best describes i and V?
View SolutionA battery of internal resistance 4Ω is connected to the network of resistance as shown . In order that the maximum power can be delivered to the network, the value of R in Ω should be
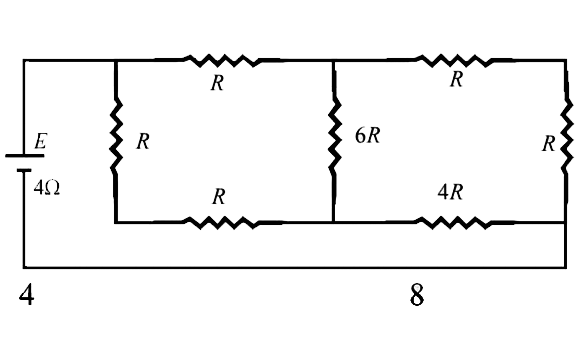 .
.
View SolutionA battery of internal resistance 4Ω is connected to the network of the resistance as shown in figure . If the maximum power can be delivered to the network, the magnitude of resistance in Ωshould be
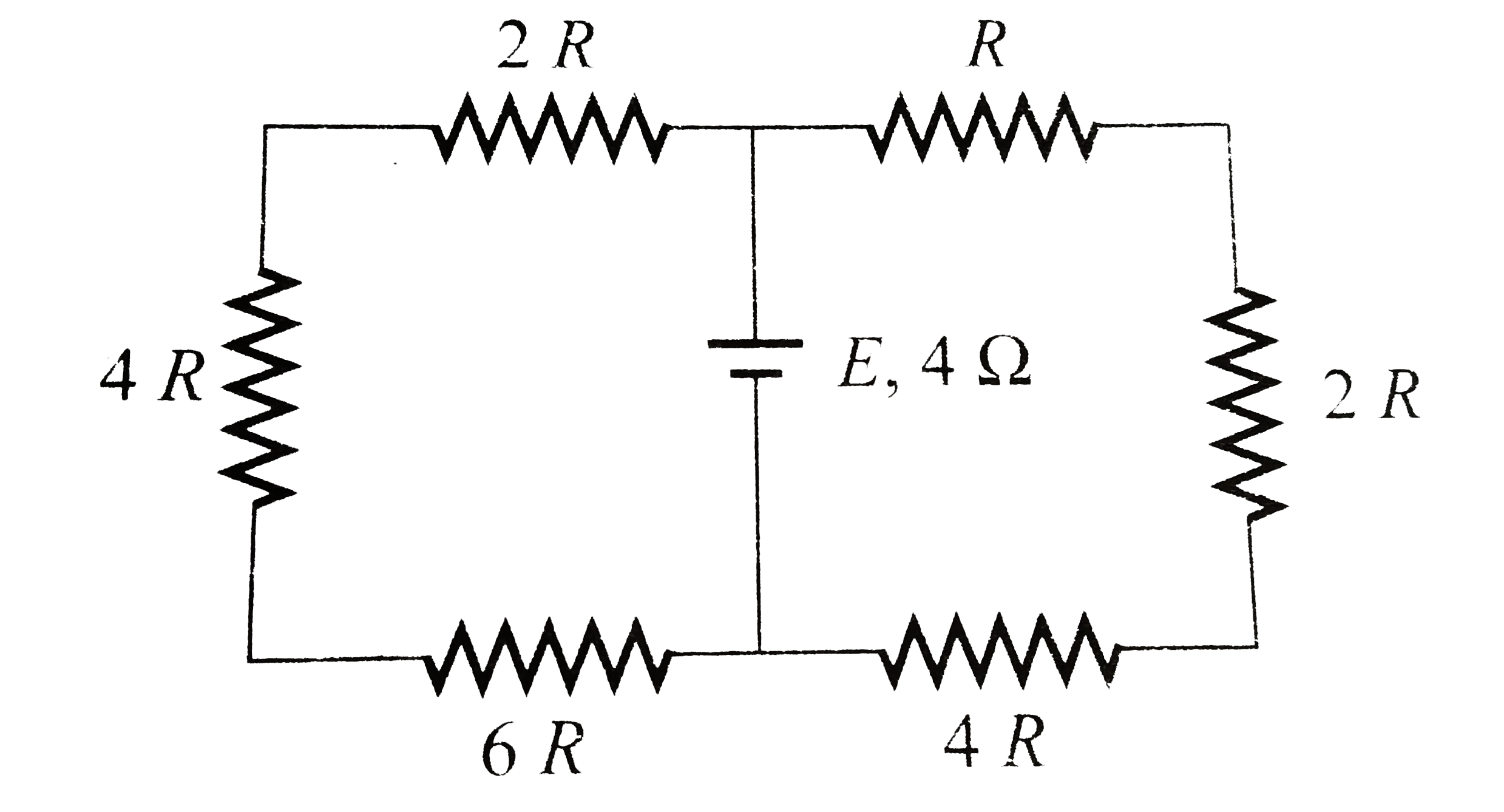
View SolutionA 5 V battery of negligible internal resistance is connected across a 200 V battery and a resistance of 39Ω as shown in the figure. Find the value of the current.

View SolutionA battery of e.m.f. 15 V and internal resistance 3Ω is connected to two resistors 3 Ω and 6 Ω connected in parallel. Find :
the current through the battery,
View Solution
