A beaker of height H is made up of a material whose coefficient of linear thermal expansion is 3α. It is filled up to the brim by a liquid whose coefficient of volume expansion is α. If now the beaker along with its contents is uniformly heated through a small temperature T, the level of liquid will reduced by (Given, αT<<1)
αTH
3αTH
9αTH
6αTH
The correct Answer is:B
To solve the problem, we need to analyze the thermal expansion of both the beaker and the liquid when they are heated. Here’s a step-by-step breakdown:
Step 1: Understand the thermal expansion of the beaker
The beaker has a height H and is made of a material with a coefficient of linear thermal expansion 3α. When the temperature increases by T, the change in height of the beaker can be expressed as:
ΔHbeaker=H⋅3αT
Step 2: Determine the change in volume of the liquid
The liquid has a coefficient of volume expansion α. The volume of the liquid will change as follows:
ΔVliquid=V⋅αT
where V is the initial volume of the liquid. Since the beaker is filled to the brim, the initial volume V can be expressed as:
V=A⋅H
where A is the cross-sectional area of the beaker.
Step 3: Calculate the final volume of the liquid
The final volume of the liquid after heating becomes:
V′=V+ΔVliquid=A⋅H+A⋅H⋅αT=A⋅H(1+αT)
Step 4: Calculate the final height of the liquid
The final height of the liquid in the beaker after heating can be expressed as:
h′=V′A′
where A′ is the new cross-sectional area of the beaker after expansion. The new area A′ can be calculated as:
A′=A(1+6αT)
Thus, the final height of the liquid is:
h′=A⋅H(1+αT)A(1+6αT)=H(1+αT)1+6αT
Step 5: Calculate the reduction in the level of the liquid
The reduction in the level of the liquid is given by:
Δh=H−h′=H−H(1+αT)1+6αT
This can be simplified as:
Δh=H(1−(1+αT)(1+6αT))
=H((1+6αT)−(1+αT)(1+6αT))
=H(5αT1+6αT)
Step 6: Approximate the reduction in level
Given that αT≪1, we can approximate:
Δh≈5αTH
However, since we need to consider the expansion of the beaker as well, the effective reduction in liquid level can be approximated as:
Δh≈3αTH
Conclusion
Thus, the reduction in the level of the liquid is:
Δh=3αTH
The correct option is the second one: 3αTH.
The beaker has a height H and is made of a material with a coefficient of linear thermal expansion 3α. When the temperature increases by T, the change in height of the beaker can be expressed as:
ΔHbeaker=H⋅3αT
The liquid has a coefficient of volume expansion α. The volume of the liquid will change as follows:
ΔVliquid=V⋅αT
where V is the initial volume of the liquid. Since the beaker is filled to the brim, the initial volume V can be expressed as:
V=A⋅H
where A is the cross-sectional area of the beaker.
The final volume of the liquid after heating becomes:
V′=V+ΔVliquid=A⋅H+A⋅H⋅αT=A⋅H(1+αT)
The final height of the liquid in the beaker after heating can be expressed as:
h′=V′A′
where A′ is the new cross-sectional area of the beaker after expansion. The new area A′ can be calculated as:
A′=A(1+6αT)
Thus, the final height of the liquid is:
h′=A⋅H(1+αT)A(1+6αT)=H(1+αT)1+6αT
The reduction in the level of the liquid is given by:
Δh=H−h′=H−H(1+αT)1+6αT
This can be simplified as:
Δh=H(1−(1+αT)(1+6αT))
=H((1+6αT)−(1+αT)(1+6αT))
=H(5αT1+6αT)
Given that αT≪1, we can approximate:
Δh≈5αTH
However, since we need to consider the expansion of the beaker as well, the effective reduction in liquid level can be approximated as:
Δh≈3αTH
Thus, the reduction in the level of the liquid is:
Δh=3αTH
The correct option is the second one: 3αTH.
Similar Questions
A liquid with coefficient of volume expansion γ is filled in a container of a material having coefficient of linear expansion α . If the liquid overflows on heating, then
View SolutionA liquid with coefficient of volume expansion γ is filled in a container of a material having coefficient of linear expansion α . If the liquid overflows on heating, then
View SolutionOn heating a liquid having coefficient of volume expension α in a container having coefficient of linear expansion α/2, the level of the liquid in the container would
View SolutionA fluid having a thermal coefficient of volume expansion γ is filled in a cylindrical vessel up to a height h0. The coefficient of linear thermal expansion of the material of the vessel is α . If the fluid is heated with the vessel , then find the level of liquid when temperature increases by Δθ.
View SolutionWhen a liquid is taken in a long cylindrical vessel of material, with linear coeffcient of expansion α, is heated, the level of liquid did not change. The volume coefficient of expansion of liquid is
View SolutionTwo straight metallic strips each of thickness t and length l are rivetted together. Their coefficients of linear expansions areα1andα2. If they are heated through temperature ΔT, the bimetallic strip will bend to form an arc of radius
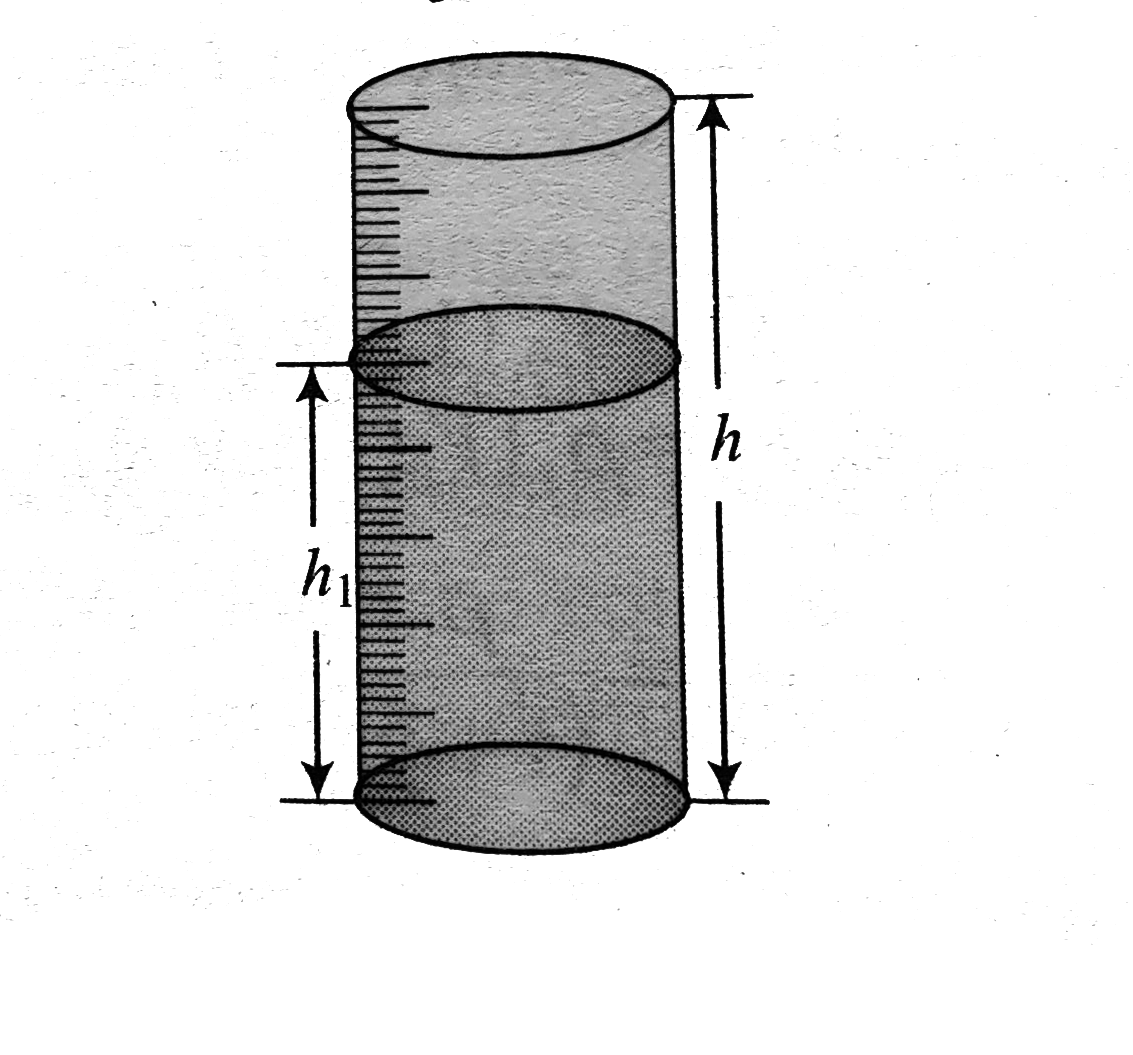
View SolutionConsider a cylindrical container of cross-section area A length h and having coefficient of linear expansion αc. The container is filled by liquid of real expansion coefficient γL up to height h1. When temperature of the system is increased by Δθ then
(a). Find out the height, area and volume of cylindrical container and new volume of liquid.
(b). Find the height of liquid level when expansion of container is neglected.
(c). Find the relation between γL and αc for which volume of container above the liquid level
(i) increases
(ii). decreases
(iii). remains constant.
(d). On the surface of a cylindrical container a scale is attached for the measurement of level of liquid of liquid filled inside it. If we increase the temperature of the temperature of the system by Δθ, then
(i). Find height of liquid level as shown by the scale on the vessel. Neglect expansion of liquid.
(ii). Find the height of liquid level as shown by the scale on the vessel. Neglect expansion of container.
View SolutionTwo rods of length l1 and l2 are made of material whose coefficient of linear expansion are α1 and α2 , respectively. The difference between their lengths will be independent of temperatiure if l1/l2 is to
View SolutionIn the shown planar frame made of thin uniform rods, the length of section AB and EF is l1 and its thermal linear coefficient of expansion is α1. The length of section CD is l2 and its thermal linear coefficient of expansion is α2.CBandDE are of same length having thermal linear coefficient of expansion α2. point A,B,EandF reside on same line, that is, sections ABandEF overlap. then the ratio of l1l2 for which the distance between end A and end F remains the same at all temperatures, is

View SolutionThe height of a mercury column measured with a brass scale, which is correct and equal to H0 at 0∘C, is H1 at t∘C? The coefficient of linear expansion of brass is α and the coefficient of linear expansion of brass is α and the corfficient of volume expansion of mercury is γ. Relate H0 and H1.
View Solution
