A small body is released from point A of smooth parabolic path y=x2, where y is vertical axis and x is horizontal axis at ground, as shown. The body leaves the surface from point B. If g=10ms−2 then what is the value of d (in m)?
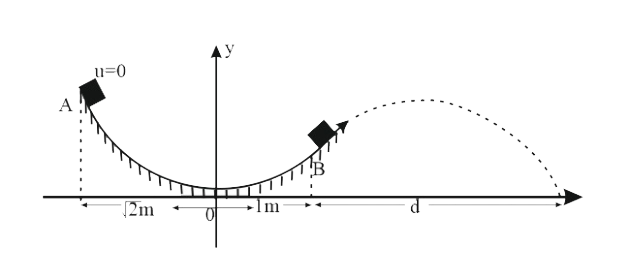
The correct Answer is:2
Step by step video & image solution for A small body is released from point A of smooth parabolic path y=x^(2), where y is vertical axis and x is horizontal axis at ground, as shown. The body leaves the surface from point B. If g = 10ms^(-2) then what is the value of d (in m)? by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Similar Questions
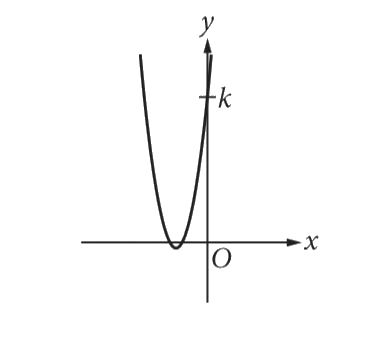
The graph of y=2x2+10x+12 is shown. If the graph crosses the y -axis at the point ( 0, k) , what is the value of k ?
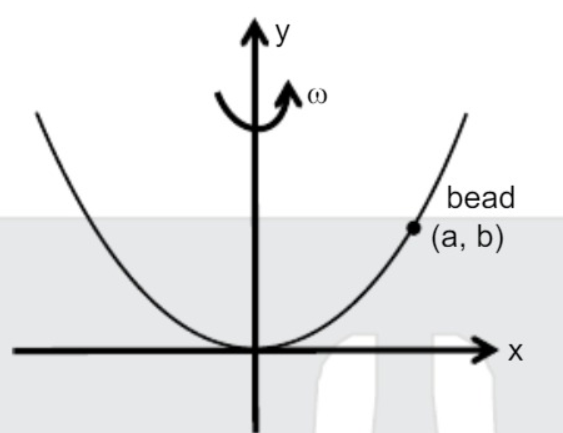
View SolutionAngular velocity of smooth parabolic wire y = 4c(x)^(2) about axis of parabola in vertical plane if bead of mass m does not slip at (a,b) will be
View SolutionFind the point on the curve y=x2−2x+3 , where the tangent is parallel to x-axis.
View Solution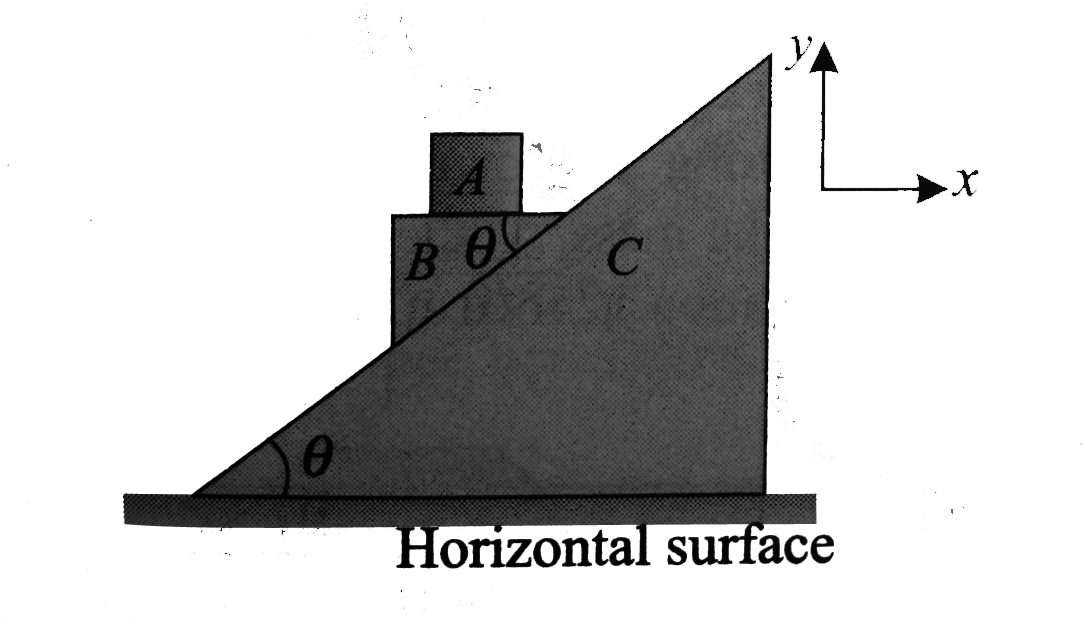
In the figure shown all the surface are smooth. All the blocks A,B and C are movable x-axis is horizontal and y-axis vertical as shown. Just after the system is relased from the position as shown.
View Solution
In the figure shown all the surface are smooth. All the blocks A,B and C are movable x-axis is horizontal and y-axis vertical as shown. Just after the system is relased from the position as shown.
View SolutionA bead of mass m is located on a parabolic wire with its axis vertical and vertex at the origin as shown in figure and whose equastion is x2=4ay. The wire frame is fixed and the bead is released from the point y=4a on the wire frame from rest. The tangential acceleration of the bead when it reaches the position given by y=a is

View SolutionA bead of mass m is located on a parabolic wire with its axis vertical and vertex at the origin as shown in figure and whose equastion is x2=4ay. The wire frame is fixed and the bead is released from the point y=4a on the wire frame from rest. The tangential acceleration of the bead when it reaches the position given by y=a is

View SolutionThe number of points on the curve y=x3−2x2+x−2 where tangents are prarllel to x-axis, is
View SolutionFor ground to ground projectile motion equation of path is y=12x−3/4x2. Given that g=10ms−2. What is the range of the projectile ?
View SolutionFind the points on the curve 2a2y=x3−3ax2 where the tangent is parallel to x-axis.
View Solution
