A random variable X follows binomial probability distribution with probability P(X), with mean as 2, probability of success as p and probability of failure as q such that p+q=1. If ΣX2P(X)=285, then the probability of exactly 2 success is
3×214510
32×21659
3×(25)10
45×(25)9
The correct Answer is:B
Step by step video & image solution for A random variable X follows binomial probability distribution with probability P(X), with mean as 2, probability of success as p and probability of failure as q such that p+q=1. If SigmaX^(2)P(X)=(28)/(5), then the probability of exactly 2 success is by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Similar Questions
Knowledge Check
IF the mean and the variance of a binomial distribution are 4 and 3 respectively , then the probability of six successes is
A.16C6(14)6(34)10B.16C6(14)10(34)6C.12C6(14)6(34)6D.12C6(14)6(34)10
In a probability distribution of a random variable X , the sum of probabilities is always
View SolutionIf a random variable X follows binomial distribution with mean 3 and variance 3/2, find P(X≤5).
View SolutionFor the following probability distribution.
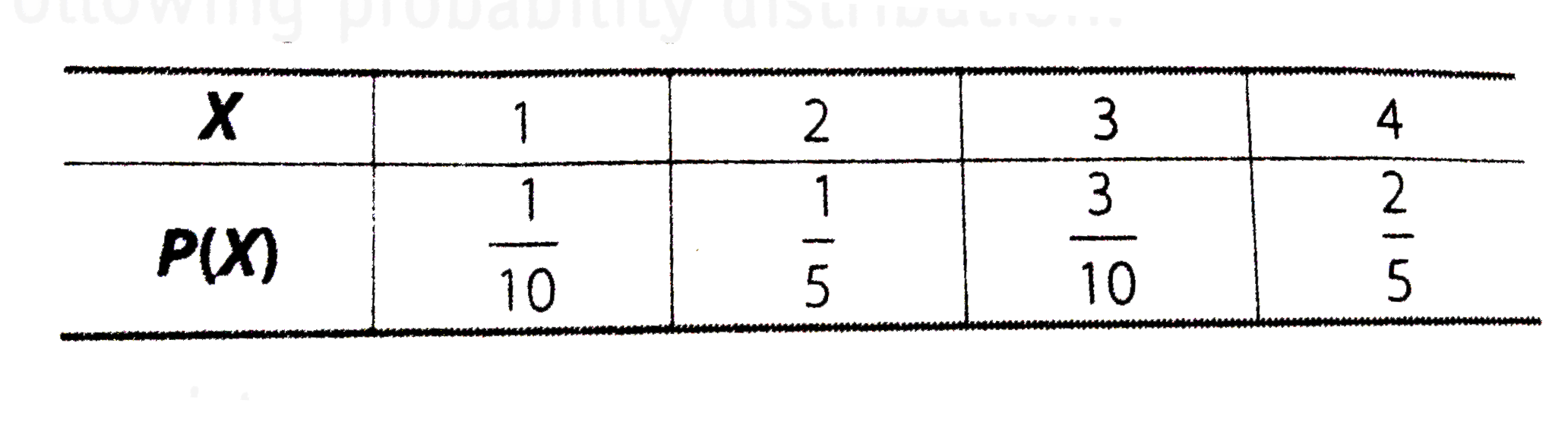
E(X2) is equal to
View SolutionFor the following probability distribution :
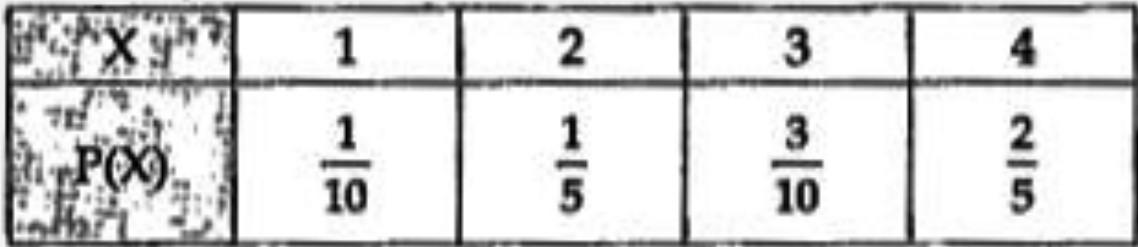
E(X2) is equal to
View Solution
is the probability distribution of a random variable X. Find the variance of X.
View Solution
is the probability distribution of a random variable X. Find the variance of X.
View SolutionIf there are n independent trials, p and q are the probability of success and failure respectively, then probability of exactly r success
View Solution


