With what minimum speed must a particle be projected from origin so that it is able to pass through a given point (30m,40m) ? Take g=10m/s2
60ms−1
30ms−1
50ms−1
40ms−1
The correct Answer is:B
To find the minimum speed with which a particle must be projected from the origin to pass through the point (30 m, 40 m), we can use the equations of projectile motion.
1. Understand the Problem: We need to determine the minimum initial velocity u required for a projectile to reach the point (30 m, 40 m) when projected from the origin.
2. Use the Trajectory Equation: The trajectory equation for projectile motion is given by:
y=xtanθ−gx22u2cos2θ
where g is the acceleration due to gravity, θ is the angle of projection, u is the initial velocity, x is the horizontal distance, and y is the vertical distance.
3. Substitute Known Values: For the point (30 m, 40 m), we substitute x=30 m, y=40 m, and g=10 m/s² into the trajectory equation:
40=30tanθ−10⋅3022u2cos2θ
4. Simplify the Equation: Rearranging the equation gives:
40=30tanθ−4500u2cos2θ
This can be rewritten as:
30tanθ−40=4500u2cos2θ
5. Define a Function: Let:
f(θ)=3tanθ−4
Then, we can express u2 as:
u2=4500cos2θf(θ)
6. Minimize u: To minimize u, we need to maximize f(θ).
7. Differentiate f(θ): We differentiate f(θ):
f′(θ)=3sec2θ
Setting f′(θ)=0 does not yield a maximum since sec2θ is always positive. Thus, we need to analyze the behavior of f(θ).
8. Find Critical Points: We can find the maximum value of f(θ) by using the identity tanθ=yx=4030=43. Therefore:
tanθ=43
and using the Pythagorean identity:
cos2θ=11+tan2θ=11+(43)2=925
9. Calculate u2: Substitute tanθ and cos2θ back into the equation for u2:
f(θ)=3⋅43−4=0
This means we need to find the minimum speed using the maximum value of cos2θ:
u2=4500925⋅0 (which is not valid)
So we need to find a valid angle that gives us a positive value.
10. Final Calculation: After solving for u:
u2=4500⋅259=12500⟹u=√12500=30 m/s
Conclusion:
The minimum speed required for the particle to pass through the point (30 m, 40 m) is 30 m/s.
y=xtanθ−gx22u2cos2θ
where g is the acceleration due to gravity, θ is the angle of projection, u is the initial velocity, x is the horizontal distance, and y is the vertical distance.
40=30tanθ−10⋅3022u2cos2θ
40=30tanθ−4500u2cos2θ
This can be rewritten as:
30tanθ−40=4500u2cos2θ
f(θ)=3tanθ−4
Then, we can express u2 as:
u2=4500cos2θf(θ)
f′(θ)=3sec2θ
Setting f′(θ)=0 does not yield a maximum since sec2θ is always positive. Thus, we need to analyze the behavior of f(θ).
tanθ=43
and using the Pythagorean identity:
cos2θ=11+tan2θ=11+(43)2=925
f(θ)=3⋅43−4=0
This means we need to find the minimum speed using the maximum value of cos2θ:
u2=4500925⋅0 (which is not valid)
So we need to find a valid angle that gives us a positive value.
u2=4500⋅259=12500⟹u=√12500=30 m/s
The minimum speed required for the particle to pass through the point (30 m, 40 m) is 30 m/s.
Similar Questions
A body is projected from the ground with some speed at some angle with the horizontal. Taking the horizontal and vertical direction to be x and y axis respectively and the point of projection as origin, calculate the minimum speed (in ms−1) of projection so that it can pass through a point whose x and y coordinates are 30 m and 40 m respectively? Take g=10ms−2
View SolutionTrack OABCD (as shown in figure ) is smooth. What minimum speed has to be given to a particle lying at point A, so that it can reach point C? (take g=10m/s2)
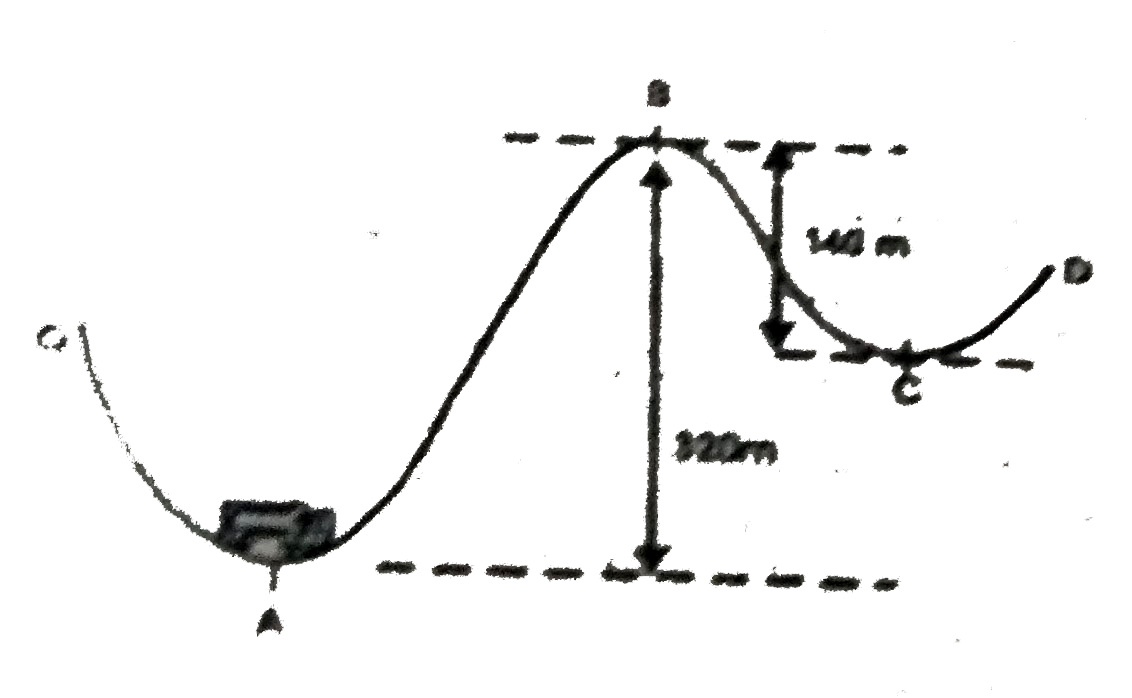
View SolutionA ball is projected vertically up with speed 20 m/s. Take g=10m/s2
View SolutionA particle is projected with velocity of 10ms at an angle of 15° with horizontal.The horizontal range will be(g=10ms2)
View SolutionA particle is projected from ground with velocity 20(√2)m/s at 45∘. At what time particle is at height 15 m from ground? (g=10m/s2)
View SolutionA particle is projected from ground with velocity 40√2m/s at 45∘. At time t=2s
View SolutionMatch the following

A particle is projected from a large fixed incline plane as shown. Here (Vertically downward) take g=10m/s2.
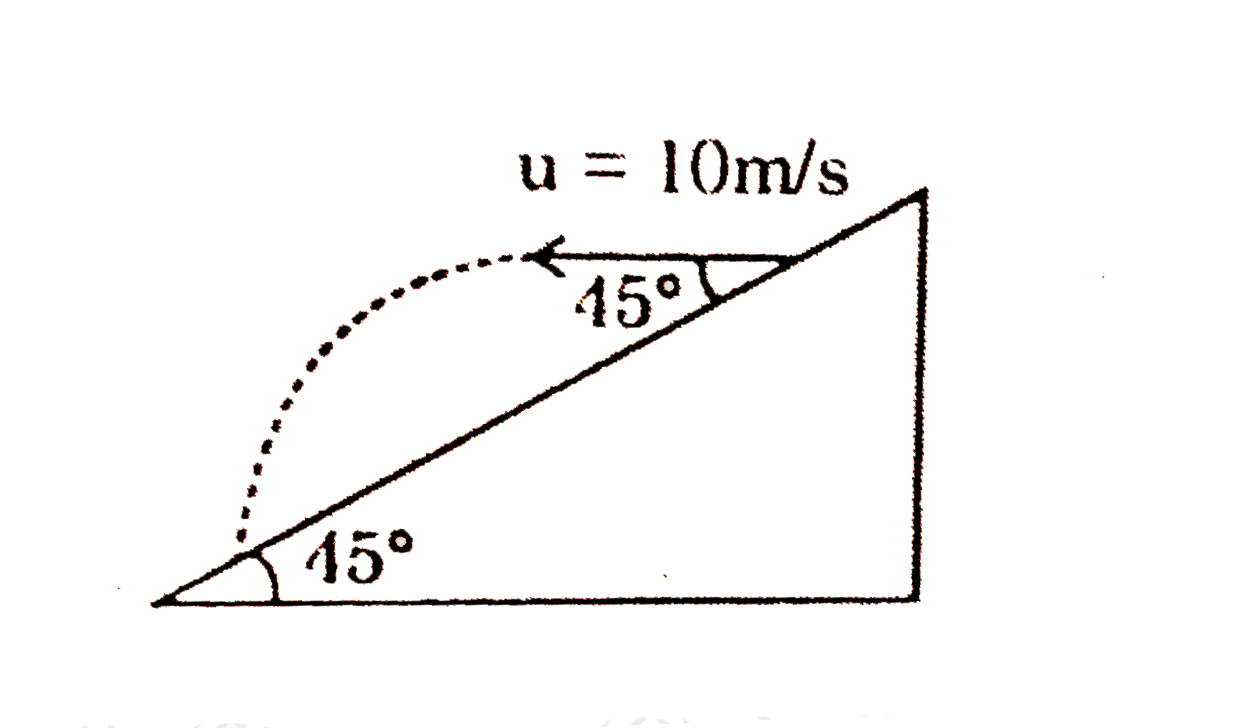
View SolutionA particle is thrown vertically up from the top of a building of height 20m with initial velocity u. A second particle is released from the same point 1 second later. If both particles reach the ground at the same instant ,3u=m/s. [Takeg=10m/s2]
View SolutionA ball is projected from a point O as shown in figure it will strike the ground after (g = 10 m/s2 )
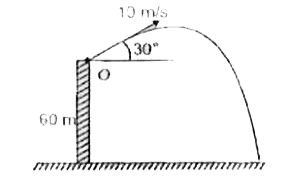
View SolutionA particle A is projected from the ground with an initial velocity of 10m/s at an angle of 60∘ with horizontal. From what height should an another particle B be projected horizontally with velocity 5m/s so that both the particles collide in ground at point C if both are projected simultaneously g=10m/s2.

View Solution