Which of the following cylindrical rods will conduct most heat, when their ends are maintained at the same steady temperature
l=1m,r=0.2m
l=1m,r=0.1m
l=10m,r=0.1m
l=0.1m,r=0.3m
The correct Answer is:D
To determine which cylindrical rod will conduct the most heat when their ends are maintained at the same steady temperature, we can use the formula for heat conduction through a cylindrical rod:
Q=−K⋅A⋅ΔTL
Where:
- Q is the amount of heat conducted,
- K is the thermal conductivity of the material,
- A is the cross-sectional area,
- ΔT is the temperature difference,
- L is the length of the rod.
Since the ends of the rods are maintained at the same steady temperature, we can assume that ΔT is constant for all rods.
1. Identify the Cross-Sectional Area:
The cross-sectional area A of a cylindrical rod is given by:
A=πr2
where r is the radius of the rod.
2. Substitute into the Heat Conduction Formula:
The heat conducted can be expressed as:
Q=−K⋅(πr2)⋅ΔTL
This can be simplified to:
Q=C⋅r2L
where C=−K⋅π⋅ΔT is a constant for the same material and temperature difference.
3. Calculate r2L for Each Rod:
For each rod, we will calculate the ratio r2L:
- Rod A: r=0.2m,L=1m
r2L=(0.2)21=0.041=0.04
- Rod B: r=0.1m,L=1m
r2L=(0.1)21=0.011=0.01
- Rod C: r=0.1m,L=10m
r2L=(0.1)210=0.0110=0.001
- Rod D: r=0.3m,L=0.1m
r2L=(0.3)20.1=0.090.1=0.9
4. Compare the Values:
Now we compare the calculated values of r2L:
- Rod A: 0.04
- Rod B: 0.01
- Rod C: 0.001
- Rod D: 0.9
The maximum value is for Rod D, which indicates that it will conduct the most heat.
5. Conclusion:
Therefore, the rod that will conduct the most heat is Rod D.
- Q is the amount of heat conducted,
- K is the thermal conductivity of the material,
- A is the cross-sectional area,
- ΔT is the temperature difference,
- L is the length of the rod.
The cross-sectional area A of a cylindrical rod is given by:
A=πr2
where r is the radius of the rod.
The heat conducted can be expressed as:
Q=−K⋅(πr2)⋅ΔTL
This can be simplified to:
Q=C⋅r2L
where C=−K⋅π⋅ΔT is a constant for the same material and temperature difference.
For each rod, we will calculate the ratio r2L:
- Rod A: r=0.2m,L=1m
r2L=(0.2)21=0.041=0.04
- Rod B: r=0.1m,L=1m
r2L=(0.1)21=0.011=0.01
- Rod C: r=0.1m,L=10m
r2L=(0.1)210=0.0110=0.001
- Rod D: r=0.3m,L=0.1m
r2L=(0.3)20.1=0.090.1=0.9
Now we compare the calculated values of r2L:
- Rod A: 0.04
- Rod B: 0.01
- Rod C: 0.001
- Rod D: 0.9
Therefore, the rod that will conduct the most heat is Rod D.
Similar Questions
Rate of heat flow through two conducting rods of identical dimensions having thermal conductivities K1 and K2 and Q1 and Q2 when their ends are maintained at the same difference of temperature individually. When the two rods are joined in series with their ends maintained at the same temperature difference (as shown in the figure), the rate of heat flow will be

View SolutionWhich of the following circular rods (given radius r and length l) each made of the same material and whose ends are maintained at the same temperature will conduct most heat?
View SolutionA thermal conductor is heated at one end. What is the theoretical value of its thermal conductivity in order that the other end also attains the same temperature?
View SolutionThe end of two rods of different materials with their thermal conductivities, area of cross-section and lengths all in the ratio 1:2 are maintained at the same temperature difference. If the rate of flow of heat in the first rod is 4cal/s. Then, in the second rod rate of heat flow in cal/s will be
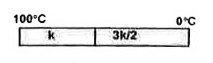
View SolutionTwo rods of similar area of cross section and length are joined as shown in the figure. The ratio of their thermal conductivity is 2:3. The free ends are maintained at 100∘C and 0∘C respectivley. THE temperature at junction in steady state is
View SolutionA cylinder of radius r made of a material of thermal conductivity K1 is surrounded by a cylindrical shell of inner radius r and outer radius 2r made of a material of thermal conductivity K2. The two ends of the combined system are maintained at two different temperatures. There is no loss of heat across the cylindrical surface and the system is in steady state. Show that the effective thermal conductivity of the system is (K1+3K2)/4.
View SolutionA cylinder of radius R made of a material of thermal conductivity K1 is surrounded by a cylindrical shell of inner radius R and outer radius 2R made of a material of thermal conductivity K2. The two ends of the combined system are maintained at two different temperatures. There is no loss of heat across the cylindrical surface and the system is in steady state. The effective thermal conductivity of the system is
View SolutionA cylinder of radius R made of a material of thermal conductivity K1 is surrounded by cylindrical shell of inner radius R and outer radius 2R made of a material of thermal con-ductivity K2 The two ends of the combined system are maintained at two differnet tem-peratures There is no loss of heat across the cylindrical surface and system is in steady state What is the effective thermal conductivity of the system
 .
.
View SolutionThe two ends of a metal rod are maintained at temperature 100∘C and 110∘C. The rate of heat flow in the rod is found to be 4.0J/s. If the ends are maintained at temperature s 200∘C and 210∘C. The rate of heat flow will be
View SolutionTwo rods (one semi-circular and other straight) of same material and of same cross-sectional area are joined as shown in the figure. The point A and B are maintained at different temperature. Find the ratio of the heat transferred through a cross-section of a semi-circular rod to the heat transferred through a cross section of the straight rod in a given time.

View Solution
