The RMS value of AC which when passed through a resistor produces heat, which is twice that produced by a steady current of 1.414A in the same resistor is
2A
3.46 A
2.818 A
1.732 A
The correct Answer is:A
To solve the problem, we need to find the RMS value of an alternating current (AC) that produces heat in a resistor, which is twice the heat produced by a steady current of 1.414 A in the same resistor.
1. Understand the Heat Produced by Current:
The heat produced (H) in a resistor by a current (I) over a time (T) is given by the formula:
H=I2RT
where R is the resistance.
2. Define the Two Scenarios:
- Let H1 be the heat produced by the AC current with RMS value IRMS.
- Let H2 be the heat produced by the steady current of 1.414A.
3. Set Up the Heat Equations:
For the steady current:
H2=(1.414)2RT
For the AC current:
H1=I2RMSRT
4. Relate the Two Heat Values:
According to the problem, the heat produced by the AC current is twice that produced by the steady current:
H1=2H2
Substituting the expressions for H1 and H2:
I2RMSRT=2×(1.414)2RT
5. Cancel Out Common Terms:
Since R and T are common in both sides, we can cancel them out:
I2RMS=2×(1.414)2
6. Calculate (1.414)2:
(1.414)2=2
Therefore, substituting this back in:
I2RMS=2×2=4
7. Find IRMS:
Taking the square root of both sides:
IRMS=√4=2A
Final Answer:
The RMS value of the AC current is 2A.
---
The heat produced (H) in a resistor by a current (I) over a time (T) is given by the formula:
H=I2RT
where R is the resistance.
- Let H1 be the heat produced by the AC current with RMS value IRMS.
- Let H2 be the heat produced by the steady current of 1.414A.
For the steady current:
H2=(1.414)2RT
For the AC current:
H1=I2RMSRT
According to the problem, the heat produced by the AC current is twice that produced by the steady current:
H1=2H2
Substituting the expressions for H1 and H2:
I2RMSRT=2×(1.414)2RT
Since R and T are common in both sides, we can cancel them out:
I2RMS=2×(1.414)2
(1.414)2=2
Therefore, substituting this back in:
I2RMS=2×2=4
Taking the square root of both sides:
IRMS=√4=2A
The RMS value of the AC current is 2A.
Similar Questions
What is the r.m.s. value of an alternating current which when passed through a resistor produces heat which is thrice of that produced by a direct current of 2 amperes in the same resistor?
View SolutionIf an AC produces same heat as that produced by a steady current of 4 A , then peak value of current is :
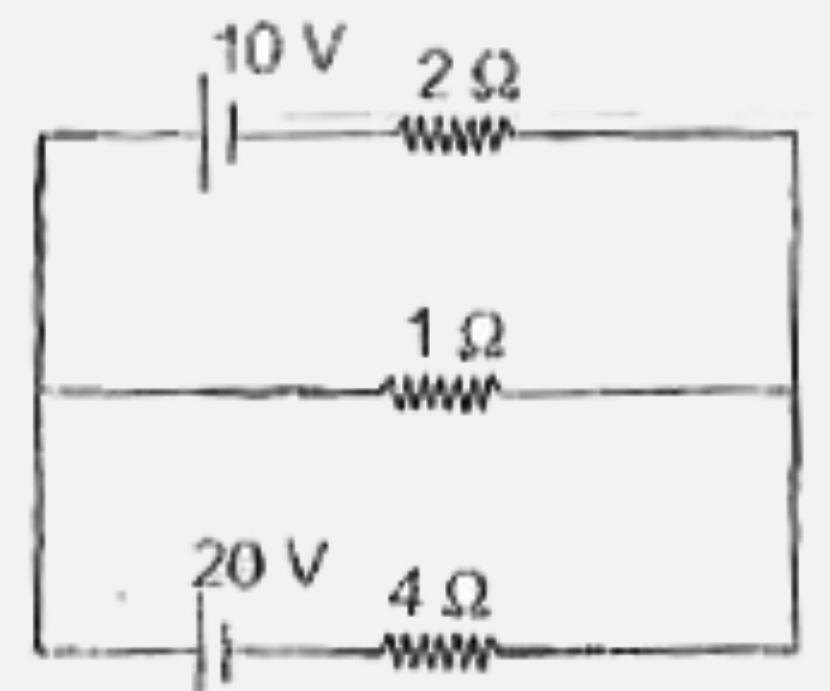
View SolutionThe value of current through 2Ω resistor is
View SolutionThe electric current passes through a metallic wire produces heat because of :
View SolutionWhat is the approximate peak value of an altemating currrent producing four times the heat produced per second by a steady current of 2 A in a resistor ?
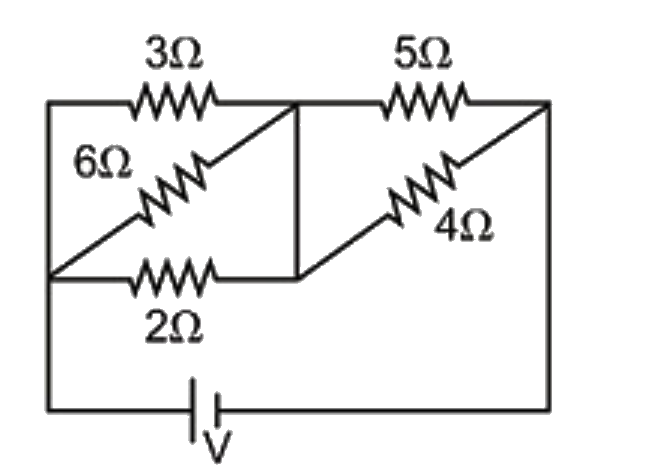
View SolutionThe resistor in which maximum heat will be produced is
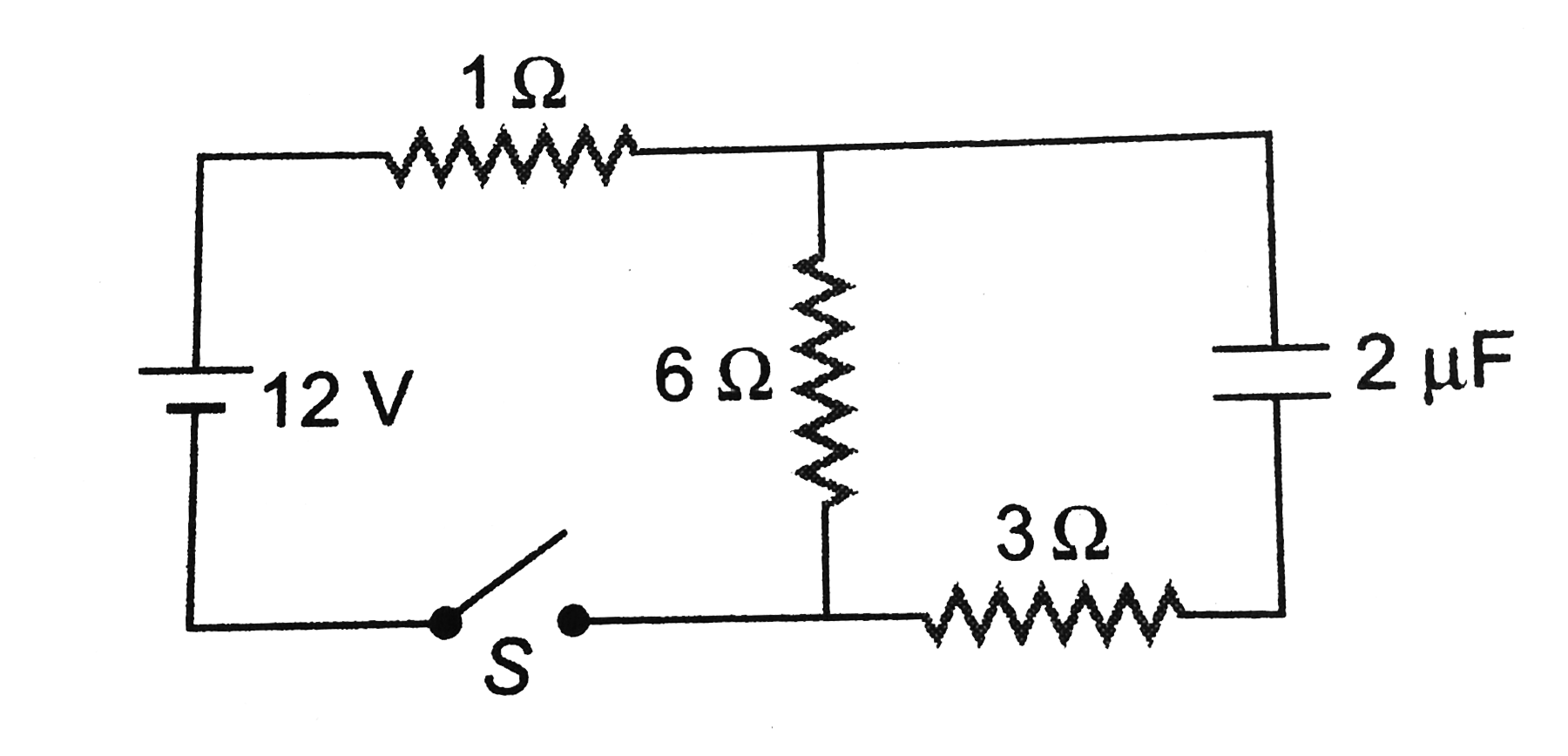
View SolutionWhen the switch is closed, the initial current through the 1Ω resistor is
View Solution10 kJ/s of heat is produced when a DC flows through a 100Ω resistor. Which of the following ac produces nearly same heat per sec through the same resistor ?
View SolutionWhen a current passes through a resistor, its temperature increases. Is it an adiabatic process?
View SolutionIn the figure given below, the current passing through 6Ω resistor is
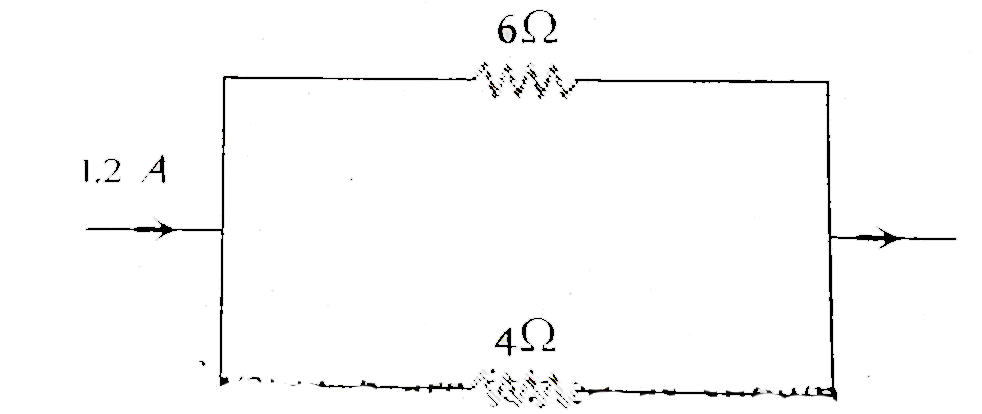
View Solution
