A spring of force constant k extends by a length X on loading . If T is the tension in the spring then the energy stored in the spring is
T22k
T22k2
2kT2
2T2k
The correct Answer is:A
To find the energy stored in a spring when it is extended by a length X under a tension T, we can follow these steps:
Step 1: Understand the formula for energy stored in a spring
The energy E stored in a spring is given by the formula:
E=12kx2
where k is the spring constant and x is the extension of the spring.
Step 2: Relate tension to extension
According to Hooke's Law, the tension T in the spring is directly proportional to the extension x:
T=kx
From this, we can express the extension x in terms of tension T:
x=Tk
Step 3: Substitute x in the energy formula
Now, we can substitute the expression for x into the energy formula:
E=12k(Tk)2
This simplifies to:
E=12k⋅T2k2
E=T22k
Conclusion
Thus, the energy stored in the spring when it is extended by a length X under a tension T is:
E=T22k
The energy E stored in a spring is given by the formula:
E=12kx2
where k is the spring constant and x is the extension of the spring.
According to Hooke's Law, the tension T in the spring is directly proportional to the extension x:
T=kx
From this, we can express the extension x in terms of tension T:
x=Tk
Now, we can substitute the expression for x into the energy formula:
E=12k(Tk)2
This simplifies to:
E=12k⋅T2k2
E=T22k
Thus, the energy stored in the spring when it is extended by a length X under a tension T is:
E=T22k
Similar Questions
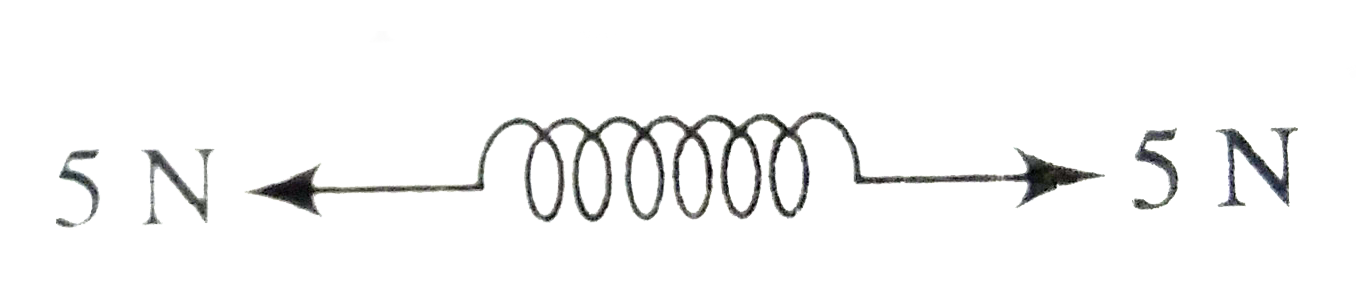
The tension is the spring is
View SolutionForce constants K1andK2 of two springs are in the ratio 5:4. They are stretched by same length. If potential energy stored in one spring is 25 J then potential energy stored in second spring is
View SolutionIn the figure shown, PQ is a uniform sphere of mass M and radius R hinged at point P , with PQ as horizontal diameter. QT is a uniform horizontal rod of mass M and length 2R rigidly attached to the sphere at Q, supported by a vertical spring of spring constant k. If the system is in equilibrium, then the potential energy stored in the spring is
View SolutionA block of mass m held touching the upper end of a light spring of force constant K as shown in figure. Find the maximum potential energy stored in the spring if the block is released suddenly on the spring.

View SolutionA block of mass m is initially moving to the right on a horizontal frictionless surface at a speed v. It then compresses a spring of spring constant k. At the instant when the kinetic energy of the block is equal to the potential energy of the spring, the spring is compressed a distance of :
View SolutionA spring of force constant k. is cut into lengths of ratio 1:2:3. They are connected in series and the new force constant is k'. Then they are connected in parallel and force constant is k''. Then K':K'' is
View SolutionA spring of force constant k is cut into lengths of ratio 1:2:3. They are connected in series and the new force constant is k'. Then they are connected in parallel and force constant is k'. Then k' : k" is :
View SolutionA force of 10 N holds an ideal spring with a 20Nm spring constant in compression. The potential energy stored in the spring is
View SolutionA long spring when stretched by xcm, has a potential energy U. On increasing the stretching to nxcm, the potential energy stored in spring will be
View SolutionIf a spring extends by x on loading, then the energy stored by the spring is (if T is tension in the spring and k is spring constant)
View Solution
