Question

Terminal velocity ${\displaystyle \left(V\right)}$ of a spherical object varies with a radius of object ${\displaystyle \left(r\right)}$ -

Options

A.
B.
C.
D.

Answer

A

Explanation

To determine how the terminal velocity $$V$$ of a spherical object varies with its radius $$r$$, we can start by using the formula for terminal velocity in a viscous fluid:

$$V=\frac{2}{9}\frac{g{r}^2(\sigma -\rho )}{\eta }$$

Where:
- $$V$$ is the terminal velocity,
- $$g$$ is the acceleration due to gravity,
- $$r$$ is the radius of the object,
- $$\sigma$$ is the density of the object,
- $$\rho$$ is the density of the fluid,
- $$\eta$$ is the coefficient of viscosity.

Step 1: Identify the constants
In the context of the problem, we can identify that \( g \), \( \sigma \), \( \rho \), and \( \eta \) are constants for a given scenario. Therefore, we can simplify our equation to focus on the relationship between \( V \) and \( r \).

Step 2: Simplify the equation
Since \( g \), \( \sigma \), \( \rho \), and \( \eta \) are constants, we can rewrite the equation as:

\( V = K r^2 \)

Where \( K = \frac{2}{9} \frac{g (\sigma - \rho)}{\eta} \).

Step 3: Analyze the relationship
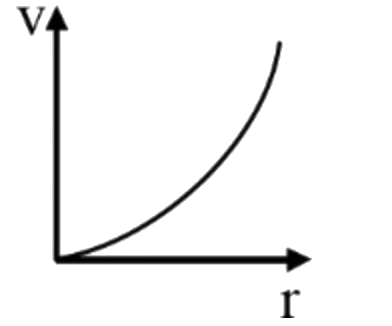
The equation \( V = K r^2 \) indicates that terminal velocity \( V \) is directly proportional to the square of the radius \( r \). This is a quadratic relationship.

Step 4: Graph the relationship
To graph this relationship, we note that it resembles the form \( y = ax^2 \), where \( y \) corresponds to \( V \) and \( x \) corresponds to \( r \). The graph of a quadratic function is a parabola that opens upwards.

Step 5: Identify the correct graph
From the options provided, we need to choose the graph that represents a quadratic relationship between \( V \) and \( r \). The correct graph will show a parabolic curve indicating that as the radius increases, the terminal velocity increases with the square of the radius.

Conclusion
Thus, the correct graph that represents the relationship between terminal velocity \( V \) and radius \( r \) is the one that shows a parabolic curve.

---