A rubber cord of density d, Young's modulus Y and length L is suspended vertically . If the cord extends by a length 0.5 L under its own weight , then L is
The correct Answer is:B
To solve the problem step by step, we will derive the expression for the length L of the rubber cord that extends by 0.5L under its own weight.
Step 1: Understand the Problem
We have a rubber cord of density d, Young's modulus Y, and length L suspended vertically. The cord extends by a length 0.5L under its own weight. We need to find the value of L.
Step 2: Define the Weight of the Cord
The weight of the cord can be expressed as:
W=mg
where m is the mass of the cord and g is the acceleration due to gravity. The mass m of the cord can be calculated as:
m=density×volume=d×(A×L)
where A is the cross-sectional area of the cord.
Step 3: Substitute the Mass into the Weight Equation
Substituting the expression for mass into the weight equation gives:
W=d⋅(A⋅L)⋅g=dAgL
Step 4: Use Young's Modulus to Relate Extension to Force
According to Young's modulus, the extension ΔL of the cord due to the force F (which is the weight of the cord) is given by:
ΔL=F⋅LA⋅Y
In our case, the force F is equal to the weight W:
ΔL=dAgL⋅LA⋅Y
Step 5: Simplify the Equation
The area A cancels out:
ΔL=dgL2Y
Step 6: Set the Extension Equal to 0.5L
We know that the extension ΔL is equal to 0.5L:
0.5L=dgL2Y
Step 7: Rearranging the Equation
Rearranging the equation gives:
L=2Ydg
Final Result
Thus, the length L of the rubber cord is:
L=2Ydg
We have a rubber cord of density d, Young's modulus Y, and length L suspended vertically. The cord extends by a length 0.5L under its own weight. We need to find the value of L.
The weight of the cord can be expressed as:
W=mg
where m is the mass of the cord and g is the acceleration due to gravity. The mass m of the cord can be calculated as:
m=density×volume=d×(A×L)
where A is the cross-sectional area of the cord.
Substituting the expression for mass into the weight equation gives:
W=d⋅(A⋅L)⋅g=dAgL
According to Young's modulus, the extension ΔL of the cord due to the force F (which is the weight of the cord) is given by:
ΔL=F⋅LA⋅Y
In our case, the force F is equal to the weight W:
ΔL=dAgL⋅LA⋅Y
The area A cancels out:
ΔL=dgL2Y
We know that the extension ΔL is equal to 0.5L:
0.5L=dgL2Y
Rearranging the equation gives:
L=2Ydg
Thus, the length L of the rubber cord is:
L=2Ydg
Similar Questions
An indian ruber cord L meter long and area of cross-secion A metre is suspended vertically. Density of rubber is ρ kg/ metre3 and Young's modulus of rubber is Y newton/ metre2. IF the cord extends by l metre under its own. Weight, then extension l is
View Solutionwire of length I has a ear mass density λ and area of cross-section A and the Young's modulus y is suspended vertically from rigid support. The extension produced in wire due to its own weight
View Solution
Knowledge Check
An indian ruber cord L meter long and area of cross-secion A metre is suspended vertically. Density of rubber is ρ kg/ metre3 and Young's modulus of rubber is Y newton/ metre2. IF the cord extends by l metre under its own. Weight, then extension l is
AL2ρg/YBL2ρg/2yCL2)ρg/4YDY/L2ρg.
A rubber cord 10m long is suspended vertically. How much does it stretch under its own weight (Density of rubber is 1500kg/m,Y=5×10N/m,g=10m/s)
View SolutionA thick rope of density ρ and length L is hung from a rigid support. The increase in length of the rope due to its own weight is (Y is the Young's modulus)
View SolutionA rubber pipe of density 1.5×103N/m2 and Young's modulus 5×106N/m2 is suspended from the roof. The length of the pipe is 8m. What will be the change in length due to its own weight.
View SolutionA wire of length l and cross-sectional are A is suspended at one of its ends from a ceiling. What will be its strain energy due to its own weight, if the density and Young's modulus of the material of the wire be d and Y?
View SolutionA copper rod length L and radius r is suspended from the ceiling by one of its ends. What will be elongation of the rod due to its own weight when p andYare the density and Young's modulus of the copper respectively?
View SolutionA wire of natural length l, young's modulus Y and area of cross-section A is extended by x. Then, the energy stored in the wire is given by
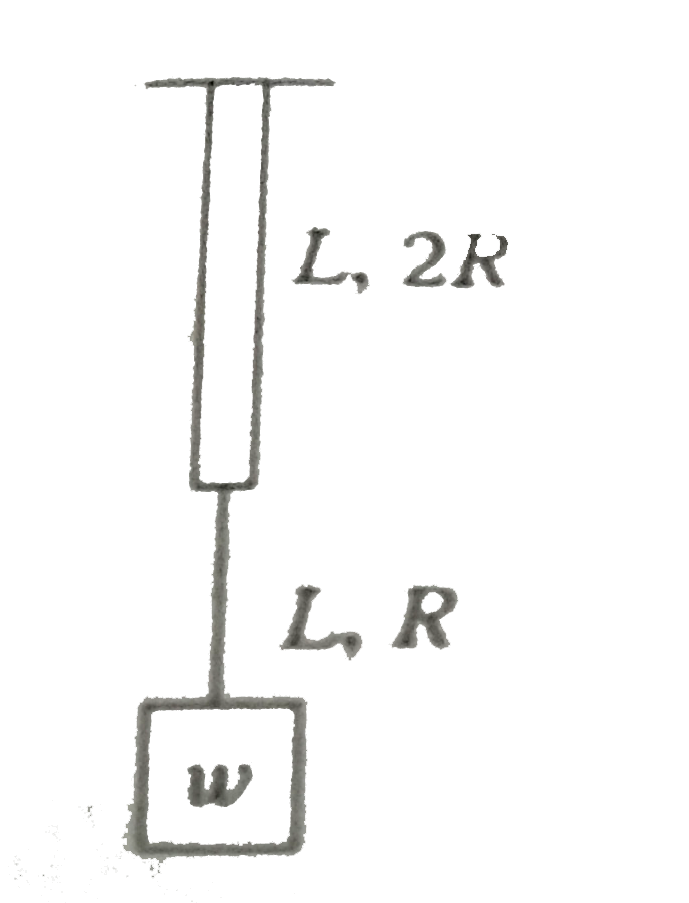
View SolutionTwo wires of the same material (Young's modulus=Y) and same length L but radii R and 2R respectively are joined end to end and a weight w is suspended from the combination as shown in the figure. The elastic potential energy in the system is
View Solution