Minimum thickness of a mica sheet having μ=32 which should be placed in front of one of the slits in YDSE is required to reduce the intensity at the centre of screen to half of maximum intensity is
λ4
λ8
λ2
λ3
The correct Answer is:C
To solve the problem of finding the minimum thickness of a mica sheet required to reduce the intensity at the center of the screen in a Young's Double Slit Experiment (YDSE) to half of the maximum intensity, we can follow these steps:
1. Understanding Maximum Intensity:
In YDSE, the maximum intensity Imax at the center of the screen is given by:
Imax=4I
where I is the intensity from each slit.
2. Finding Half of Maximum Intensity:
To find the intensity that is half of the maximum intensity:
Ihalf=Imax2=4I2=2I
3. Phase Difference Calculation:
When a mica sheet of thickness t and refractive index μ=32 is placed in front of one of the slits, it introduces a path difference Δx:
Δx=(μ−1)t=(32−1)t=12t
4. Phase Difference Relation:
The phase difference ϕ due to this path difference is given by:
ϕ=2πλΔx=2πλ(12t)=πtλ
5. Setting Up the Intensity Equation:
The net intensity Inet at the center of the screen can be expressed as:
Inet=I1+I2+2√I1I2cosϕ
Since I1=I2=I:
Inet=I+I+2Icosϕ=2I+2Icosϕ=2I(1+cosϕ)
6. Setting the Intensity to Half Maximum:
We want Inet=2I:
2I(1+cosϕ)=2I
Dividing both sides by 2I (assuming I≠0):
1+cosϕ=1⟹cosϕ=0
7. Finding the Corresponding Phase Difference:
The condition cosϕ=0 occurs when:
ϕ=π2+nπ(n∈Z)
For minimum thickness, we take n=0:
ϕ=π2
8. Substituting Phase Difference into the Equation:
Now substituting ϕ=π2 into the phase difference equation:
πtλ=π2
9. Solving for Thickness:
Canceling π from both sides:
tλ=12⟹t=λ2
Final Answer:
The minimum thickness of the mica sheet required is:
t=λ2
In YDSE, the maximum intensity Imax at the center of the screen is given by:
Imax=4I
where I is the intensity from each slit.
To find the intensity that is half of the maximum intensity:
Ihalf=Imax2=4I2=2I
When a mica sheet of thickness t and refractive index μ=32 is placed in front of one of the slits, it introduces a path difference Δx:
Δx=(μ−1)t=(32−1)t=12t
The phase difference ϕ due to this path difference is given by:
ϕ=2πλΔx=2πλ(12t)=πtλ
The net intensity Inet at the center of the screen can be expressed as:
Inet=I1+I2+2√I1I2cosϕ
Since I1=I2=I:
Inet=I+I+2Icosϕ=2I+2Icosϕ=2I(1+cosϕ)
We want Inet=2I:
2I(1+cosϕ)=2I
Dividing both sides by 2I (assuming I≠0):
1+cosϕ=1⟹cosϕ=0
The condition cosϕ=0 occurs when:
ϕ=π2+nπ(n∈Z)
For minimum thickness, we take n=0:
ϕ=π2
Now substituting ϕ=π2 into the phase difference equation:
πtλ=π2
Canceling π from both sides:
tλ=12⟹t=λ2
The minimum thickness of the mica sheet required is:
t=λ2
Similar Questions
A thin glass plate of thickness t and refractive index μ is inserted between screen and one of the slits in a young's experiment. If the intensity at the centre of the screen is I, what was the intensity at the same point prior to the introduction of the sheet.
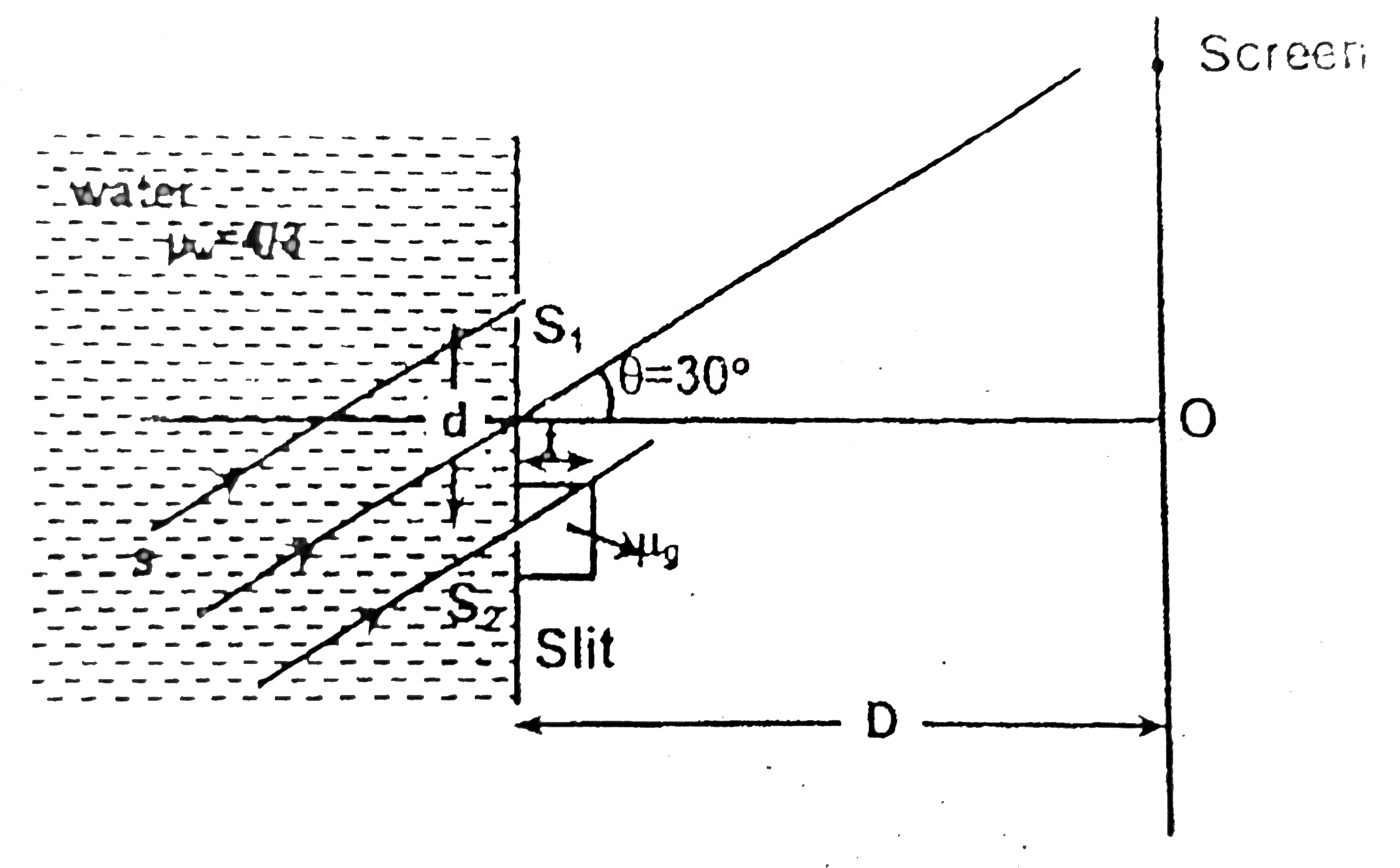
View SolutionIn a YDSE experiment the two slits are covered with a transparent membrane of negligible thickness which allows light to pass through it but does not allow water. A glass slab of thickness t=0.41mm and refractive index μg=1.5 is placed infront of one of the slits as shown in the figure. The separation between the slits is d=0.30mm. The entire space to the left of the slits is filled with water of refractive index μw=4/3.
A coherent light of intensity I and absolute wavelength λ=5000Å is being incident on the slits making an angle 30∘ with horizontal. If screen is placed at a distance D=1m from the slits, find
(a) the position of central maxima.
(b) the intensity at point O.
View SolutionA monochromatic light of λ=500nm is incident on two identical slits separated by a distance of 5×10−4m. The interference pattern is seen on a screen placed at a distance of 1m from the plane of slits. A thin glass plate of thickness 1.5×10−6m and refractive index μ=1.5 is placed between one of the slits and the screen. Find the intensity at the center of the screen if the intensity is I0 in the absence of the plate. Also find the lateral shift of the central maxima and number of fringes crossed through center.
View SolutionIn young's double-slit experiment, both the slits produce equal intensities on a screen. A 100% transparent thin film of refractive index μ=1.5 is kept in front of one of the slits, due to which the intensity at the point O on the screen becomes 75% of its initial value. If the wavelength of monochromatic light is 720 nm, then what is the minimum thickness (in nm) of the film?
View SolutionA transparent glass plate of thickness 0.5 mm and refractive index 1.5 is placed infront of one of the slits in a double slit experiment. If the wavelength of light used is 6000A∘, the ratio of maximum to minimum intensity in the interference pattern is 25/4. Then the ratio of light intensity transmitted to incident on thin transparent glass plate is
View SolutionIn YDSE, both slits produce equal intensities on the screen. A 100% transparent thin film is placed in front of one of the slits. Now, the intensity on the centre becomes 75% of the previous intensity. The wavelength of light is 6000Å and refractive index of glass is 1.5. Thus, minimum thickness of the glass slab is
View SolutionIn YDSE if a slab whose refractive index can be varied is placed in front of one of the slits. Then, the variation of resultant intensity at mid-point of screen with μ will be best represented by (mu is greater than or equal to 1)
View SolutionIn YDSE if a slab whose refractive index can be varied is placed in front of one of the slits. Then, the variation of resultant intensity at mid-point of screen with μ will be best represented by (mu is greater than or equal to 1)
View SolutionA plate of thickness t made of a material of refractive index μ is placed in front of one of the slits in a double slit experiment. (a) Find the changes in he optical path due to introduction of the plate. (b) Wht should be the minimum thickness t which will make the intensity at the centre of the fringe pattern zero ? Wavelength of the light used is λ. Neglect any absorption of light in the plate.
View SolutionIn YDSE, find the thickness of a glass slab (μ=1.5) which
should be placed in front of the upper slit S1 so that the central maximum now
lies at a point where 5th bright fringe was lying earlier (before inserting the
slab). Wavelength of light used is 5000Å.
View Solution
