A circular loop with N turns has radius r . It lies in the x-y plane carrying current I in the anti-clockwise direction . If the magnetic field in the region is →B=B0ˆi , then find the torque (→r) acting on the loop .
B0NIπr2ˆj
B0Iπr2ˆj
B0NIπr2ˆk
B0πr2atk
The correct Answer is:A
To find the torque acting on a circular loop carrying current in a magnetic field, we can follow these steps:
Step 1: Determine the Magnetic Moment (M)
The magnetic moment →M of a circular loop is given by the formula:
→M=N⋅I⋅A⋅^j
where:
- N is the number of turns,
- I is the current,
- A is the area of the loop,
- ^j indicates the direction of the magnetic moment (upward for anti-clockwise current).
The area A of a circular loop is:
A=πr2
Thus, we can write:
→M=N⋅I⋅πr2⋅^j
Step 2: Identify the Magnetic Field (B)
The magnetic field in the region is given as:
→B=B0^i
Step 3: Calculate the Torque (τ)
The torque →τ acting on the loop is given by the cross product of the magnetic moment and the magnetic field:
→τ=→M×→B
Substituting the expressions for →M and →B:
→τ=(N⋅I⋅πr2⋅^j)×(B0^i)
Step 4: Evaluate the Cross Product
Using the right-hand rule and the properties of cross products:
^j×^i=−^k
Thus,
→τ=N⋅I⋅πr2⋅B0⋅(−^k)
This simplifies to:
→τ=−N⋅I⋅πr2⋅B0^k
Final Result
The torque acting on the loop is:
→τ=−N⋅I⋅πr2⋅B0^k
The magnetic moment →M of a circular loop is given by the formula:
→M=N⋅I⋅A⋅^j
where:
- N is the number of turns,
- I is the current,
- A is the area of the loop,
- ^j indicates the direction of the magnetic moment (upward for anti-clockwise current).
A=πr2
Thus, we can write:
→M=N⋅I⋅πr2⋅^j
The magnetic field in the region is given as:
→B=B0^i
The torque →τ acting on the loop is given by the cross product of the magnetic moment and the magnetic field:
→τ=→M×→B
→τ=(N⋅I⋅πr2⋅^j)×(B0^i)
Using the right-hand rule and the properties of cross products:
^j×^i=−^k
Thus,
→τ=N⋅I⋅πr2⋅B0⋅(−^k)
This simplifies to:
→τ=−N⋅I⋅πr2⋅B0^k
The torque acting on the loop is:
→τ=−N⋅I⋅πr2⋅B0^k
Similar Questions
In figure is shown a circular loop carrying current I. Show the direction of the magnetic field with the help of lines of force.
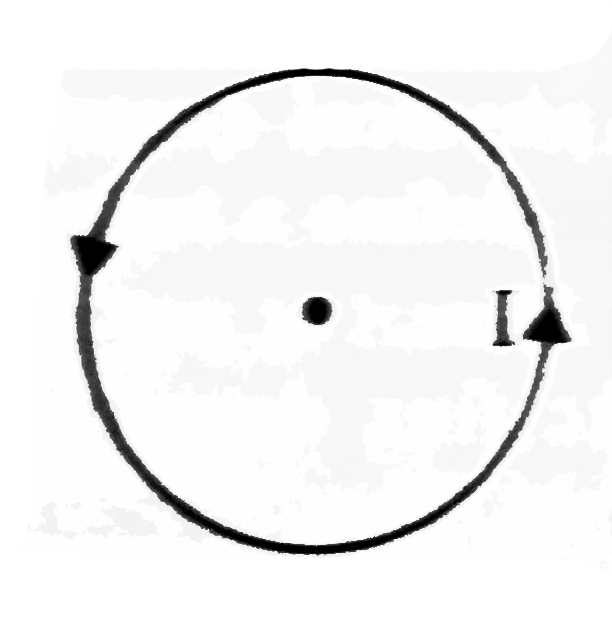
View SolutionA wire loop carrying current I is placed in the x−y plane as shown in the figure. If an external uniform magnetic field →B=Bˆi is switched on, then the torque acting on the loop is
View SolutionA circular loop of radius 4.0 cm is placed in a horizontal plane and carries an electric current of 5.0 A in the clockwise direction as seen from above. Find the magnetic field (a) at a point 3.0 cm above the centre of the loop (b) at a point 3.0 cm below the centre of the loop.
View SolutionA circular coil having N turns and radius r carries a current I. It is held in the XZ plane in a magnetic field Bˆi. The torque on the coil due to the magnetic field is :
View SolutionA circular coil having N turns and radius r carries a current I. It is held in the XZ plane in a magnetic field Bˆi. The torque on the coil due to the magnetic field is :
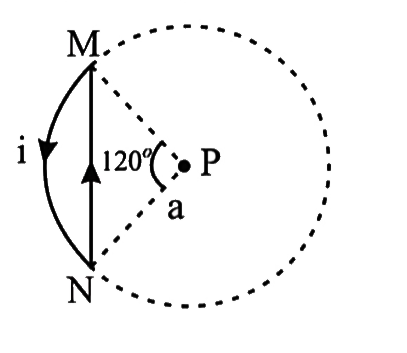
View SolutionA loop, carring a current i, lying in the plane of the paper, is in the field of a long straight wire with current i0 (inward) as shown in Fig. Find the torque acting on the loop.

View SolutionA circular loop of one turn carries a current of 5.00 A. If the magnetic field B at the centre is 0.200 mT, find the radius of the loop.
View SolutionA current carrying circular coil is bent so as to convert it into a double loop, both the loops being concentric and are carrying current in the same direction. If B is the initial magnetic field at the centre, the final magnetic field at the centre will be
View SolutionA circular coil of n turns and radius r carries a current I. The magnetic field at the centre is
View SolutionA circular loop of radius a, carrying a current I, is placed in a tow dimensional magnetic field. The centre of the loop coincides with the centre of the filed The strenght of the magnetic field at the pariphery of the loop is B. find the magnetic force on the wire.

View Solution
