A wire in the from of a square of side 'a' carries a current i. Then the magnetic induction at the centre of the square wire is (Magnetic permeability of free space=μ0)
μ0i2πa
μ0i√2πa
2√2μ0iπa
μ0i√2πa
The correct Answer is:C
To find the magnetic induction (magnetic field) at the center of a square wire carrying current, we can follow these steps:
1. Understanding the Configuration:
- We have a square wire of side length a carrying a current i.
- We need to calculate the magnetic field at the center O of the square.
2. Magnetic Field due to a Straight Wire:
- The magnetic field B at a distance R from a long straight wire carrying current I is given by the formula:
B=μ0I2πR
- Here, μ0 is the permeability of free space.
3. Distance from the Center to the Wire:
- The distance R from the center O to the midpoint of each side of the square is a2.
4. Calculating the Magnetic Field from One Side:
- For one side of the square, we can substitute R=a2 into the magnetic field formula:
Bone side=μ0i2π(a2)=μ0iπa
5. Considering the Contribution from All Four Sides:
- Since the square has four sides and the magnetic field contributions from each side will have the same magnitude but different directions, we need to consider the angles.
- The angle θ between the wire and the line connecting the wire to the center O is 45∘ for each side.
6. Using the Sine Component:
- The effective magnetic field contribution from each side at the center is:
Beffective=Bone side⋅sin(45∘)=μ0iπa⋅1√2=μ0i√2πa
7. Total Magnetic Field at the Center:
- Since there are four sides contributing equally, the total magnetic field Btotal at the center is:
Btotal=4⋅Beffective=4⋅μ0i√2πa=4μ0i√2πa=2√2μ0iπa
Final Result:
The magnetic induction at the center of the square wire is:
B=2√2μ0iπa
- We have a square wire of side length a carrying a current i.
- We need to calculate the magnetic field at the center O of the square.
- The magnetic field B at a distance R from a long straight wire carrying current I is given by the formula:
B=μ0I2πR
- Here, μ0 is the permeability of free space.
- The distance R from the center O to the midpoint of each side of the square is a2.
- For one side of the square, we can substitute R=a2 into the magnetic field formula:
Bone side=μ0i2π(a2)=μ0iπa
- Since the square has four sides and the magnetic field contributions from each side will have the same magnitude but different directions, we need to consider the angles.
- The angle θ between the wire and the line connecting the wire to the center O is 45∘ for each side.
- The effective magnetic field contribution from each side at the center is:
Beffective=Bone side⋅sin(45∘)=μ0iπa⋅1√2=μ0i√2πa
- Since there are four sides contributing equally, the total magnetic field Btotal at the center is:
Btotal=4⋅Beffective=4⋅μ0i√2πa=4μ0i√2πa=2√2μ0iπa
The magnetic induction at the center of the square wire is:
B=2√2μ0iπa
Similar Questions
A square loop of side a carris a current I. The magnetic field at the centre of the loop is
View SolutionThe magnetic field at the centre of square of side a is
 View Solution
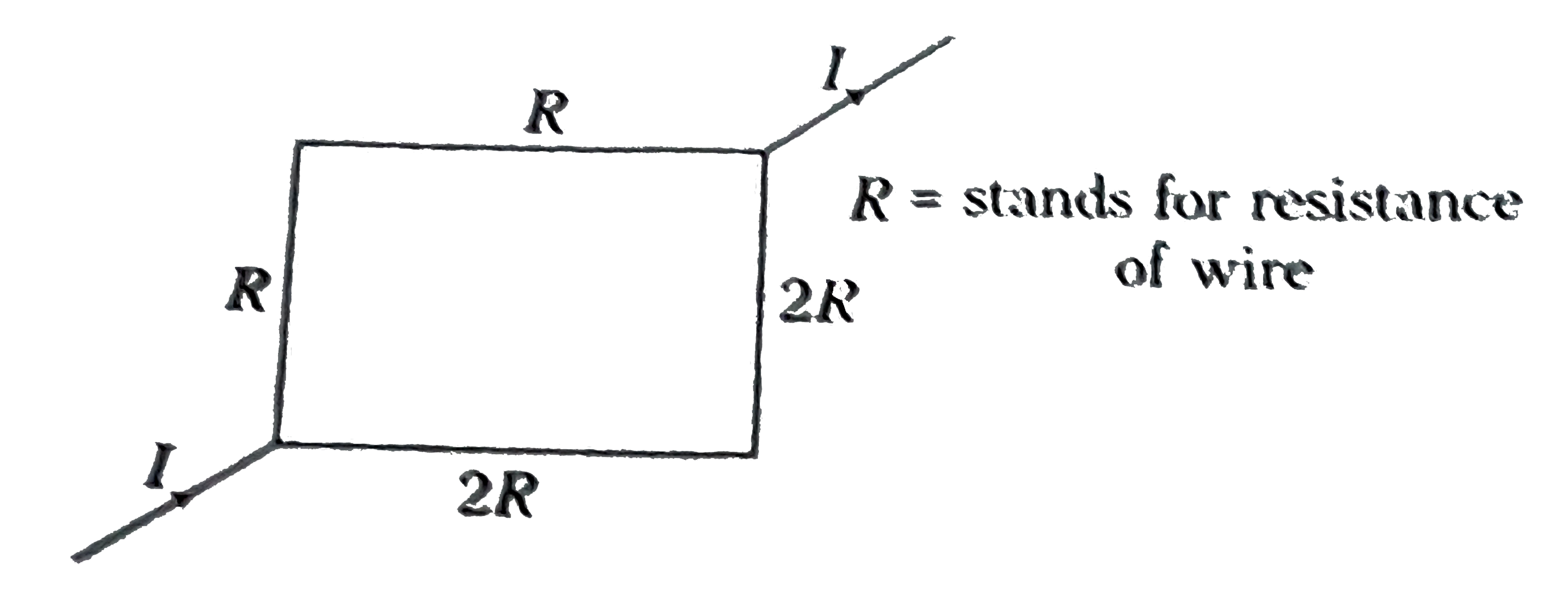
View SolutionThe magnetic induction at the point O, if the wire carries a current I, is
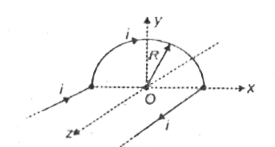 View Solution
View SolutionA square conducting loop of side length L carries a current I.The magnetic field at the centre of the loop is
View SolutionA square conducting loop of side length L carries a current I.The magnetic field at the centre of the loop is (dependence on L)
View SolutionThe dimensional formula for permeability of free space, μ0 is
View SolutionA wire of length l is bent in the form of an equilateral triangle and carries an electric current i. (a) Find the magnetic field B at the centre. (b) If the wire is bent in the form of a square, what would be the value of B at the centre?
View SolutionA rectangular loop of metallic wire is of length a and breadth b and carries a current i. The magnetic field at the centre of the loop is
View SolutionCurrent 'i' is flowing in heaxagonal coil of side a. The magnetic induction at the centre of the coil will be
View SolutionTwo identical wires AandB , each of length 'l', carry the same current I. Wire A is bent into a circle of radius RandwireB is bent to form a square of side 'a' . If BAandBB are the values of magnetic field at the centres of the circle and square respectively , then the ratio BABB is :
View Solution

