A stone is projected from the ground with velocity 50ms at an angle of 30∘. It crosses a wall after 3 sec. How far beyond the wall the stone will strike the ground (g=10msec2?
90.2 m
12.5 m
86.5 m
216.25 m
The correct Answer is:C
To solve the problem step by step, we will follow the principles of projectile motion.
Step 1: Identify the components of the initial velocity
The stone is projected with an initial velocity u=50m/s at an angle θ=30∘.
The horizontal and vertical components of the initial velocity can be calculated as:
- Horizontal component ux=ucosθ=50cos(30∘)
- Vertical component uy=usinθ=50sin(30∘)
Using the values:
- cos(30∘)=√32
- sin(30∘)=12
Calculating:
- ux=50⋅√32=25√3m/s
- uy=50⋅12=25m/s
Step 2: Calculate the total time of flight
The total time of flight T for a projectile is given by:
T=2uyg
where g=10m/s2.
Substituting the values:
T=2⋅2510=5s
Step 3: Determine the time in the air after crossing the wall
The stone crosses the wall after t=3s. Therefore, the time remaining in the air after crossing the wall is:
tremaining=T−t=5−3=2s
Step 4: Calculate the horizontal distance traveled after crossing the wall
The horizontal distance d traveled after crossing the wall can be calculated using the horizontal component of the velocity and the remaining time:
d=ux⋅tremaining
Substituting the values:
d=25√3⋅2=50√3m
Step 5: Calculate the numerical value of the distance
Using √3≈1.732:
d≈50⋅1.732≈86.6m
Final Answer
The stone will strike the ground approximately 86.6 meters beyond the wall.
---
The stone is projected with an initial velocity u=50m/s at an angle θ=30∘.
- Horizontal component ux=ucosθ=50cos(30∘)
- Vertical component uy=usinθ=50sin(30∘)
- cos(30∘)=√32
- sin(30∘)=12
- ux=50⋅√32=25√3m/s
- uy=50⋅12=25m/s
The total time of flight T for a projectile is given by:
T=2uyg
where g=10m/s2.
T=2⋅2510=5s
The stone crosses the wall after t=3s. Therefore, the time remaining in the air after crossing the wall is:
tremaining=T−t=5−3=2s
The horizontal distance d traveled after crossing the wall can be calculated using the horizontal component of the velocity and the remaining time:
d=ux⋅tremaining
Substituting the values:
d=25√3⋅2=50√3m
Using √3≈1.732:
d≈50⋅1.732≈86.6m
The stone will strike the ground approximately 86.6 meters beyond the wall.
Similar Questions
A stone is projected from the ground with a velocity of 20 m/s at angle 30∘ with the horizontal. After one second it clears a wall then find height of the wall. (g=10ms−2)
View SolutionA stone is projected vertically up from the ground with velocity 40ms−1 . The interval of time between the two instants at which the stone is at a height of 60 m above the ground is (g=10ms−2)
View SolutionA stone is projected from the ground with a velocity of 14ms−1. One second later it clears a wall 2m high. The angle of projection is (g=10ms−2)
View SolutionA person, standing on the roof of a 40 m high tower, throws a ball vertically upwards with speed 10 m/s. Two seconds later, he throws another ball again in vertical direction (use g=10ms2). Both the balls hit the ground simultaneously.
View SolutionA ball is projected from a point O as shown in figure it will strike the ground after (g = 10 m/s2 )
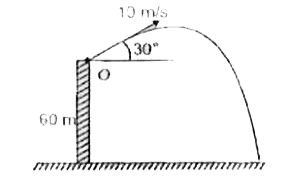
View SolutionThe surface of a hill is inclined at an angle α to the horizontal .A stone is thrown from the summit of the hill at an angle β to the vertical with velocity v0.How far from the summit will the stone strike the ground ?
View SolutionA helicopter while flying at a height of 100 m with velocity 30 m.s at an angle 30∘ with the horizontal, drops a packet. Where will the packet strike the ground ? ( g = 10 m/s2)
View Solution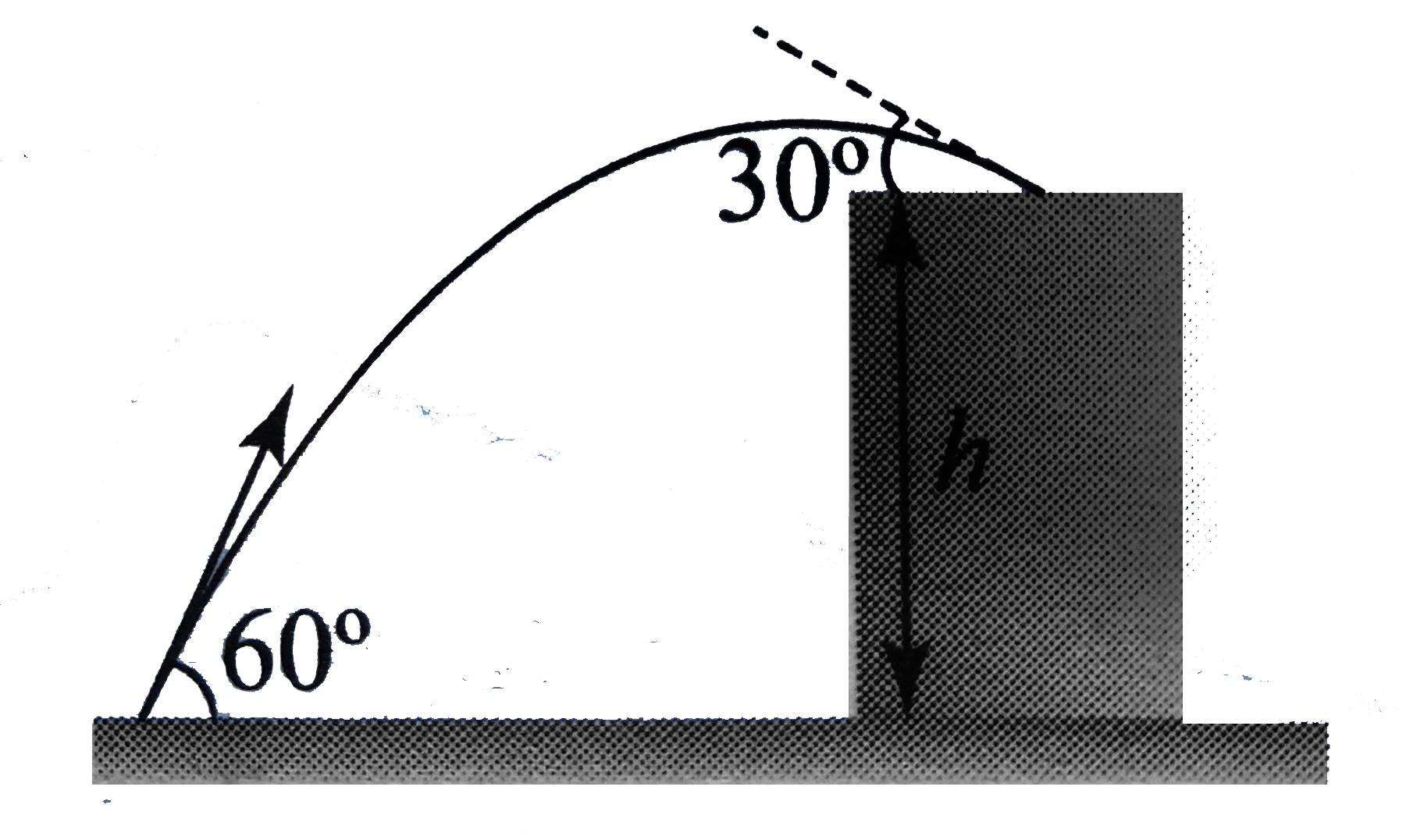
A stone projected at an angle of 60∘ from the ground level strikes at an angle of 30∘ on the roof of a building of height h. Then the speed of projection of the stone is:
View Solution
A stone projected at an angle of 60∘ from the ground level strikes at an angle of 30∘ on the roof of a building of height h. Then the speed of projection of the stone is:
View SolutionA particle A is projected from the ground with an initial velocity of 10m/s at an angle of 60∘ with horizontal. From what height should an another particle B be projected horizontally with velocity 5m/s so that both the particles collide in ground at point C if both are projected simultaneously g=10m/s2.

View Solution
