A suitcase is gently dropped on a conveyor belt moving at 3m/s. If the coefficient of friction between the belt and the suitcase is 0.5. Find the displacement of suitcase relative to conveyor belt before the slipping between the two is stopped (g=10m/s2)
2.7 m
1.8 m
0.9 m
1.2 m
The correct Answer is:C
To solve the problem step by step, we will analyze the motion of the suitcase on the conveyor belt and apply the principles of physics, particularly the concepts of friction and kinematics.
Step 1: Identify the Given Data
- Initial velocity of the suitcase, u=3m/s (the same as the conveyor belt)
- Coefficient of friction, μ=0.5
- Acceleration due to gravity, g=10m/s2
Step 2: Calculate the Maximum Static Friction
The maximum static friction force can be calculated using the formula:
fmax=μ⋅m⋅g
However, we will need the acceleration due to friction to find the displacement. The frictional force will cause a deceleration (retardation) of the suitcase.
Step 3: Calculate the Acceleration Due to Friction
The acceleration (retardation) due to friction can be calculated as:
a=−μg
Substituting the values:
a=−0.5⋅10=−5m/s2
Step 4: Use Kinematic Equation to Find Displacement
We will use the kinematic equation that relates initial velocity, final velocity, acceleration, and displacement:
v2=u2+2as
Where:
- v=0m/s (final velocity when slipping stops)
- u=3m/s (initial velocity)
- a=−5m/s2 (acceleration)
Rearranging the equation to solve for displacement s:
0=(3)2+2(−5)s
0=9−10s
10s=9
s=910=0.9m
Step 5: Conclusion
The displacement of the suitcase relative to the conveyor belt before slipping stops is 0.9m.
---
- Initial velocity of the suitcase, u=3m/s (the same as the conveyor belt)
- Coefficient of friction, μ=0.5
- Acceleration due to gravity, g=10m/s2
The maximum static friction force can be calculated using the formula:
fmax=μ⋅m⋅g
However, we will need the acceleration due to friction to find the displacement. The frictional force will cause a deceleration (retardation) of the suitcase.
The acceleration (retardation) due to friction can be calculated as:
a=−μg
Substituting the values:
a=−0.5⋅10=−5m/s2
We will use the kinematic equation that relates initial velocity, final velocity, acceleration, and displacement:
v2=u2+2as
Where:
- v=0m/s (final velocity when slipping stops)
- u=3m/s (initial velocity)
- a=−5m/s2 (acceleration)
0=(3)2+2(−5)s
0=9−10s
10s=9
s=910=0.9m
The displacement of the suitcase relative to the conveyor belt before slipping stops is 0.9m.
Similar Questions
An ice skater moving at 10m/s comes to a halt in 100m on a ice surface. Calculate the coefficient of friction between the ice and the skater ?
View SolutionA conveyor belt is moving at a constant speed of 2m/s . A box is gently dropped on it. The coefficient of friction between them is μ=0.5 . The distance that the box will move relative to belt before coming to rest on it taking g=10ms−2 is:
View SolutionGravel is dropped on a conveyor belt at the rate of 2 kg/s. The extra force required to keep the belt moving at 3ms−1 is
View Solution
A solid sphere of mass m and radius R is gently placed on a conveyer belt moving with constant velocity v0. If coefficient of friction between belt and sphere is 2/7 the distance traveled by the centre of the sphere before it starts pure rolling is
View SolutionA package rest on a conveyor belt which is initially at rest . The belt is started and moves to the right for 1.3s with a constant acceleration of 2m/s2. The belt then moves with a constant deceleration a2 and comes to a stop after a total displacement of 2.2m . Knowing that the coefficient of friction between the package and the belt are μs=0.35 and μk=0.25 , determine (a) the deceleration a2 of the belt , (b) the displacement of the package relative to the belt as the belt comes to a stop. (g=9.8m/s2)

View SolutionA force of 200N is applied as shown in the figure on block of 3 kg. The coefficient of friction between the block and the wall is 0.3. Find the friction acting on the block. (in N) {Take g = 10 m/s2

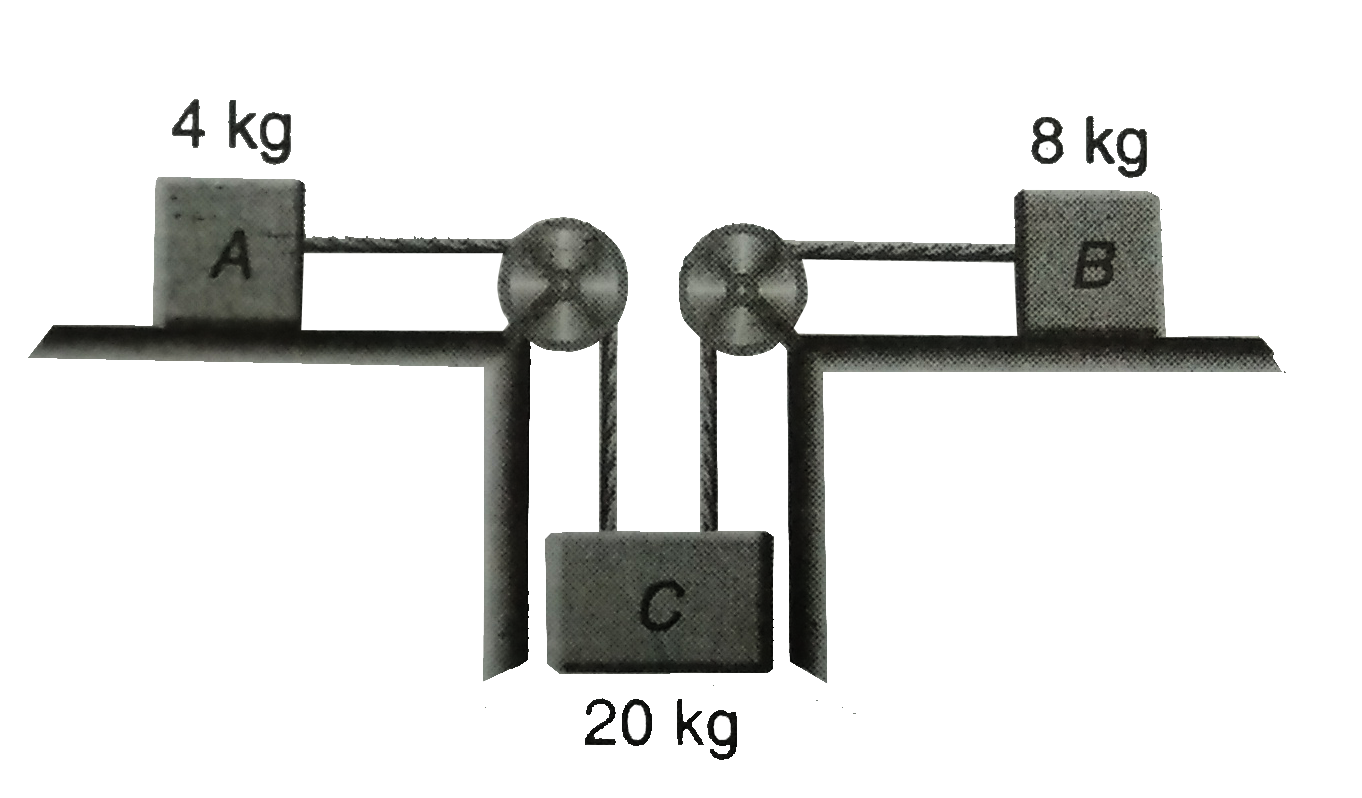
View SolutionConsider the situation shown in figure. The block B moves on a frictionless surface ,while the coefficient of friction between A and the surface on which it moves is 0.2. Find the acceleration with which the masses move and also the tension in the strings. (Takeg=10m/s2).
View SolutionGravels are dropped on a conveyor belt at the rate of 0.5 kg / sec . The extra force required in newtons to keep the belt moving at 2 m/sec is
View SolutionFigure shown a man standing stationary with respect to a horizontal converyor belt that is accelerationg with 1m/s−2 . What is the net force on the man?If the coefficient of ststic friction between the man's shoes and the belt is 0.2 upto what maximum acceleration of the belt can the man continue to be stationary relative to the belt? Mass of the man=65kg(g=9.8m/s2)
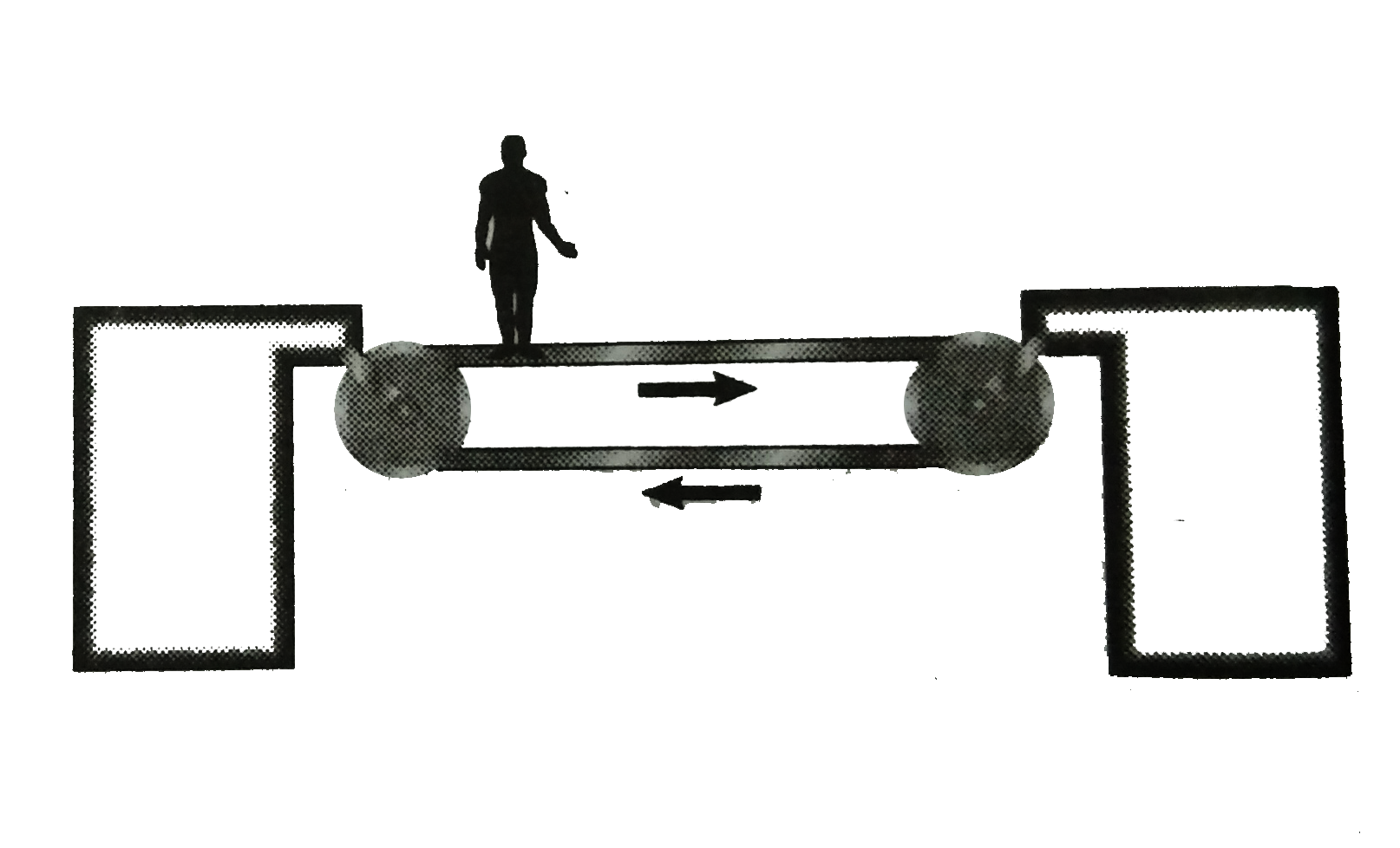
View SolutionFigure shown a man standing stationary with respect to a horizontal converyor belt that is accelerationg with 1m/s−2 . What is the net force on the man?If the coefficient of ststic friction between the man's shoes and the belt is 0.2 upto what maximum acceleration of the belt can the man continue to be stationary relative to the belt? Mass of the man=65kg(g=9.8m/s2)

View Solution
