A conveyor belt is moving at a constant speed of 2m/s . A box is gently dropped on it. The coefficient of friction between them is μ=0.5 . The distance that the box will move relative to belt before coming to rest on it taking g=10ms−2 is:
0.4m
1.2m
0.6m
0
The correct Answer is:A
To solve the problem, we need to determine the distance that the box will move relative to the conveyor belt before coming to rest. Here’s a step-by-step breakdown of the solution:
Step 1: Understand the Problem
The box is dropped onto a conveyor belt that is moving at a constant speed of 2 m/s. The coefficient of friction (μ) between the box and the belt is 0.5. We need to find out how far the box will slide on the belt before it stops relative to the belt.
Step 2: Identify Initial Conditions
- Initial speed of the box (u) = 2 m/s (since it was dropped onto the moving belt).
- Final speed of the box (v) = 0 m/s (when it comes to rest relative to the belt).
- Coefficient of friction (μ) = 0.5.
- Acceleration due to gravity (g) = 10 m/s².
Step 3: Calculate the Frictional Force
The frictional force (f) acting on the box can be calculated using:
f=μ⋅m⋅g
where m is the mass of the box. However, since we are looking for acceleration, we can express it as:
f=μ⋅m⋅g
Step 4: Calculate the Acceleration
The acceleration (a) of the box due to friction can be found using Newton's second law:
a=fm=μ⋅m⋅gm=μ⋅g
Substituting the values:
a=0.5⋅10=5m/s2
This acceleration will act in the opposite direction to the motion of the box (deceleration).
Step 5: Use the Kinematic Equation
We can use the kinematic equation to find the distance (s) the box moves relative to the belt before coming to rest:
v2=u2+2as
Substituting the known values:
- v=0
- u=2m/s
- a=−5m/s2 (negative because it's deceleration)
The equation becomes:
0=(2)2+2(−5)s
0=4−10s
Step 6: Solve for Distance (s)
Rearranging the equation gives:
10s=4
s=410=0.4m
Final Answer
The distance that the box will move relative to the belt before coming to rest is 0.4 meters.
---
The box is dropped onto a conveyor belt that is moving at a constant speed of 2 m/s. The coefficient of friction (μ) between the box and the belt is 0.5. We need to find out how far the box will slide on the belt before it stops relative to the belt.
- Initial speed of the box (u) = 2 m/s (since it was dropped onto the moving belt).
- Final speed of the box (v) = 0 m/s (when it comes to rest relative to the belt).
- Coefficient of friction (μ) = 0.5.
- Acceleration due to gravity (g) = 10 m/s².
The frictional force (f) acting on the box can be calculated using:
f=μ⋅m⋅g
where m is the mass of the box. However, since we are looking for acceleration, we can express it as:
f=μ⋅m⋅g
The acceleration (a) of the box due to friction can be found using Newton's second law:
a=fm=μ⋅m⋅gm=μ⋅g
Substituting the values:
a=0.5⋅10=5m/s2
This acceleration will act in the opposite direction to the motion of the box (deceleration).
We can use the kinematic equation to find the distance (s) the box moves relative to the belt before coming to rest:
v2=u2+2as
Substituting the known values:
- v=0
- u=2m/s
- a=−5m/s2 (negative because it's deceleration)
0=(2)2+2(−5)s
0=4−10s
Rearranging the equation gives:
10s=4
s=410=0.4m
The distance that the box will move relative to the belt before coming to rest is 0.4 meters.
Similar Questions
A conveyor belt is moving with constant speed of 6m/s. A small block is just dropped on it.Coefficient of friction between the two is μ=0.3. Find
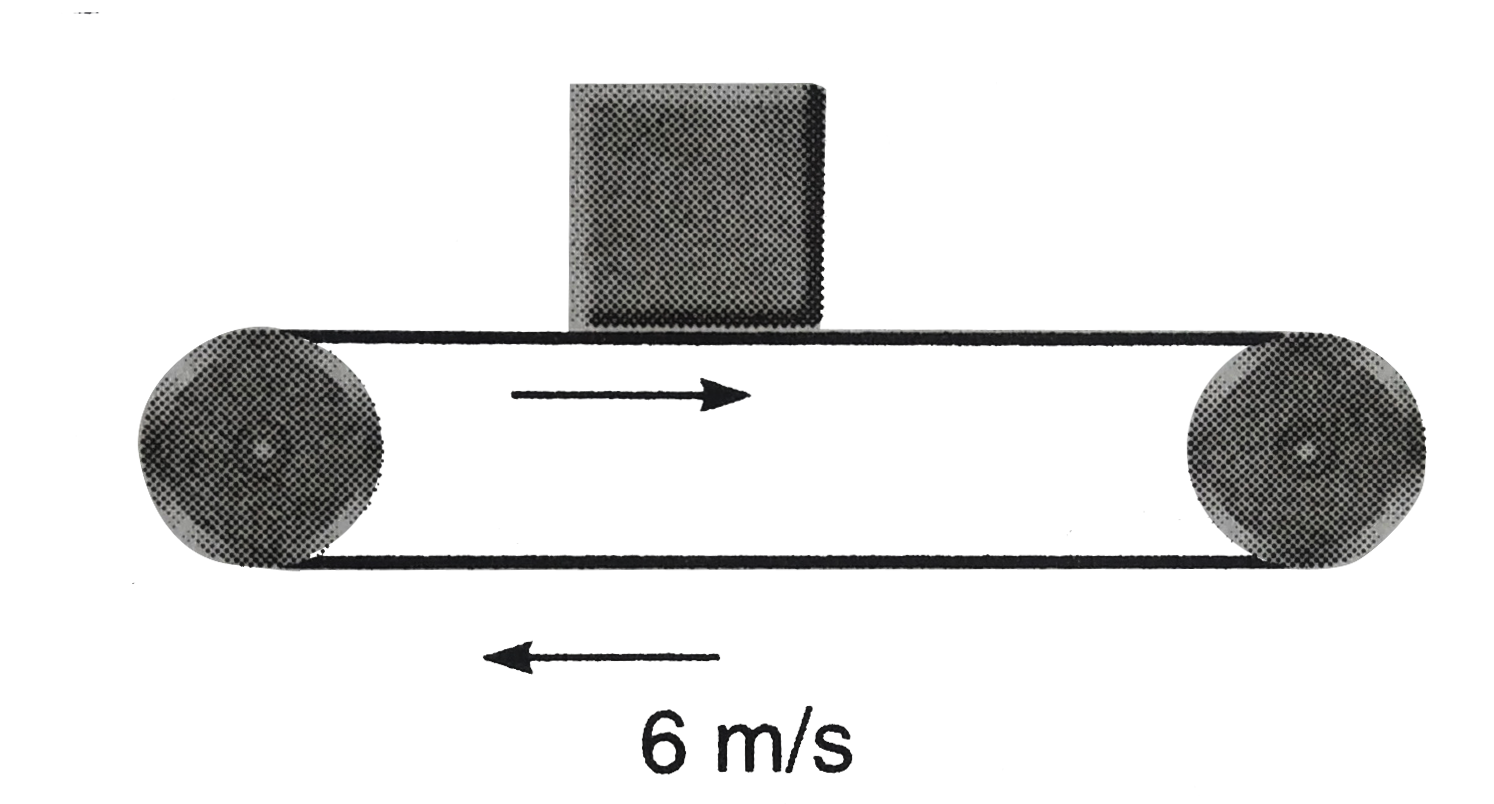
(a) The time when relative motion between them will stop
(b) Displacement of block upto that instant (g=10m/s2).
View SolutionA suitcase is gently dropped on a conveyor belt moving at 3m/s. If the coefficient of friction between the belt and the suitcase is 0.5. Find the displacement of suitcase relative to conveyor belt before the slipping between the two is stopped (g=10m/s2)
View SolutionA suitcase is gently dropped on a conveyor belt moving at 3m/s. If the coefficient of friction between the belt and the suitcase is 0.5. Find the displacement of suitcase relative to conveyor belt before the slipping between the two is stopped (g=10m/s2)
View SolutionThe coefficient of friction between the tyres and road is 0.4. The minimum distance covered before attaining a speed of 8ms−1 starting from rest is nearly ( take g=10ms−2)
View SolutionA body moving with a speed of 54kmph is brought to rest in a distances. If the coefficient of friction between the body and the surface is 0.3. Calculate the distance travelled by the body ?
View SolutionA body is moving up an inclined plane of angle θ with an initial kinetic energy E. The coefficient of friction between the plane and body is μ. The work done against friction before the body comes to rest is
View SolutionA block of mass m is gently placed over a massive plank moving horizontal over a smooth surface with velocity 10ms−1. The coefficient of friction between the block and the plank is 0.2. The distance travelled by the block till it slides on the plank is [g=10ms−2]
View SolutionAn ice skater moving at 10m/s comes to a halt in 100m on a ice surface. Calculate the coefficient of friction between the ice and the skater ?
View SolutionA block is gently placed on a conveyor belt moving horizontal with constant speed After t=4s the velocity of the block becomes equal to velocity of the belt If the coefficient of friction between the block and the belt is μ=0.2, then the velocity of the conveyor belt is .
View SolutionA horizontal force F pulls a 20 kg box at a constant speed along a rough horizotal floor. The coefficient of friction between the box and the floor is 0.25. The work done by force F on the block in displacing it by 2 m is
View Solution