Two glass bulbs x and y are connected by a very small tube having a stop - cock. Bulb X has a volume of and contained the gas, while bulb Y was empty. On opening the stop - cock, the pressure fell down to . The volume of the bulb Y must be
The correct Answer is:C
To solve the problem step by step, we will use Boyle's Law, which states that the pressure of a gas multiplied by its volume is constant when the temperature is constant.
Step 1: Define the initial conditions
Let:
- The initial pressure in bulb X be P.
- The initial volume of bulb X be VX=100cm3.
- The volume of bulb Y be VY (which we need to find).
Step 2: Determine the final pressure
When the stop-cock is opened, the pressure in the system falls to 60% of its initial value. Therefore, the final pressure Pf is:
Pf=0.6P=35P
Step 3: Calculate the total volume after opening the stop-cock
After opening the stop-cock, the total volume VT of the gas is the sum of the volumes of both bulbs:
VT=VX+VY=100+VY
Step 4: Apply Boyle's Law
According to Boyle's Law:
P⋅VX=Pf⋅VT
Substituting the known values:
P⋅100=(35P)⋅(100+VY)
Step 5: Simplify the equation
We can cancel P from both sides (assuming P≠0):
100=35(100+VY)
Step 6: Solve for VY
To eliminate the fraction, multiply both sides by 5:
500=3(100+VY)
Expanding the right side:
500=300+3VY
Now, isolate VY:
500−300=3VY
200=3VY
VY=2003≈66.67cm3
Step 7: Conclusion
The volume of bulb Y is approximately 66.67cm3.
Let:
- The initial pressure in bulb X be P.
- The initial volume of bulb X be VX=100cm3.
- The volume of bulb Y be VY (which we need to find).
When the stop-cock is opened, the pressure in the system falls to 60% of its initial value. Therefore, the final pressure Pf is:
Pf=0.6P=35P
After opening the stop-cock, the total volume VT of the gas is the sum of the volumes of both bulbs:
VT=VX+VY=100+VY
According to Boyle's Law:
P⋅VX=Pf⋅VT
Substituting the known values:
P⋅100=(35P)⋅(100+VY)
We can cancel P from both sides (assuming P≠0):
100=35(100+VY)
To eliminate the fraction, multiply both sides by 5:
500=3(100+VY)
Expanding the right side:
500=300+3VY
Now, isolate VY:
500−300=3VY
200=3VY
VY=2003≈66.67cm3
The volume of bulb Y is approximately 66.67cm3.
Similar Questions
Two glass bulbs A and B are connected by a very small tube having a stop cock. Bulb A has a volume of 100 cm3 and contained the gas, while bulb B was empty. On opening th stop cock. The pressure fell down to 40%. The volume of the bulb B must be:
View SolutionTwo glass bulbs of same volume containing a gas at NTP are connected by a very narrow tube. The pressure is 88.46cm of mercury when one bulb is kept in ice and the other in hot water. Calculate the temperature of the hot water.
View Solutiontwo glass bulbs of equal volume are connected by a narrow tube and are filled with a gas at 0(∘)C at a pressure of 76cm of mercury. One of the bulbs is then placed in melting ice and the other is placed in a water bath maintained at 62∘C.What is the new value of the pressure inside the bulbs? The volume of the connecting tube is neigligible.
View SolutionInitially bulb "a" contained oxygen gas at 27∘C and 950 mm of Hg and bulb "B" contained neon gas at 27∘C and 900 mm of Hg. These bulbs are connected by a narrow tube of negligible volume equipped with a stopcock and gases were allowed to mix-up freely. The pressure obtained in the combined system was found to be 910 mm of Hg.

If volume of bulb B was measured to be 10 L, then find the volume of oxygen gas present initially in bulb "A" .View SolutionA glass bulb of volume 400cm3 is connected to another bulb of volume 200cm3 by means of a tube of negligible volume. The bulbs contain dry air and are both at a common temperature and pressure of 20∘C and 1.000 atm, respectively. The larger bulb is immersed in steam at 100∘C and the smaller in melting ice at 0∘. Find the final common pressure.
View SolutionTwo closed bulbs of equal volume (V) containing an ideal gas initially at pressure Pi and temperature T1 are connected through a narrow tube of negligible volume as shown in the figure below . The temperature of one of the bulbs is then raised to T2 . the final pressure Pf is
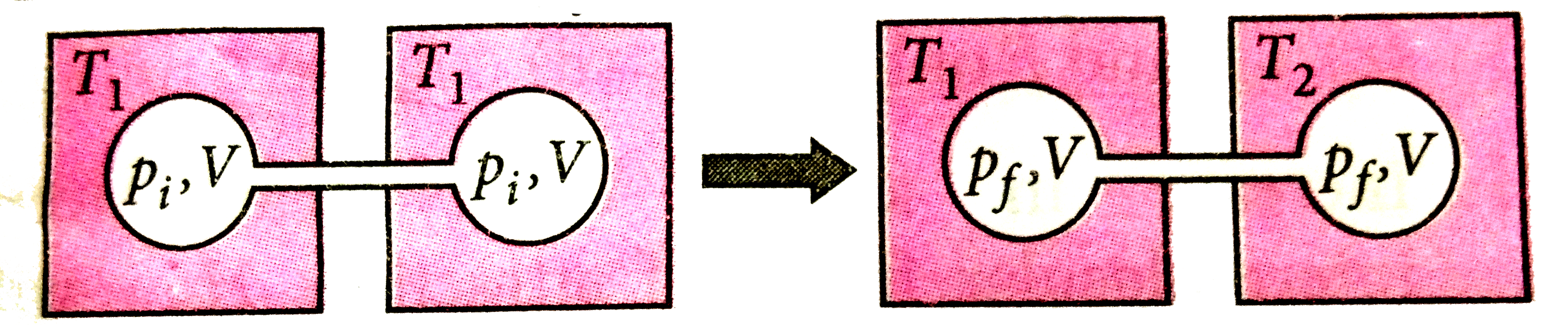 View Solution
View SolutionA bulb A containing gas at 1.5 bar pressure was connected to an evacuated vessel of 1.0 dm3 capacity through a stopcock. The final pressure of the system dropped to 920 mbar at the same temperature. What is the volume of the container A?
View SolutionA certain vessel X has water and nitrogen gas at a total pressure of 2 atm and 300K. All the contents of vessel and transferred to another vessel Y having half the capacity of the vessel X. The pressure of N2 in this vessel was 3.8 atm at 300K. The vessel Y is heated to 320K and the total pressure observed was 4.32 atm. Assume that the volume occupied by the gases in vessel is equal to the volume of the vessel. Calculate the following:
Pressure of H2 at 320K.View SolutionA certain vessel X has water and nitrogen gas at a total pressure of 2 atm and 300K. All the contents of vessel and transferred to another vessel Y having half the capacity of the vessel X. The pressure of N2 in this vessel was 3.8 atm at 300K. The vessel Y is heated to 320K and the total pressure observed was 4.32 atm. Assume that the volume occupied by the gases in vessel is equal to the volume of the vessel. Calculate the following:
Pressure of water vapour at 320K.View SolutionA certain vessel X has water and nitrogen gas at a total pressure of 2 atm and 300K. All the contents of vessel and transferred to another vessel Y having half the capacity of the vessel X. The pressure of N2 in this vessel was 3.8 atm at 300K. The vessel Y is heated to 320K and the total pressure observed was 4.32 atm. Assume that the volume occupied by the gases in vessel is equal to the volume of the vessel. Calculate the following:
Enthalpy of vapourization.View Solution

