How many are example of passive immunity?
P. Vaccination
Q. crossing of antibodies from mother to foetus through the placenta
R. Graft rejection
S. Injections given to patents of snakebite
T. Tetanus antitoxin
Two
Three
Four
Five
The correct Answer is:B
To determine how many examples of passive immunity are present in the given options, let's analyze each one step by step.
1. Vaccination (P):
- Vaccination involves the introduction of antigens into the body to stimulate the immune system to produce antibodies. This process leads to the development of active immunity, as the body produces its own antibodies in response to the vaccine.
- Conclusion: This is NOT an example of passive immunity.
2. Crossing of antibodies from mother to fetus through the placenta (Q):
- This process involves the transfer of maternal antibodies to the fetus, providing the fetus with immediate immunity. Since these antibodies are produced by the mother and transferred to the fetus, this is a classic example of passive immunity.
- Conclusion: This IS an example of passive immunity.
3. Graft rejection (R):
- Graft rejection is a process where the immune system recognizes the transplanted tissue as foreign and mounts an immune response against it. This involves cellular immunity rather than antibody-mediated immunity.
- Conclusion: This is NOT an example of passive immunity.
4. Injections given to patients of snakebite (S):
- In cases of snakebite, patients are often given antivenom, which contains antibodies that neutralize the venom. This is a direct transfer of antibodies into the patient’s body, making it a form of passive immunity.
- Conclusion: This IS an example of passive immunity.
5. Tetanus antitoxin (T):
- Tetanus antitoxin is an injection of antibodies that provides immediate protection against tetanus. Like the snakebite antivenom, this involves the administration of antibodies produced outside the body.
- Conclusion: This IS an example of passive immunity.
Now, let's summarize the findings:
- Passive immunity examples:
- Q (Crossing of antibodies from mother to fetus)
- S (Injections for snakebite)
- T (Tetanus antitoxin)
Thus, there are 3 examples of passive immunity in the given options.
Final Answer:
The number of examples of passive immunity is 3.
- Vaccination involves the introduction of antigens into the body to stimulate the immune system to produce antibodies. This process leads to the development of active immunity, as the body produces its own antibodies in response to the vaccine.
- Conclusion: This is NOT an example of passive immunity.
- This process involves the transfer of maternal antibodies to the fetus, providing the fetus with immediate immunity. Since these antibodies are produced by the mother and transferred to the fetus, this is a classic example of passive immunity.
- Conclusion: This IS an example of passive immunity.
- Graft rejection is a process where the immune system recognizes the transplanted tissue as foreign and mounts an immune response against it. This involves cellular immunity rather than antibody-mediated immunity.
- Conclusion: This is NOT an example of passive immunity.
- In cases of snakebite, patients are often given antivenom, which contains antibodies that neutralize the venom. This is a direct transfer of antibodies into the patient’s body, making it a form of passive immunity.
- Conclusion: This IS an example of passive immunity.
- Tetanus antitoxin is an injection of antibodies that provides immediate protection against tetanus. Like the snakebite antivenom, this involves the administration of antibodies produced outside the body.
- Conclusion: This IS an example of passive immunity.
- Passive immunity examples:
- Q (Crossing of antibodies from mother to fetus)
- S (Injections for snakebite)
- T (Tetanus antitoxin)
The number of examples of passive immunity is 3.
Similar Questions
Immunity that develops in foetus after receiving antibodies from mother's blood through placenta is
View SolutionA given line L1 cut x and y-axes at PandQ respectively and has intercepts aandb2 on x and y-axes respectively. Let another line L2 perpendicular to L1 cut x and y-axes at RandS respectively. Let T be the point of intersection of PSandQR. A straight line passes through the centre of locus of T. Then locus of the foot of perpendicular to it from origin is : (A) a straight line (B) a circle (C) a parabola (D) none of these
View Solution
Knowledge Check
Read the following statements and select the correct ones.
(i) Vaccine is a preparation (or suspension ) of a dead/attenuated pathogen of a disease which on inoculation ( or injection ) into a healthy person, provides temporary/permanent active immunity by inducing antibodies formation.
(ii) Immunisation is the process by which the body produces antibodies against the vaccine preventable diseases through administration of specific vaccines.
(iii) The principle of immunisation or vaccination is based on the property of 'memory' of the immune system.
iv) If a person is infected with some deadly microbes to which quick immune response is required; in that case, we need to directly inject the performed antibodies or antitoxins e.g., in case of tetanus.A(i) and (ii)B(iii) and (iv)C(i), (ii) and (iii)D(i),(ii),(iii) and (iv)
Consider a uniform wire of length l. Cross-sectional area A, Young's modulus of the material of the wave is Y. Some information related to the wire is given in column-1 and dependence of the result is given in column-2. Then match the approprite choice between the columns and match the list given in options
Column-I Column-II(A) Let us suspend wire vertically from a (p) Young'sModulusrigud support and attach a mass m atits lower end. If the mass is slightly pulleddown and released, it executes S.H.Mof a time period which will depend on(B) Work done in stretching the wire up to length (q) elongation (x)l+xwill depend on(C) If the given is fixed between two rigid (r) length (l)supports and its temperature is decreasedthermal stress that develops in the wirewill depend on(D) If the wire is pulled at its ends equal and (s) area of cross-section (A) opposite forces of magnitude F so that itundergoes an elongation x, according toHook's law, F=kx, where k is the forceconstant.Force constant (k) of the wirewill depend on
View SolutionRead the following matching carefully :
(i) Artificial active immunity : vaccination
(ii) Natural passive immunity : Anti - tetanus serum (ATS)
(iii) First line of defense : physical barriers
(iv ) Anamnestic responses : Quick and highly intensified response
(V) Graft rejection : Due to cell - mediated immunity (CMI)
How many from the above matching's are correct ?
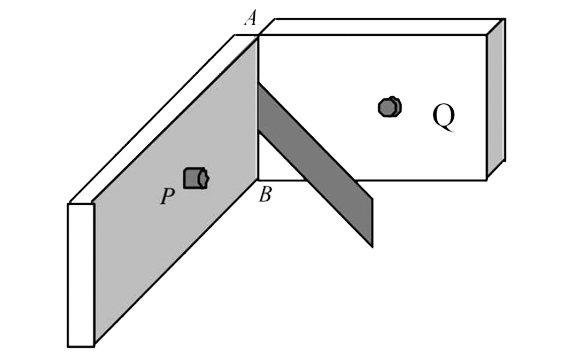
View SolutionTwo heavy metallic plates are joined together at 90∘ to each other. A laminar sheet of mass 30kg is hinged at the line AB joining the two heavy metallic plates. The hinges are frictionless. The moment of inertia of the laminar sheet about an axis parallel to AB and passing through its center of mass is 1.2 kg m2. Two rubber obstacles P and Q are fixed, one on each metallic plate at a distance 0.5m from the line AB. This distance is chosen so that the reaction due to the hinges on the laminar sheet is zero during the impact. Initially the laminar sheet hits one of the obstacles with an angular velocity 1 rad/s and turns back. If the impulse on the sheet due to each obstacal is 6 N-s,
(a) Find the location of the center of mass of the laminar sheet from AB.
(b) At what angular velocity does the laminar sheet come back after the first impact?
(c) After how many impacts, does the laminar sheet come to rest?
View SolutionP.Normal activities of the heart are regulated extrinsically i. e auto regulated by specialized muscles.
Q. A special neural center in the medulla oblongata can moderate the cardiac function through the autonomic nervous function ( ANS) .
R. Parasympathetic mental signals decrease the rate of heartbeat.
S. Adrenal medullary hormones increase cardiac output.
How many of the given statements are incorrect about the regulation of cardiac activities ?
View SolutionWhen an object moves through a fluid, as when a ball falls through air or a glass sphere falls through water te fluid exerts a viscous foce F on the object this force tends to slow the object for a small sphere of radius r moving is given by stoke's law, Fw=6πηrv. in this formula η in the coefficient of viscosity of the fluid which is the proportionality constant that determines how much tangential force is required to move a fluid layer at a constant speed v, when the layer has an area A and is located a perpendicular distance z from and immobile surface. the magnitude of the force is given by F=ηAv/z. For a viscous fluid to move from location 2 to location 1 along 2 must exceed that at location 1, poiseuilles's law given the volumes flow rate Q that results from such a pressure difference P2−P1. The flow rate of expressed by the formula Q=πR4(P2−P1)8ηL poiseuille's law remains valid as long as the fluid flow is laminar. For a sfficiently high speed however the flow becomes turbulent flow is laminar as long as the reynolds number is less than approximately 2000. This number is given by the formula Re=2¯vρRη In which ¯v is the average speed ρ is the density η is the coefficient of viscosity of the fluid and R is the radius of the pipe. Take the density of water to be ρ=1000kg/m3
Q. If the sphere in previous question has mass of 1×10−5kg what is its terminal velocity when falling through water? (η=1×10−3Pa−s)
A. 1.3m/s
B. 3.4m/s
C. 5.2m/s
D. 6.5m/s
View SolutionDr. Bansal needs to determine the distance PQ across a river in and east-west direction as shown in the adjoining figure. He can't measure this distance directly over the water. So, he selects the point S from where a straight line to point Q stays on land so he can mesure distance. he then moves eastward a distance of 400 m from point S to T , so that the line of sight from point T to P cuts the previous line SQ at R. finally with a long measuring tape. he determines that. SR = 250 m, QR= 1250 m
Determine if this is enough information to calculate the distance PQ and if so, find PQ and hence find the time taken by a swimmer to cross the river PQ with a uniform speed of 800 m/hr.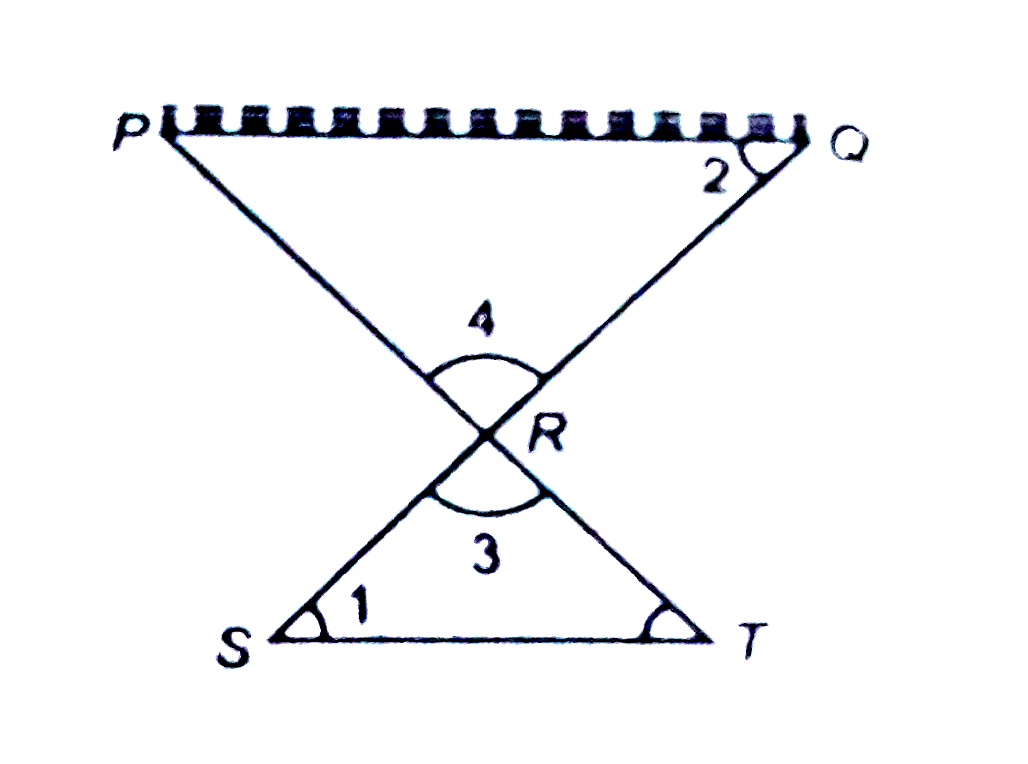
View SolutionWhen an object moves through a fluid, as when a ball falls through air or a glass sphere falls through water te fluid exerts a viscous foce F on the object this force tends to slow the object for a small sphere of radius r moving is given by stoke's law, Fw=6πηrv. in this formula η in the coefficient of viscosity of the fluid which is the proportionality constant that determines how much tangential force is required to move a fluid layer at a constant speed v, when the layer has an area A and is located a perpendicular distance z from and immobile surface. the magnitude of the force is given by F=ηAv/z. For a viscous fluid to move from location 2 to location 1 along 2 must exceed that at location 1, poiseuilles's law given the volumes flow rate Q that results from such a pressure difference P2−P1. The flow rate of expressed by the formula Q=πR4(P2−P1)8ηL poiseuille's law remains valid as long as the fluid flow is laminar. For a sfficiently high speed however the flow becomes turbulent flow is laminar as long as the reynolds number is less than approximately 2000. This number is given by the formula Re=2¯vρRη In which ¯v is the average speed ρ is the density η is the coefficient of viscosity of the fluid and R is the radius of the pipe. Take the density of water to be ρ=1000kg/m3
Q. What is the viscous force on a glass sphere of radius r=1mm falling through water (η=1×10−3Pa−s) when the sphere has speed of 3m/s?
View Solution
