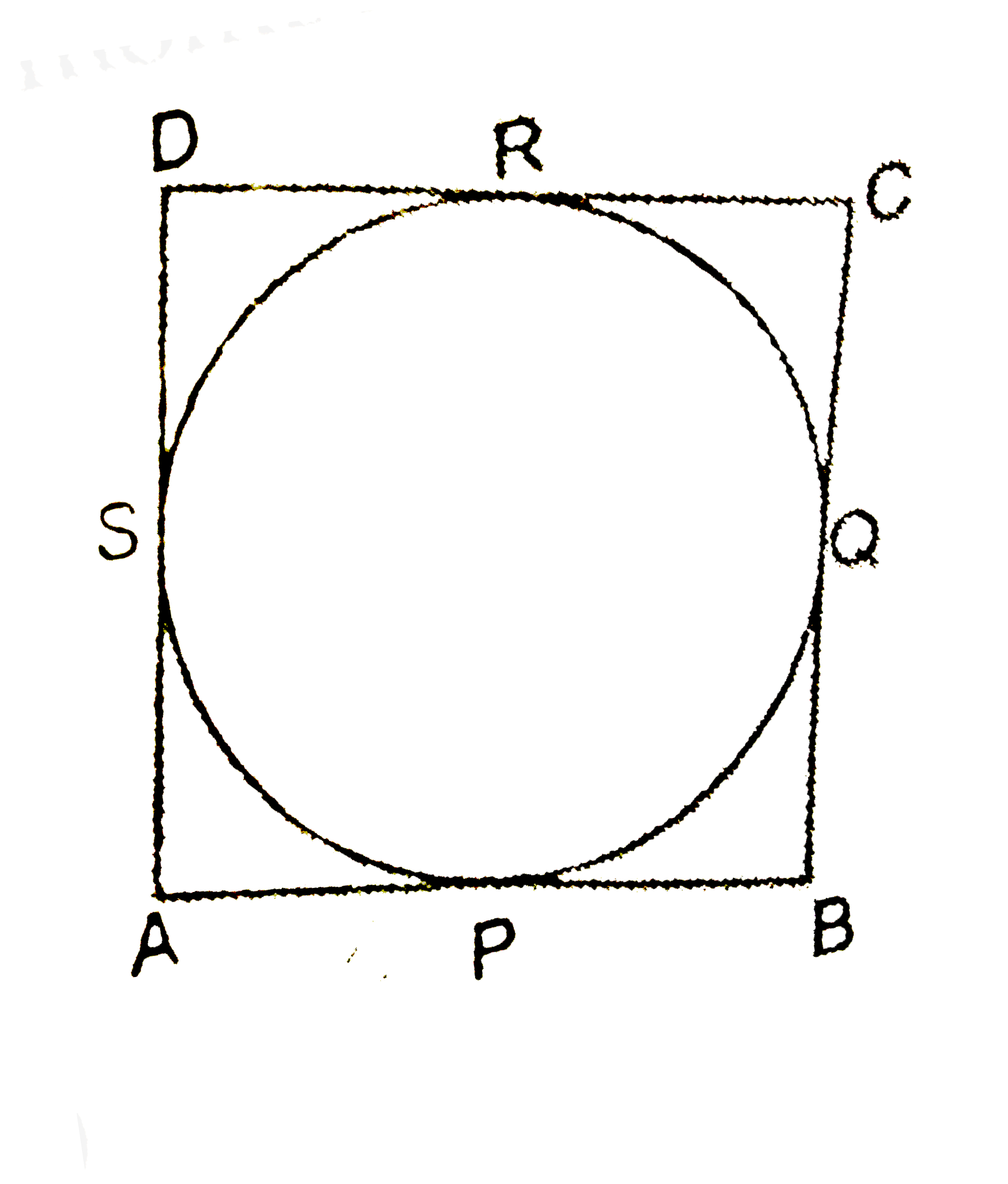
prove that the parallelogram circumscribing a circle is a rhombus.
Step by step video & image solution for prove that the parallelogram circumscribing a circle is a rhombus. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
Assertion(A) If two tangents are drawn to a circle from an external point then they subtend equal angles at the centre.
Reason (R ) A parallelogram circumscribing a circle is a rhombusABoth Assertion (A) and Reason (R ) are true and Reason (R ) is a correct explanation of Asseration (A).BBoth Assertion (A) and Reason (R ) are true but Reason (R ) is not a correct explanation of Asseration (A).CAssertion(A) is true and Reason (R ) is false.DAssertion(A) is false and Reason (R ) is true.
If a parallelogram circumscribes a circle then prove that it must be a rhombus.
 View Solution
View SolutionProve that opposite sides of a quadrilateral circumscribing a circle subtend supplementary angles at the centre of the circle.
View SolutionProve that opposite sides of a quadrilateral circumscribing a circle, subtend supplementary angles at the centre of a circle.
View SolutionProve that opposite sides of a quadrilateral circumscribing a circle subtend supplementary angles at the centre of the circle.
View SolutionProve that the centre of the circle circumscribing the cyclic rectangle ABCD is the point of intersection of its diagonals.
View SolutionRhombus a parallelogram having all sides equal is called a rhombus.
View SolutionRhombus a parallelogram having all sides equal is called a rhombus.
View Solution

