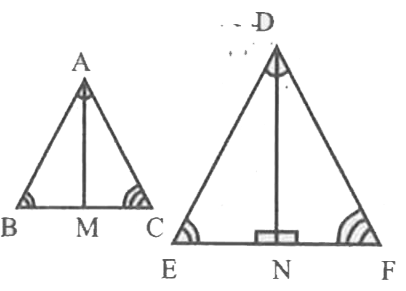
AX and DY are altitudes of two similar triangle ΔABC and ΔDEF. Prove that AX:DY=AB:DE.Solution in Telugu
Step by step video & image solution for AX and DY are altitudes of two similar triangle DeltaABC and DeltaDEF. Prove that AX:DY=AB:DE. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
In △ABC, DE∣∣BC and AD=13BD.
If BC = 4.5cm, Find DE.
View SolutionWhich of the following are sets? Justify your answer.
The collection of all even integers.
View SolutionThe median of the data x/5, x, x/4, x/2 and x/8 is 8. Find the value of x.
View SolutionΔABC तथा ΔDEF समरूप है तथा BC=3cm,EF=4cm,ΔABC का क्षेत्रफल =54cm2 तो ΔDEF का क्षेत्रफल ज्ञात करें?
View Solutionदो त्रिभुज ΔABC तथा ΔDEF समरूप है तथा AB=10cm,DE=8cm,ΔABC तथा ΔDEF के क्षेत्रफल का अनुपात ज्ञात करें?
View SolutionIf ΔABC~ΔEDF and ΔABC is not similar to ΔDEF, then which of the following is not true?
View Solutionनीचे दिए गए चित्र में ΔABC~ΔDEF जिसमे (AXऔर DY क्रमशः ∠A और ∠D के अर्द्धक है । यदि AX=6.5cm और DY=5.2cm तो ΔDEF के क्षेत्रफलों के अनुपात ज्ञात करें ।
View SolutionAX and DY are altitudes of two similar triangle ΔABC and ΔDEF. Prove that AX:DY=AB:DE.
View SolutionProve that " the ratio of areas of two similar triangles is equal to the square of the ratio of their altitudes.
View SolutionΔABCandΔDEF are two similar triangle and the primeters of ΔABCandΔDEF are 30 cm and 18 cm respectively . If the length of DE = 36 cm , then length of AB is
View SolutionAX and DY are altitudes of two similar triangles ΔABCandΔDEF. Prove that AX : DY = AB : DE.
View SolutionAX and DY are altitudes of two similar triangles ΔABCandΔDEF. Prove that AX : DY = AB : DE.
View SolutionBE और CF एक त्रिभुज ABC के दो बराबर शीर्षलम्ब है | RHS सर्वाग्समता नियम का प्रयोग करके सिद्ध कीजिए कि ΔABC एक समद्धिबाहू त्रिभुज है |
View SolutionAX and DY are altitudes of two similar triangles ΔABCandΔDEF. Prove that AX : DY = AB : DE.
View SolutionIf ΔABC~ΔEDF and ΔABC is npot similar to ΔDEF, then which of the following is not true?
View SolutionProve that the ratio of altitudes of two similar triangles is equal to the ratio of their corresponding sides.
View Solution
