The coordinates o the foot of the perpendicular drawn from the origin to the line joining the point (−9,4,5)and(10,0,−1) will be a. (−3,2,1) b. (1,2,2) c. 4,5,3 d. none of theseSolution in Tamil
(-3,2,1)
(1,2,2,)
(4,5,3)
none of these
The correct Answer is:D
Let AD be the perpendicular and D be the foot of the perpendicular which divides BC in the ratio λ:1, then
D(10λ−9λ+1,4λ+1,−λ+5λ+1) (i)
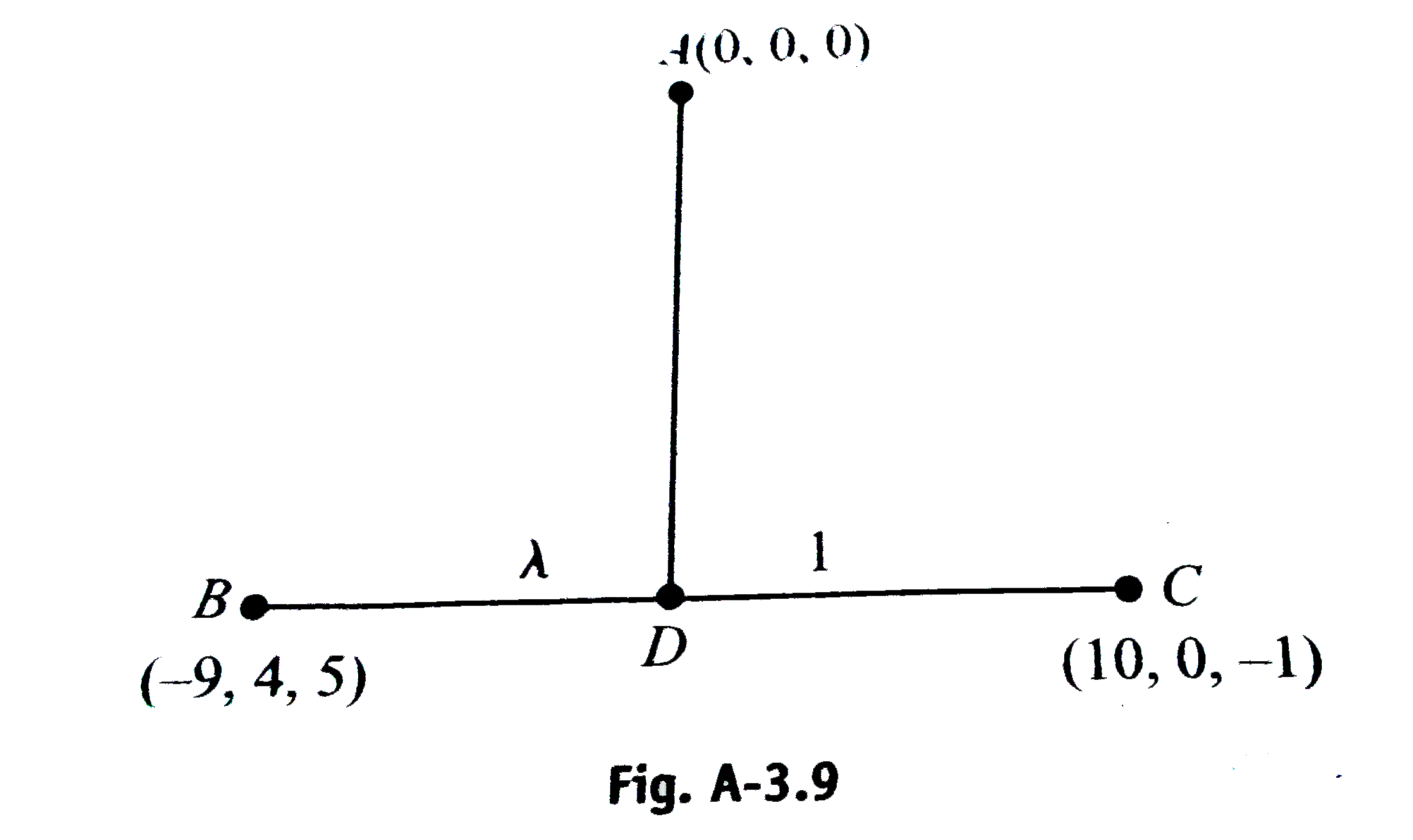
The direction ratio of AD are 10λ−0λ+1,4λ+1and−λ+5λ+1 and direction ratios of BC are 19,-4 and -6.
Since AD⊥BC, we get
19(10λ−9λ+1)−4(4λ+1)−6(−λ+5λ+1)=0
or λ=3128
Hence, on putting the value of λ in (i) ,we get required foot of the perpendicular, i.e., (5859,11259,10959).
Topper's Solved these Questions
- BOOK - CENGAGECHAPTER - THREE-DIMENSIONAL GEOMETRY EXERCISE - Exercise (Multiple)17videos
THREE-DIMENSIONAL GEOMETRY
- BOOK - CENGAGECHAPTER - THREE-DIMENSIONAL GEOMETRY EXERCISE - Exercise (Reasoning Questions)10videos
THREE-DIMENSIONAL GEOMETRY
- BOOK - CENGAGECHAPTER - THREE-DIMENSIONAL GEOMETRY EXERCISE - SUBJECTIVE TYPE1videos
THREE-DIMENSIONAL GEOMETRY
- BOOK - CENGAGECHAPTER - THREE DIMENSIONAL GEOMETRYEXERCISE - Question Bank12videos
THREE DIMENSIONAL GEOMETRY
- BOOK - CENGAGECHAPTER - TRIGNOMETRIC RATIOS IDENTITIES AND TRIGNOMETRIC EQUATIONSEXERCISE - Question Bank4videos
TRIGNOMETRIC RATIOS IDENTITIES AND TRIGNOMETRIC EQUATIONS
Similar Questions
Let PM be the perpendicular from the point P(1,2,3) to the x−y plane. If →OP makes an angle θ with the positive direction of the z− axis and→OM makes an angle φ with the positive direction of x− axis, whereO is the origin and θandφ are acute angels, then a. cosθcosφ=1/√14 b. sinθsinφ=2/√14 c. tanφ=2 d. tanθ=√5/3
06:08View SolutionStatement 1 : Lines →r=ˆi−ˆj+λ(ˆi+ˆj−ˆk)and→r=2ˆi−ˆj+μ(ˆi+ˆj−ˆk) do not intersect.
Statement 2 : Skew lines never intersect.06:05View SolutionProve that the volume of tetrahedron bounded by the planes →r.mˆj+nˆk=0,→r.nˆk+lˆi=0,→r.lˆi+mˆj=0,→r.lˆi+mˆj+nˆk=πs2p33lmn
05:42View SolutionThe equation of the plane which has the property that the point Q(5,4,5) is the reflection of point P(1,2,3) through that plane, is ax+by+cz=d where a,b,c,d∈N. Find the least value of (a+b+c+d).
15:02View SolutionThe number of solution (s) of the equation
√2+cos2x=(sinx+cosx)∈[−π2,π]03:36View SolutionFind the coordinates of the foot of the perpendicular drawn from the point A(1,2,1) to the line joining B(1,4,6)andC(5,4,4).
06:52View SolutionFind the coordinates of the foot of perpendicular drawn from th point A(1,8,4) to the line joining the points B(0,−1,3)and C(2−3,−1).
06:05View SolutionThe coordinates o the foot of the perpendicular drawn from the origin to the line joining the point (−9,4,5)and(10,0,−1) will be a. (−3,2,1) b. (1,2,2) c. 4,5,3 d. none of these
05:16View SolutionFind the coordinates of the foot of the perpendicular drawn from the point A(1,8,4) to the line joining the points B(0,-1,3) and C(2,-3,-1)
05:11View SolutionThe point in which the join of (-9,4,5) and (11,0,-1) is met by the perpendicular from the origin is-
04:15View Solutionबिंदुओं (-9,4,5) व (10,0,-1) को मिलाने वाली रेखा पर मूलबिंदु से डाले गए लम्ब के पाद बिंदु के निर्देशांक हैं
03:48View SolutionFind the coordinates of the foot of perpendicular drawn from th point A(1,8,4) to the line joining the points B(0,−1,3)andC(2−3,−1).
05:42View SolutionFind the coordinates of the foot of the perpendicular drawn from point A(1,0,3) to the join of points B(4,7,1)andC(3,5,3).
08:51View SolutionThe coordinates o the foot of the perpendicular drawn from the origin to the line joining the point (−9,4,5)and(10,0,−1) will be a. (−3,2,1) b. (1,2,2) c. 4,5,3 d. none of these
05:36View SolutionFind the coordinates of the foot of the perpendicular drawn from point A(1,0,3) to the join of points B(4,7,1)andC(3,5,3).
View SolutionThe coordinates of the foot of the perpendicular drawn from the origin to the line joining the point (−9,4,5) and (10,0,−1) will be
04:37View Solution

