Write a rational function g with vertical asymptotes at x=3 and x=−3, a horizontal asymptote at y=−4 and with no x-intercept.Solution in Tamil
⋅ Since g has vertical is asymptotes x = 3 and x = -3, then the denominator of the rational function contains the product of (x-3) and (x+3) . Function g has the form
g(x)=h(x)(x−3)(x+3)
⋅ For the horizontal asymptote to exist, the numerator h(x) of g(x) has same degree as that of the denominator with a leading coefficient equal to -4. At the same time, h(x) has no real zeros. Hence
f(x)=−4x2−6(x−3)(x+3)
⋅ Check the characteristics in the graph of g shown below.
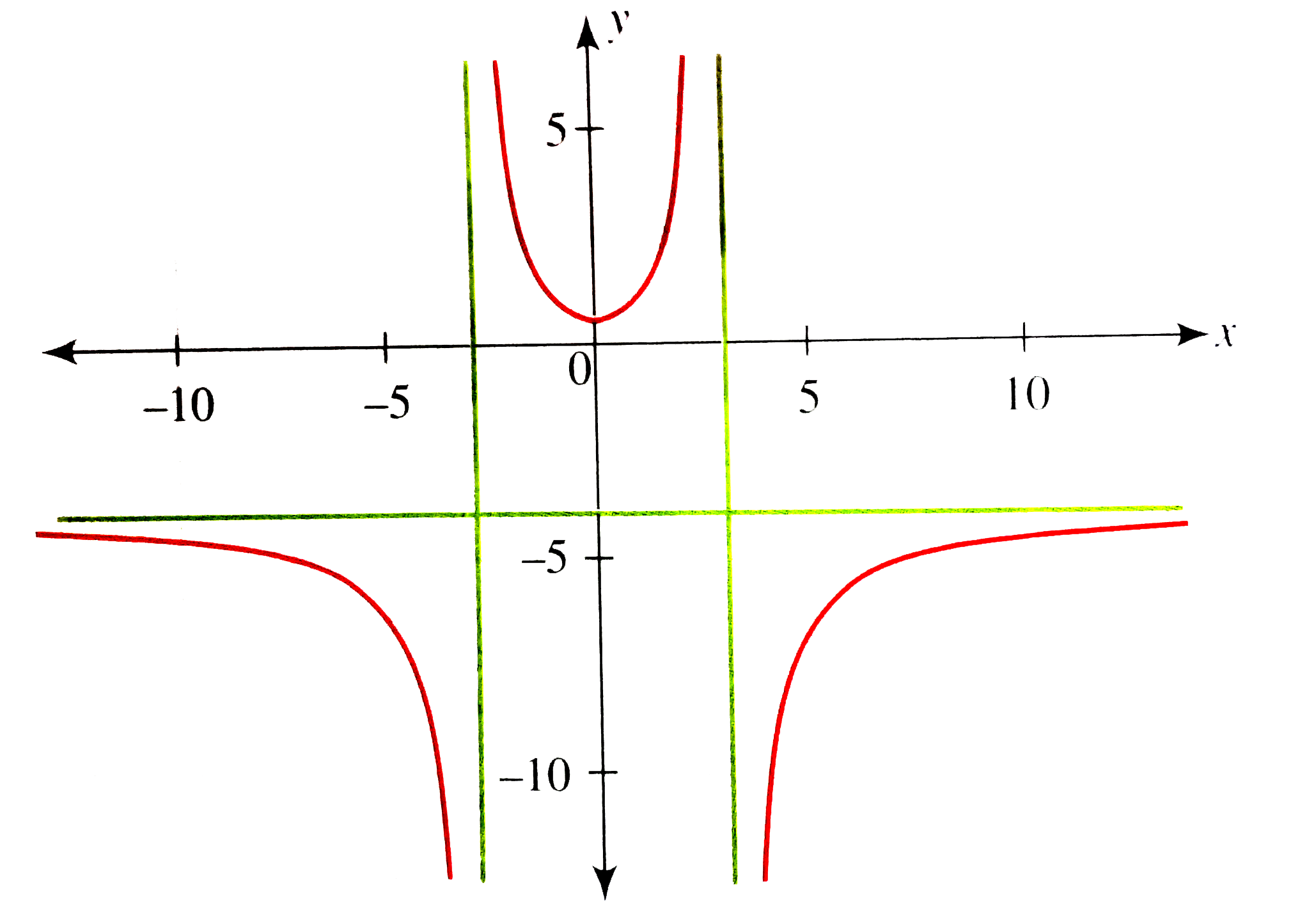
Topper's Solved these Questions
- GRAPHS OF POLYNOMIAL AND RATIONAL FUNCTIONSBOOK - CENGAGECHAPTER - GRAPHS OF POLYNOMIAL AND RATIONAL FUNCTIONSEXERCISE - Exercise
13 Videos
- GRAPHS OF POLYNOMIAL AND RATIONAL FUNCTIONSBOOK - CENGAGECHAPTER - GRAPHS OF POLYNOMIAL AND RATIONAL FUNCTIONSEXERCISE - Exercises
4 Videos
- GRAPHICAL TRANSFORMATIONSBOOK - CENGAGECHAPTER - GRAPHICAL TRANSFORMATIONSEXERCISE - Exercise
14 Videos
- GRAPHS OF ELEMENTARY FUNCTIONS BOOK - CENGAGECHAPTER - GRAPHS OF ELEMENTARY FUNCTIONS EXERCISE - Exercise
34 Videos
Similar Questions
Draw the graph of y=(x−1)(x2−x+1).
View SolutionSketch the graph of an example of a rational function f that satisfies all the given conditions.
(i) f(0)=0,f(1),limx→∞f(x)=0,f is odd
(ii) limx→0+f(x)=∞,limx→0−f(x)=−∞,limx→∞f(x)=1,limx→−∞f(x)=1
(iii) limx→−2f(x)=∞,limx→−∞f(x)=3,limx→∞f(x)=3,f(0)=0
View SolutionDraw the graph of y=tan−1x−cot−1x.
View SolutionDraw the graph of y=1(1/x).
View SolutionWrite a possible rational function h with a hole at x = 5, a vertical asymptote at x = -1, a horizontal asymptote at y = 2 and x-intercept at x = 2.
View SolutionWrite a rational function g with vertical asymptotes at x = 3 and x = -3, a horizontal asymptote at y = -4 and with no x-intercept.
View SolutionWrite a rational function f with a slant asymptote y=x+4, a vertical asymptote at x = 5 and one of the zeros at x = 2.
View SolutionWrite a possible rational function f that has a vertical asymptote at x = 2, a horizontal asymptote y = 3 and a zero at x = -5. Also draw the graph of the function.
View SolutionWhat is the equation of the horizontal asymptote of the function f(x)=(2x−1)(x+3)(x+3)2?
View SolutionWrite a rational function g with vertical asymptotes at x = 3 and x = -3, a horizontal asymptote at y = -4 and with no x-intercept.
View SolutionWrite a rational function f with a slant asymptote y=x+4, a vertical asymptote at x = 5 and one of the zeros at x = 2.
View SolutionWrite a possible rational function f that has a vertical asymptote at x = 2, a horizontal asymptote y = 3 and a zero at x = -5. Also draw the graph of the function.
View SolutionFind all the vertical asymptotes and holes of (x−3)2(x−4)(x−5)(x−3).
View SolutionFind the asymptotes, intercepts and sketch the graph of the function f(x)=x3x2−9
View SolutionFind the asymptotes, intercepts and sketch the graph the function f(x)=x2−x−6x−3
View Solution
