Sketch the regions which points satisfy |x+y|≥2. Solution in Tamil
|x+y|≥2
x+y≤−2oex+y≥2
That is,the points lie in the region satisfying the inequality x+y+2≥0orx+y−2≤0.
For x+y+2≤0, the points lie on the line x+y+2=0 or in the half region in which (0,0) does not lie as (0, 0) does not satisfy x+y+2≤0.
For x+y−2≥0, the points lie on the (0, 0) does not satisfy x+y−2≥0.
Hence the required region is as shown in the following figure.
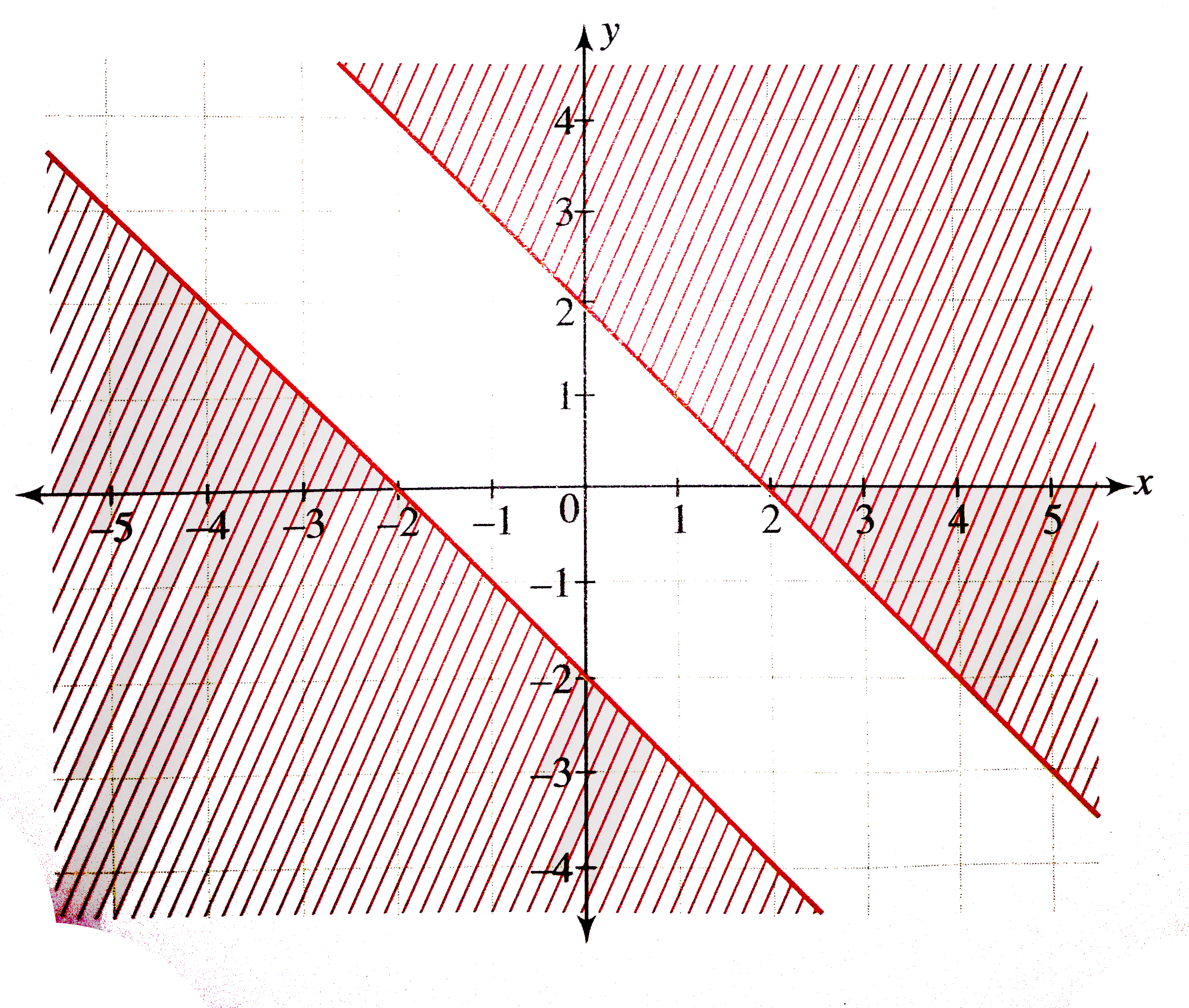
Topper's Solved these Questions
- GRAPHS OF ELEMENTARY FUNCTIONS BOOK - CENGAGECHAPTER - GRAPHS OF ELEMENTARY FUNCTIONS EXERCISE - Exercise
34 Videos
- GRAPHS OF POLYNOMIAL AND RATIONAL FUNCTIONSBOOK - CENGAGECHAPTER - GRAPHS OF POLYNOMIAL AND RATIONAL FUNCTIONSEXERCISE - Exercises
4 Videos
- GRAPHS OF TRIGONOMETRIC FUNCTIONSBOOK - CENGAGECHAPTER - GRAPHS OF TRIGONOMETRIC FUNCTIONSEXERCISE - Exercise
22 Videos
Similar Questions
Draw the graph of y=1(1/x).
View SolutionSketch the graph of an example of a rational function f that satisfies all the given conditions.
(i) f(0)=0,f(1),limx→∞f(x)=0,f is odd
(ii) limx→0+f(x)=∞,limx→0−f(x)=−∞,limx→∞f(x)=1,limx→−∞f(x)=1
(iii) limx→−2f(x)=∞,limx→−∞f(x)=3,limx→∞f(x)=3,f(0)=0
View SolutionDraw the graph of y= cosec 2x−cot2x. Is f(x) periodic ? If yes, what is its fundamental period ?
View SolutionArea bounded by the region consisting of points (x,y) satisfying y≤√2−x2,y2≥x,√y≥−x is
View SolutionSketch the regions satisfying the following inequalities:
(a) x>2
(b) |y|≥1
View SolutionSketch the region of the points satisfying max.{|x|,|y|}≤4.
View SolutionSketch the regions which points satisfy |x+y|≥2.
View SolutionSketch the region satisfying |x|<|y|.
View SolutionGiven that x∈[0,1] and y∈[0,1]. Let A be the event of selecting a point (x,y) satisfying y2≥x and B be the event selecting a point (x,y) satisfying x2≥y, then
View SolutionSketch the regions satisfying the following inequalities:
(a) x>2
(b) |y|≥1
View SolutionSketch the region of the points satisfying max.{|x|,|y|}≤4.
View SolutionSketch the regions which points satisfy |x+y|≥2.
View SolutionSketch the region satisfying |x|<|y|.
View SolutionGiven that x∈[0,1] and y∈[0,1]. Let A be the event of selecting a point (x,y) satisfying y2≥x and B be the event selecting a point (x,y) satisfying x2≥y, then
View SolutionArea bounded by the region consisting of points (x,y) satisfying y≤√2−x2,y2≥x,√y≥−x is
View Solution