Question

In the adjoining figure , $\Delta PQR$ is an equilateral triangle. QR = RN . Prove that $P{N}^2=3P{R}^2$

Explanation

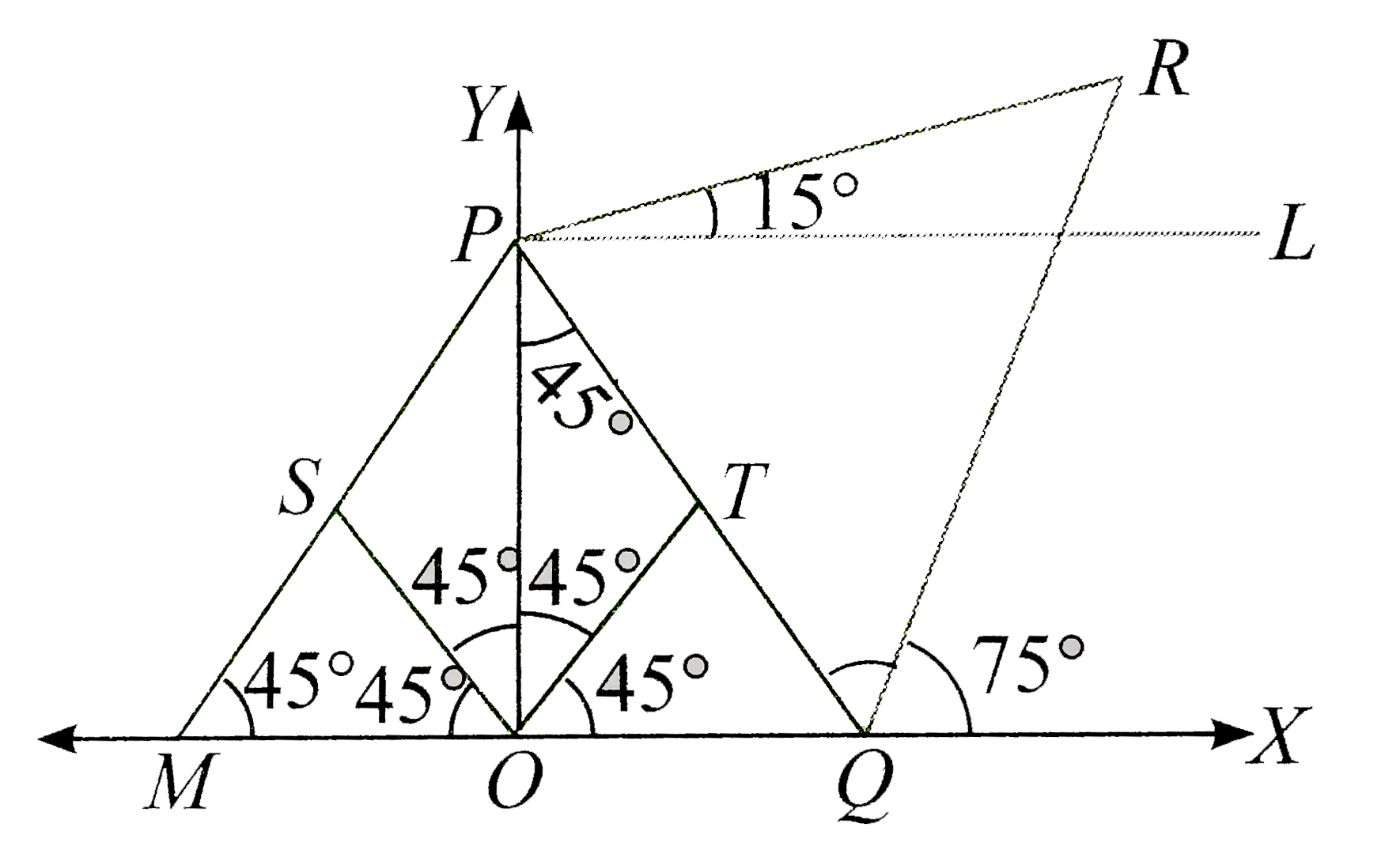
Equation of line OT:
`O-=(0,0) " and the slope of OT is tan" 45^(@) = 1`. Therefore, the equation of line OT will be
y-0=1(x-0)
or x-y=0
Equation of line OS:
`O-=(0,0) " and " angle SOX = 135^(@)`. Therefore, the slope of line OS is `"tan "135^(@)` =-1.
The equation of line OS will be
y-0 = (-1)(x-0)
or y=-x or x+y=0
Equation of line SP:
`"Given that OT" = 2sqrt(2). "Therefore, OP" =OT "sec" 45^(@) = 2sqrt(2)sqrt(2) = 4.` So,
`P-= (0,4). "Also, the slope of SP is tan" 45^(@) = 1`
Therefore, the equation of lien SP will be
Y-4 = 1 (x-0)
or x-y+4=0
Equation of QR:
Given that OQ = OT sec `45^(@) = 2sqrt(2)sqrt(2) = 4`. Therefore, `Q-=(4,0).`
Also, the slope of line QR is tan `75^(@) = 2+sqrt(3)`.
Therefore, the equation of line QR will be
y-0 `=(2+sqrt(3))(x-4)`
or `(2+sqrt(3))x-y-8-4sqrt(3)=0`
Equation of PR:
`P-=(0,4)`
`"Slope of line " PR = tan15^(@) = 2-sqrt(3)`
Therefore, the equation of line PR is
`y-4 = (2-sqrt(3))(x-0)`
`" or " y-4 = (2-sqrt(3))x`
`" or " (2-sqrt(3))x-y+4 = 0`
Equation of PQ :
`P -= (0,4) and Q-= (4,0)`
Therefore, the equation of line PQ will be
`(x)/(4) + (y)/(4) = 1`
or x+y = 4