A≡(−4,0),B≡(4,0).MandN are the variable points of the y-axis such that M lies below NandMN=4 . Lines AMandBN intersect at P. The locus of P is a. 2xy−16−x2=0 b. 2xy+16−x2=0 c. 2xy+16+x2=0 d. 2xy−16+x2=0Solution in Tamil
2xy−16−x2=0
2xy+16−x2=0
2xy+16+x2=0
2xy−16+x2=0
The correct Answer is:D
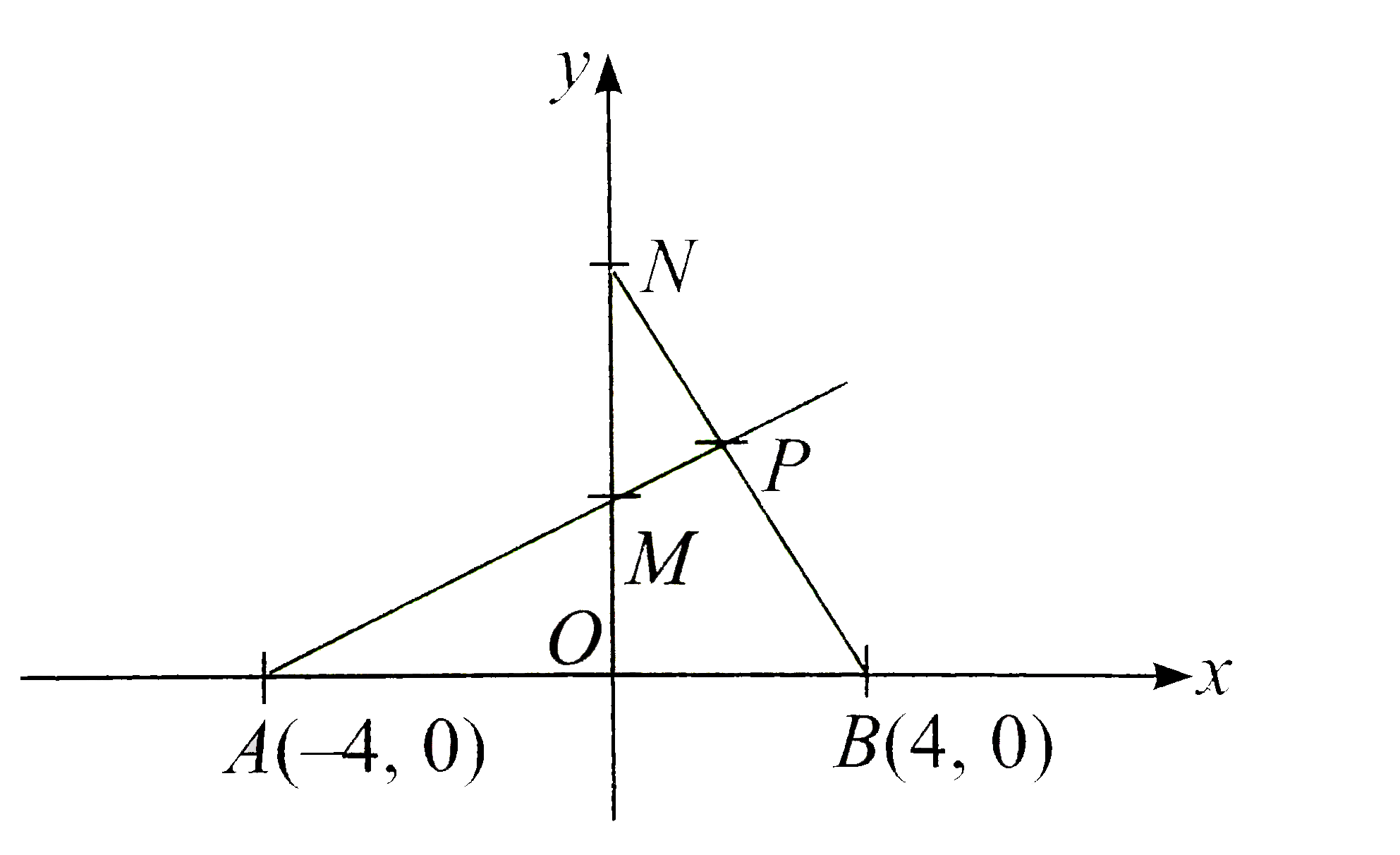
Let M≡(0,h).Then
or N≡(0,h+4)
The equation of AM is
x−4+yh=1
or yh=4+x4
or h=4y4+x
The equation of BN is
x4+yh+4=1
or yh+4=4−x4
or h+4=4y4−x
or h=4y−16+4x4−x
or 4(y−4+x)4−x=4y4+x (Eliminating h)
or 2xy−16+x2=0, which is the required locus
Topper's Solved these Questions
- STRAIGHT LINESBOOK - CENGAGECHAPTER - STRAIGHT LINESEXERCISE - Exercise (Multiple)
30 Videos
- STRAIGHT LINESBOOK - CENGAGECHAPTER - STRAIGHT LINESEXERCISE - Exercise (Comprehension)
27 Videos
- STRAIGHT LINESBOOK - CENGAGECHAPTER - STRAIGHT LINESEXERCISE - Exercise 2.6
5 Videos
- STRAIGHT LINEBOOK - CENGAGECHAPTER - STRAIGHT LINEEXERCISE - Multiple Correct Answers Type
8 Videos
- THEORY OF EQUATIONSBOOK - CENGAGECHAPTER - THEORY OF EQUATIONSEXERCISE - JEE Advanced Previous Year
9 Videos
Similar Questions
If P is a point (x,y) on the line y=−3x such that P and the point (3, 4) are on the opposite sides of the line 3x−4y=8, then x>815 (b) x>85 y<−85 (d) y<−815
View SolutionLet L be the line belonging to the family of straight lines (a+2b) x+(a-3b)y+a-8b =0, a, b ∈R, which is the farthest from the point (2, 2).
Area enclosed by the line L and the coordinate axes is
View SolutionIf aandb are two arbitrary constants, then prove that the straight line (a−2b)x+(a+3b)y+3a+4b=0 will pass through a fixed point. Find that point.
View SolutionThe point P(α,α+1) will lie inside the triangle whose vertices are A(0,3),B(−2,0) and C(6,1) if
View SolutionQ. Let p and q real number such that p≠0,p2≠q and p2≠−q. if α and β are non-zero complex number satisfying α+β=−p and α3+β3=q, then a quadratic equation having αβ and βα as its roots is
View SolutionA≡(−4,0),B≡(4,0) M and N are the variable points of the y-axis such that M lies below N and MN=4 . Lines AMandBN intersect at P. The locus of P is (a) 2xy−16−x2=0 (b)2xy+16−x2=0 (c) 2xy+16+x2=0 (d)2xy−16+x2=0
View SolutionSuppose ax+by+c=0 , where a,bandc are in AP be normal to a family of circles. The equation of the circle of the family intersecting the circle x2+y2−4x−4y−1=0 orthogonally is (a)x2+y2−2x+4y−3=0 (b)x2+y2−2x+4y+3=0 (c)x2+y2+2x+4y+3=0 (d) x2+y2+2x−4y+3=0
View SolutionIf the straight line 2x+sqrt(2)y+n=0 touches the hyperbola (x^(2))/(9)-(y^(2))/(16)=1, then find the value of n.
View SolutionIf f(x+y)=f(x).f(y) for all xandy.f(1)=2, and αn=f(n),nεN, then equation of the circle having (α1,α2)and(α3,α4) as the ends of its one diameter is : (A) (x−2)(x−8)+(y−4)(y−16)=0 (B) (x−4)(x−8)+(y−2)(y−16)=0 (C) (x−4)(x−16)+(y−4)(y−8)=0 (D) none of these
View SolutionA line intersects x-axis at A(2,0) and y-axis at B(0,4). A variable lines PQ which is perpendicular to AB intersects x-axis at P and y-axis at Q. AQandBP intersect at R. Locus of R is : (A) x2+y2−2x+4y=0 (B) x2+y2+2x+4y=0 (C) x2+y2−2x−4y=0 (D) x2+y2+2x−4y=0
View SolutionA line intersects x-axis at A(2,0) and y-axis at B(0,4). A variable lines PQ which is perpendicular to AB intersects x-axis at P and y-axis at Q. AQandBP intersect at R. Image of the locus of R in the line y=−x is : (A) x2+y2−2x+4y=0 (B) x2+y2+2x+4y=0 (C) x2+y2−4y=0 (D) x2+y2+2x−4y=0
View Solutionx2,−4y2−2x+16y−40=0 reprsents
View SolutionThe locus of the poles of the line 2x+3y-4=0 w.r.t. the circle x2+y2+2λx−16=0 is
View SolutionA≡(−4,0),B≡(4,0).MandN are the variable points of the y-axis such that M lies below NandMN=4 . Lines AMandBN intersect at P. The locus of P is (a) 2xy−16−x2=0 (b) 2xy+16−x2=0 (c) 2xy+16+x2=0 (d) 2xy−16+x2=0
View SolutionSuppose ax+by+c=0 , where a,bandc are in AP be normal to a family of circles. The equation of the circle of the family intersecting the circle x2+y2−4x−4y−1=0 orthogonally is (a)x2+y2−2x+4y−3=0 (b)x2+y2−2x+4y+3=0 (c)x2+y2+2x+4y+3=0 (d) x2+y2+2x−4y+3=0
View SolutionA≡(−4,0),B≡(4,0).MandN are the variable points of the y-axis such that M lies below NandMN=4 . Lines AMandBN intersect at P. The locus of P is (a) 2xy−16−x2=0 (b) 2xy+16−x2=0 (c) 2xy+16+x2=0 (d) 2xy−16+x2=0
View Solution
