Find the area bounded by y=−(log)ex,y=−(log)ex,y=(log)e(−x),andy=−(log)e(−x).
Text Solution
The correct Answer is:4 sq. units
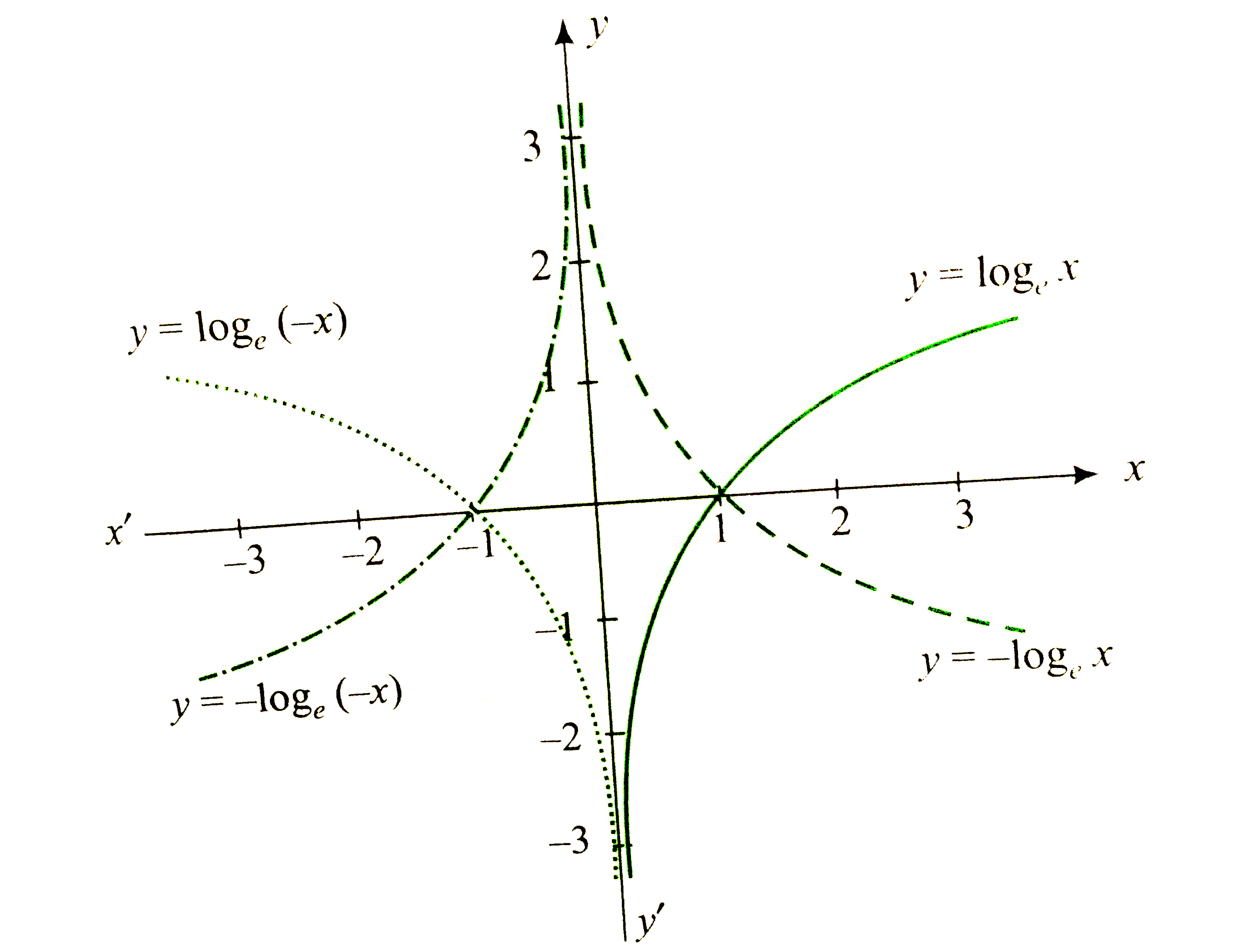
From the figure, required area = area of shaded region
=1+1+1+1=4 sq. units.
|
Topper's Solved these Questions
- AREABOOK - CENGAGECHAPTER - AREAEXERCISE - Exercise 9.3
7 Videos
- AREABOOK - CENGAGECHAPTER - AREAEXERCISE - Exercise (Single)
40 Videos
- AREABOOK - CENGAGECHAPTER - AREAEXERCISE - Exercise 9.1
9 Videos
- APPLICATIONS OF DERIVATIVESBOOK - CENGAGECHAPTER - APPLICATIONS OF DERIVATIVESEXERCISE - Subjective Type
2 Videos
- AREA UNDER CURVESBOOK - CENGAGECHAPTER - AREA UNDER CURVESEXERCISE - Question Bank
10 Videos
Similar Questions
Find the area bounded by y=logex,y=−logex,y=loge(−x),andy=−loge(−x).
View SolutionFind the area bounded by (a) y=(log)e|x|andy=0 (b) y=∣∣(log)e∣∣x∣∣andy=0
View SolutionThe area bounded by the curves y=(log)exandy=((log)ex)2 is
View SolutionFind the area bounded by a . y=(log)e|x|andy=0 b y=∣∣(log)3∣∣x∣∣andy=0
View SolutionFind the area bounded by
(i) y=loge|x|andy=0
(ii) y=|loge|x||andy=0
View SolutionFind the area bounded by
(i) y=loge|x|andy=0
(ii) y=|loge|x||andy=0
View SolutionPlot the curve y=(log)e(−x).
View SolutionPlot the curve y=(log)e(−x).
View SolutionCompute the area of the region bounded by the curves y=ex(log)exandy=logxex
View SolutionCompute the area of the region bounded by the curves y−ex(log)exandy=logxex
View Solution
