Question

If ${\displaystyle (-2,5)}$ and (3,7) are the points of intersection of the tangent and normal at a point on a parabola with the axis of the parabola, then the focal distance of that point is

Options

A. `(sqrt(29))/(2)`
B. `(5)/(2)`
C. `sqrt(29)`
D. `(2)/(5)`

Answer

A

Explanation

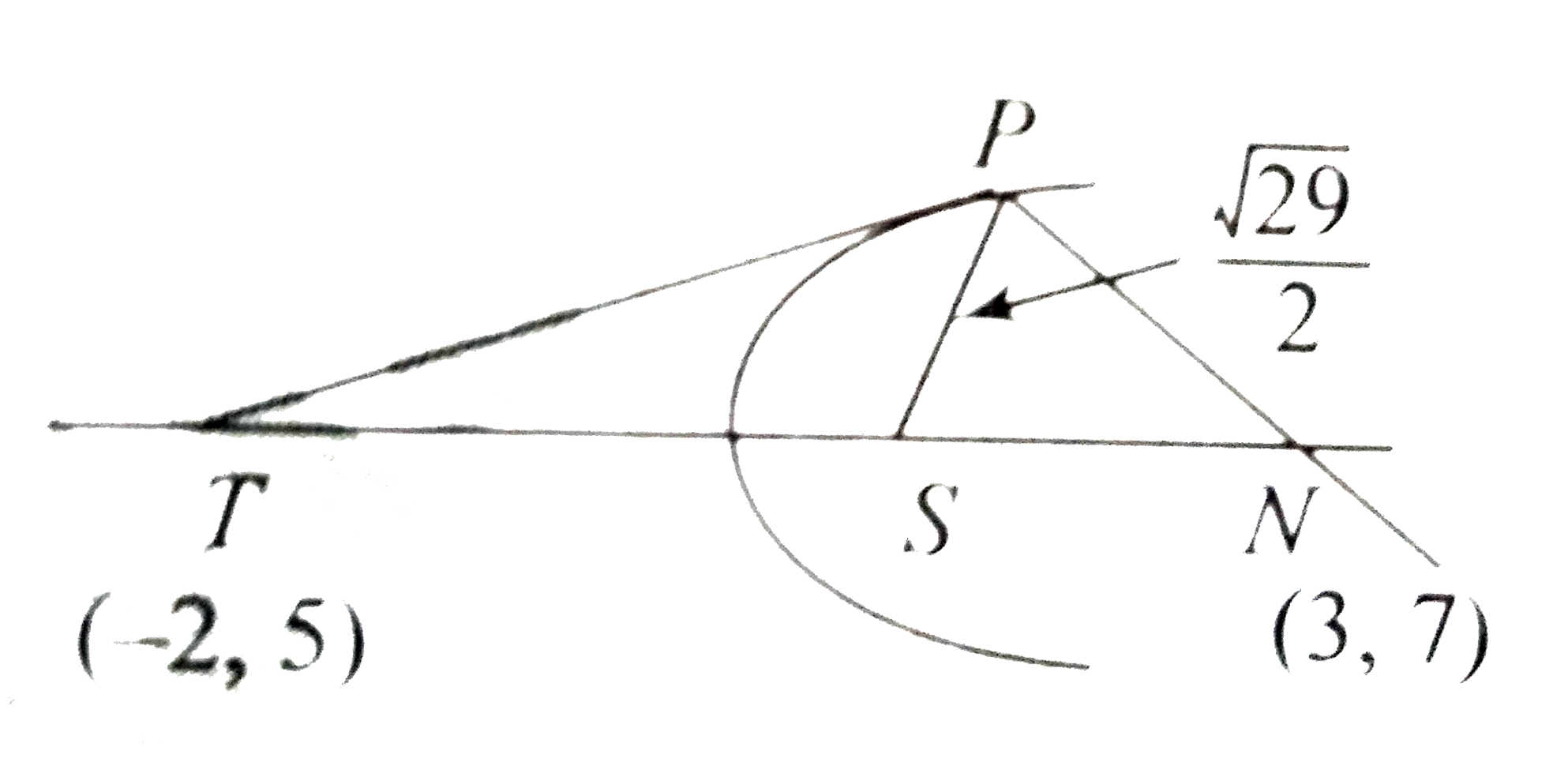
For parabola `y^(2) = 4ax`, tangent and normal at point `P(a t^(2),2a t)` meets x-axis at `T(-a t^(2),0)` and `N(2a +a t^(2),0)` Thus, focus S is the mid-point of TN.
Also, `SP = ST = SN = a + a t^(2)`
`:. SP = TN//2`
For given data, `TN = sqrt(29)`
`:. SP = (sqrt(29))/(2)`